Two long coaxial and conducting cylinders of radius a and b are separated by a material of conductivity σ and a constant potential difference V is maintained between them, by a battery. Then the current, per unit length of the cylinder flowing from one cylinder to the other is
4πσln(b/a)V
4πσ(b+a)V
2πσln(b/a)V
2πσ(b+a)V
The correct Answer is:C
To find the current per unit length flowing between two coaxial conducting cylinders separated by a material of conductivity σ, we can follow these steps:
Step 1: Understand the Setup
We have two long coaxial cylinders:
- Inner cylinder radius = a
- Outer cylinder radius = b
- Conductivity of the material between the cylinders = σ
- Potential difference maintained between the cylinders = V
Step 2: Define the Resistance
The resistance R of the cylindrical shell between the two cylinders can be calculated using the formula for resistance:
R=ρLA
Where:
- ρ is the resistivity of the material, which is given by ρ=1σ
- L is the length of the cylinder
- A is the cross-sectional area
Step 3: Calculate the Cross-Sectional Area
For a cylindrical shell at a distance x from the center, the thickness is dx and the area A is given by the circumference of the cylinder multiplied by the thickness:
A=2πx⋅L
Step 4: Calculate the Resistance of a Differential Element
The differential resistance dR of a thin cylindrical shell of thickness dx at radius x is:
dR=ρ⋅dx2πxL
Substituting ρ=1σ:
dR=1σ⋅dx2πxL
Step 5: Integrate to Find Total Resistance
To find the total resistance R from x=a to x=b, we integrate:
R=∫badR=∫ba1σ⋅dx2πxL
This simplifies to:
R=1σ⋅2πL∫badxx
The integral ∫dxx=lnx, so:
R=1σ⋅2πL(lnb−lna)=1σ⋅2πLlnba
Step 6: Calculate the Current
Using Ohm's law, the current I flowing through the resistance R when a potential difference V is applied is:
I=VR
Substituting the expression for R:
I=V⋅σ⋅2πL⋅1lnba
Step 7: Calculate Current per Unit Length
To find the current per unit length IL:
IL=V⋅σ⋅2πlnba
Final Result
Thus, the current per unit length flowing from one cylinder to the other is:
IL=V⋅σ⋅2πlnba
We have two long coaxial cylinders:
- Inner cylinder radius = a
- Outer cylinder radius = b
- Conductivity of the material between the cylinders = σ
- Potential difference maintained between the cylinders = V
The resistance R of the cylindrical shell between the two cylinders can be calculated using the formula for resistance:
R=ρLA
Where:
- ρ is the resistivity of the material, which is given by ρ=1σ
- L is the length of the cylinder
- A is the cross-sectional area
For a cylindrical shell at a distance x from the center, the thickness is dx and the area A is given by the circumference of the cylinder multiplied by the thickness:
A=2πx⋅L
The differential resistance dR of a thin cylindrical shell of thickness dx at radius x is:
dR=ρ⋅dx2πxL
Substituting ρ=1σ:
dR=1σ⋅dx2πxL
To find the total resistance R from x=a to x=b, we integrate:
R=∫badR=∫ba1σ⋅dx2πxL
This simplifies to:
R=1σ⋅2πL∫badxx
The integral ∫dxx=lnx, so:
R=1σ⋅2πL(lnb−lna)=1σ⋅2πLlnba
Using Ohm's law, the current I flowing through the resistance R when a potential difference V is applied is:
I=VR
Substituting the expression for R:
I=V⋅σ⋅2πL⋅1lnba
To find the current per unit length IL:
IL=V⋅σ⋅2πlnba
Thus, the current per unit length flowing from one cylinder to the other is:
IL=V⋅σ⋅2πlnba
Topper's Solved these Questions
Similar Questions
A long thick conducting cylinder of radius 'R' carries a current uniformly distributed over its cross section :
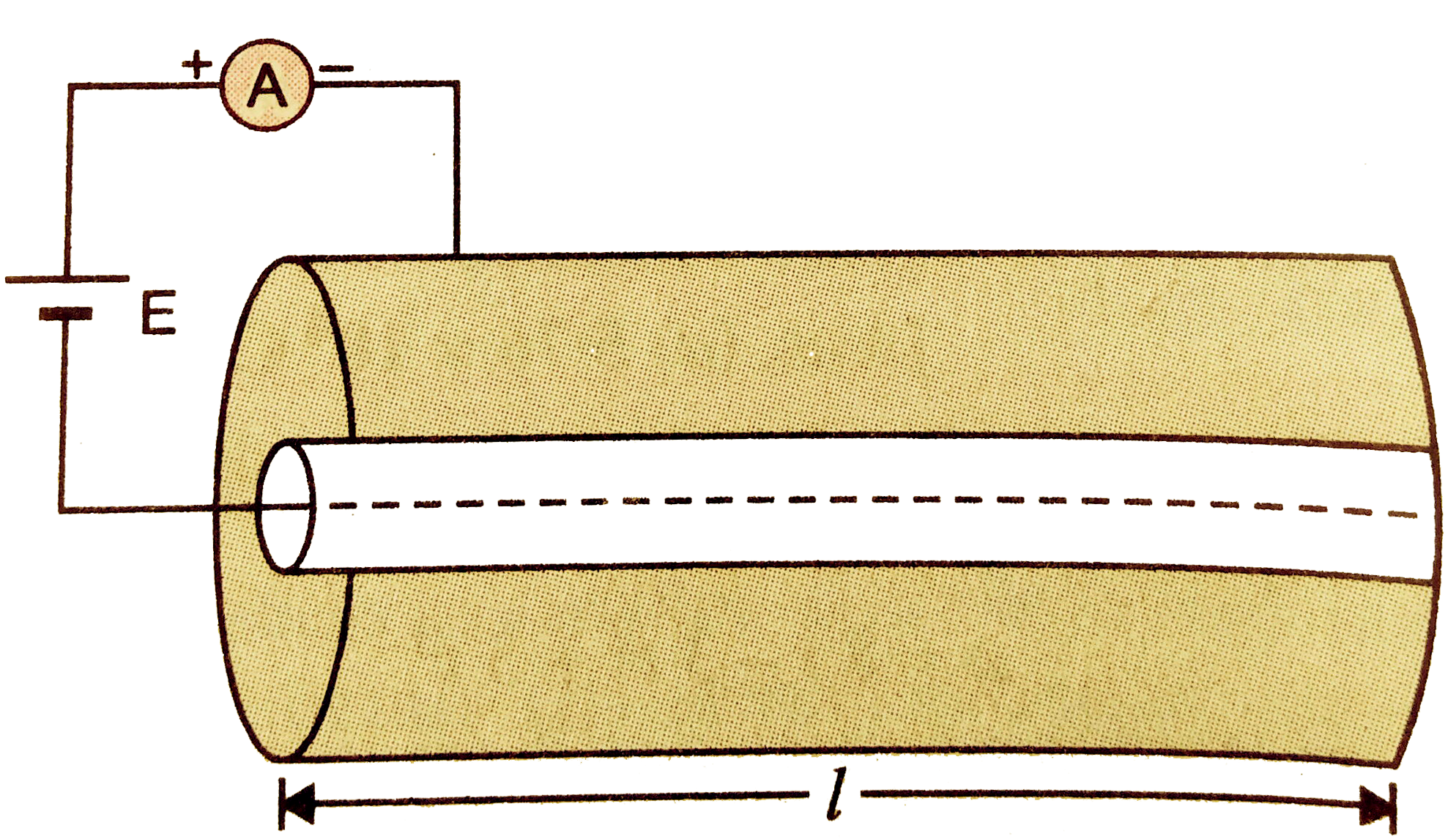
View SolutionA hollow cylinder of length l and of radii a and b is filled with a material of resistivity ρ and is connected to a battery of emf E through an ammeter. Find the current through ammeter
View Solution
Knowledge Check
Consider a thick cylinder of inner radius a and b has conductivity σ. A battery of emf V is connected between the inner and outer surface of the cyllinder. Find the current flowing, per unit length of the cylinder
A4πσln(b/a)VB4πσ(b+a)VC2πσ(b+a)VD2πσln(b/a)VA parallel -plate capacitor is connected to a battery that maintains a constant potential difference V between the plates. If the plates are pulled away from each other, increasing their separation, does the magnitude of the charge on the plates (a) increase, (b) decrease, or (c ) remain the same ?
AIncreasesBDecreasesCRemain sameDBecome zeroA cylinder of radius R made of a material of thermal conductivity K1 is surrounded by a cylinderical shell of inner radius R and outer radius 2R made of a material of thermal conductivity K2. The two ends of the combined system are maintained at two different temperatures. There is no loss of heat across the cylindrical surface and the system is in steady state. The effictive thermal conductivity of the system is
AK1+K2BK1K2/(K1+K2)C(K1+3K2)/4D(3K1+3K2)/4
Find the force per unit area on the surface of a long hollow cylinder carrying uniform current I and radius of the cylinder is R.
View SolutionA conducting wire of length 'I', area of cross-section A and electric resistivity ρ is connected between the terminals of a battery. A potential difference V is developed between its ends, causing an electric current.
If the length of the wire of the same material is doubled and the area of cross-section is halved, the resultant current would be:
View SolutionA long charged cylinder of linear charge density λ surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders?
View SolutionA long charged cylinder of linear charged density à is surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders ?
View SolutionThere is one long non-conducting solid cylinder of radius R. The volume charge density is given by ρ=kx2 where k is a constant and x is the distance of the point from centre of cylinder. Electric field intensity inside the cylinder at a distance x from the centre is
View Solution