In the triangle ABC, the base BC is trisected
at D and E. The line through D, parallel to
AB, meets AC at F and the line through E
parallel to AC meets AB at G. Let EG and
DF intersect at H. What is the ratio of the
sum of the area of parallelogram AGHF and
the area of the triangle DHE to the area of
the triangle ABC ?
A. 1/2
B. 1/3
C. 1/4
D. 1/6
To solve the problem, we will follow these steps:
Step 1: Understand the triangle and its trisection
We have triangle ABC where the base BC is trisected at points D and E. This means that if BC is divided into three equal parts, then BD = DE = EC.
Step 2: Assign lengths
Let's assign a length to BC. For simplicity, let BC = 3 units. Therefore, we have:
- BD = 1 unit
- DE = 1 unit
- EC = 1 unit
Step 3: Draw parallel lines
According to the problem:
- A line through D parallel to AB meets AC at point F.
- A line through E parallel to AC meets AB at point G.
Step 4: Identify the areas
We need to find the areas of:
1. Parallelogram AGHF
2. Triangle DHE
3. Triangle ABC
Step 5: Calculate the area of triangle ABC
The area of triangle ABC can be calculated using the formula for the area of an equilateral triangle:
AreaABC=√34×side2
Here, the side length is BC = 3 units.
AreaABC=√34×32=√34×9=9√34
Step 6: Calculate the area of triangle DHE
Since DHE is also an equilateral triangle with side length DE = 1 unit:
AreaDHE=√34×12=√34
Step 7: Calculate the area of parallelogram AGHF
The area of parallelogram AGHF can be thought of as being composed of two triangles (AGF and AHF). Since AG and DF are parallel to the sides of triangle ABC, the area of AGHF will be equal to the area of two smaller equilateral triangles with side length 1 unit:
AreaAGHF=2×√34×12=2×√34=√32
Step 8: Calculate the total area of AGHF and DHE
Now we can find the total area of parallelogram AGHF and triangle DHE:
Total Area=AreaAGHF+AreaDHE=√32+√34
To add these, we need a common denominator:
Total Area=2√34+√34=3√34
Step 9: Find the ratio
Now, we find the ratio of the total area of AGHF and DHE to the area of triangle ABC:
Ratio=Total AreaAreaABC=3√349√34=39=13
Final Answer
The ratio of the sum of the area of parallelogram AGHF and the area of triangle DHE to the area of triangle ABC is 13.
---
We have triangle ABC where the base BC is trisected at points D and E. This means that if BC is divided into three equal parts, then BD = DE = EC.
Let's assign a length to BC. For simplicity, let BC = 3 units. Therefore, we have:
- BD = 1 unit
- DE = 1 unit
- EC = 1 unit
According to the problem:
- A line through D parallel to AB meets AC at point F.
- A line through E parallel to AC meets AB at point G.
We need to find the areas of:
1. Parallelogram AGHF
2. Triangle DHE
3. Triangle ABC
The area of triangle ABC can be calculated using the formula for the area of an equilateral triangle:
AreaABC=√34×side2
Here, the side length is BC = 3 units.
AreaABC=√34×32=√34×9=9√34
Since DHE is also an equilateral triangle with side length DE = 1 unit:
AreaDHE=√34×12=√34
The area of parallelogram AGHF can be thought of as being composed of two triangles (AGF and AHF). Since AG and DF are parallel to the sides of triangle ABC, the area of AGHF will be equal to the area of two smaller equilateral triangles with side length 1 unit:
AreaAGHF=2×√34×12=2×√34=√32
Now we can find the total area of parallelogram AGHF and triangle DHE:
Total Area=AreaAGHF+AreaDHE=√32+√34
To add these, we need a common denominator:
Total Area=2√34+√34=3√34
Now, we find the ratio of the total area of AGHF and DHE to the area of triangle ABC:
Ratio=Total AreaAreaABC=3√349√34=39=13
The ratio of the sum of the area of parallelogram AGHF and the area of triangle DHE to the area of triangle ABC is 13.
Similar Questions
Draw any triangle ABC . Bisect side AB at D . Through D , draw a line parallel to BC , meeting AC in E . Measure AE and EC .
View SolutionIn △ ABC, the bisectors of ∠B and ∠ C intersect at I. A line drawn through I and parallel to BC intersects AB at P and AC at Q. Prove that PQ = BP + CQ.
View Solution
Knowledge Check
- Question 1 - Select One
If D, E and F are the mid-points of sides BC, CA and AB respectively of △ABC, then the ratio of the areas of △DEF to the area of △ABC is :
A1:4B1:2C1:3D2:3 - Question 2 - Select One
ABC is a triangle with base AB. D is a point on AB such that AB = 5 and DB = 3. What is the ratio of the area of ΔADC to the area of ΔABC ?
A3/2B2/3C3/5D2/5
P is a point on the bisector of an angle ∠ABC. If the line through P parallel to AB meets BC at Q , prove that triangle BPQ is isosceles.
View SolutionIn the figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that

ar(ΔAEDF) = ar(ΔABCDE)View SolutionABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
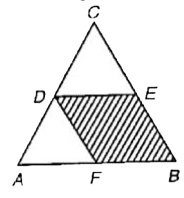 View Solution
View SolutionIn a triangle ABC, the medians AD and BE intersect at G. A line DF is drawn parallel to BE such that F is on AC. If AC = 9 cm, then what is CF equal to ?
View SolutionLet P be an interior point of a triangle ABC whose side lengths are 26, 65, 78. The line through P parallel to BC meets AB in K and AC in L. The line through P parallel to CA mmets BC in M and BA ln N. The line through P parallel to AB meets CA ln S and CB ln T. If KL, MN, ST are of equal lengths, find this common length.
View SolutionThe side AB of a parallelogram ABCD is produced to any point E. A line through A and parallel to CE meets CB produced at G and then parallelogram EBGF is completed (see the figure). Prove that
area of ||gmABCD= area of ||gmBEFG.
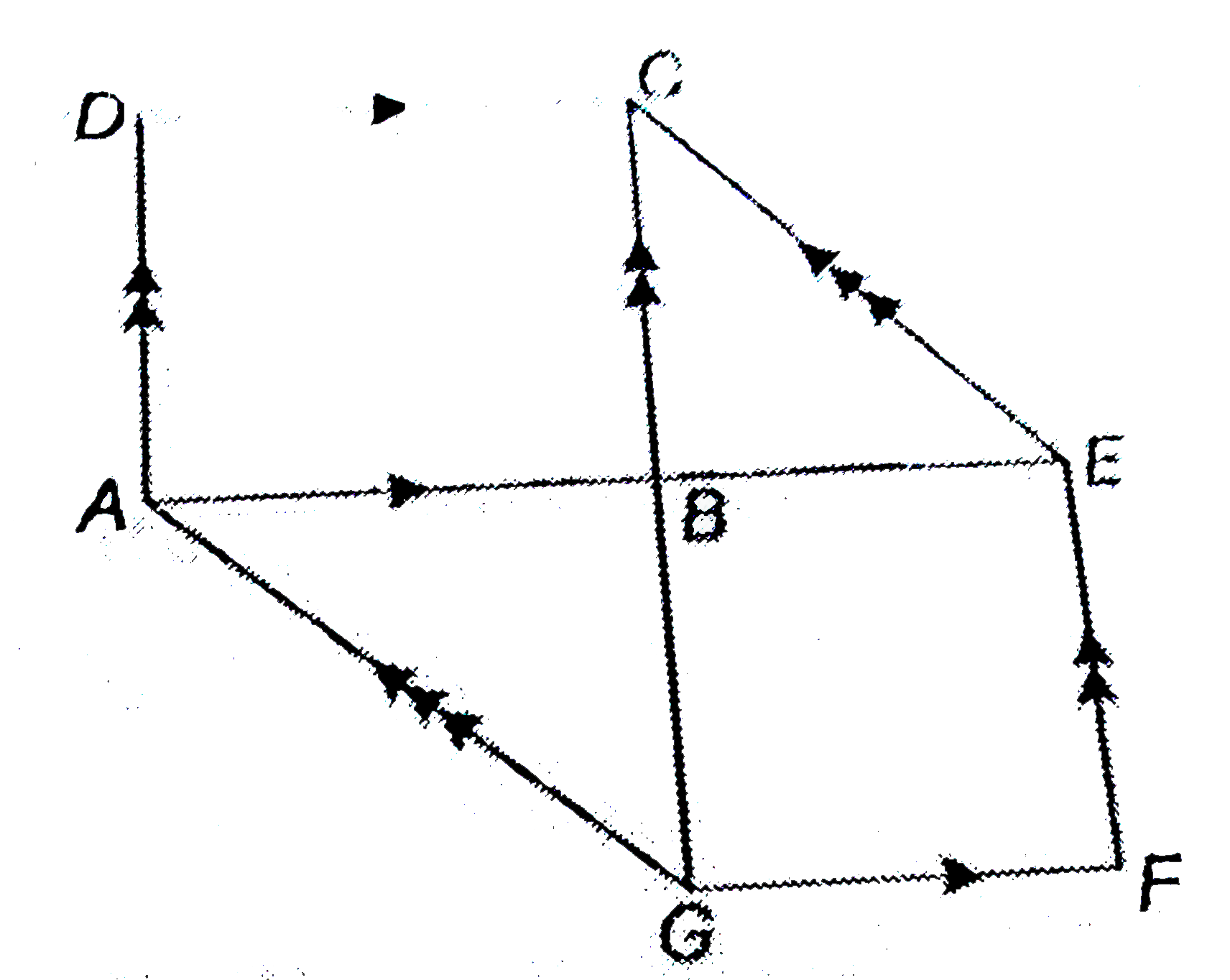 View Solution
View Solution

