Question

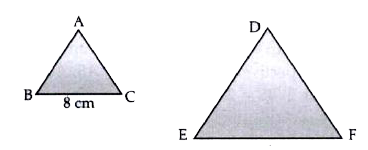
If triangles ABC and DEF are similar such that 2AB = DE and BC = 8 cm, then what is EF equal to ?

Options

A. 16 cm
B. 12 cm
C. 10 cm
D. 8 cm

Explanation

To solve the problem step by step, we will use the properties of similar triangles.

1. Understand the given information: We have two similar triangles, ABC and DEF. We know that \(2AB = DE\) and \(BC = 8 \, \text{cm}\).

2. Set up the ratio of corresponding sides: Since the triangles are similar, the ratio of their corresponding sides is equal. This can be expressed as:
\( \frac{AB}{DE} = \frac{BC}{EF} \)

3. Substitute the relationship between AB and DE: We know from the problem that \(2AB = DE\). This implies:
\( DE = 2AB \implies \frac{AB}{DE} = \frac{AB}{2AB} = \frac{1}{2} \)

4. Substitute the known value of BC: We know \(BC = 8 \, \text{cm}\). Now we can substitute this value into the ratio:
\( \frac{1}{2} = \frac{8}{EF} \)

5. Cross-multiply to solve for EF: To find \(EF\), we can cross-multiply:
\( 1 \cdot EF = 2 \cdot 8 \)
\( EF = 16 \, \text{cm} \)

6. Conclusion: Therefore, the length of \(EF\) is:
\( EF = 16 \, \text{cm} \)

Final Answer:
EF = 16 cm.

---