ΔABCandΔDEFare similar. Also ∠A=∠Dand∠B=∠E. If 4AB=DEand BC=12cm, then EF is equal to
3 cm
24 cm
16 cm
48 cm
The correct Answer is:D
To solve the problem, we need to use the properties of similar triangles. Here’s a step-by-step solution:
Step 1: Understand the similarity of triangles
Given that triangles ABC and DEF are similar, we know that the ratios of their corresponding sides are equal. This means:
ABDE=BCEF=ACDF
Step 2: Use the given information
We are given that:
1. 4AB=DE
2. BC=12cm
Step 3: Express DE in terms of AB
From the equation 4AB=DE, we can express DE as:
DE=4AB
Step 4: Set up the ratio of corresponding sides
Using the similarity of triangles, we can set up the ratio:
ABDE=BCEF
Substituting DE from step 3 into the ratio gives us:
AB4AB=12EF
Step 5: Simplify the ratio
The left side simplifies to:
14=12EF
Step 6: Cross-multiply to solve for EF
Cross-multiplying gives us:
1⋅EF=4⋅12
EF=48cm
Conclusion
Thus, the length of EF is 48cm.
---
Given that triangles ABC and DEF are similar, we know that the ratios of their corresponding sides are equal. This means:
ABDE=BCEF=ACDF
We are given that:
1. 4AB=DE
2. BC=12cm
From the equation 4AB=DE, we can express DE as:
DE=4AB
Using the similarity of triangles, we can set up the ratio:
ABDE=BCEF
Substituting DE from step 3 into the ratio gives us:
AB4AB=12EF
The left side simplifies to:
14=12EF
Cross-multiplying gives us:
1⋅EF=4⋅12
EF=48cm
Thus, the length of EF is 48cm.
Topper's Solved these Questions
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-VI)8videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-VII)6videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-IV)35videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself10videos
DISCOUNT
- BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself18videos
LCM AND HCF
Similar Questions
Knowledge Check
- Question 1 - Select One
Triangles ABC and DEF are similar. If area of ΔABC=16cm2, area of ΔDEF=25cm2 and BC=2.3cm, then EF is :
A2.875 cmB2.758 cmC2.578 cmDNone of these - Question 2 - Select One
ΔABC~ΔEDF and area (ΔABC): Area (ΔDEF)=1:4. If AB = 7cm, BC = 8 cm then DF=?
A8 cmB16 cmC18 cmD14 cm - Question 3 - Select One
In triangle ABC and DEF ,∠C=∠F,AC=DFandBC=EF . If AB = 2x - 1 and DE = 5x - 4 then the value of x is
A4B1C2D3
If triangle ABC is similar to triangle DEF such that 2AB = DE and BC = 8 cm then EF is .
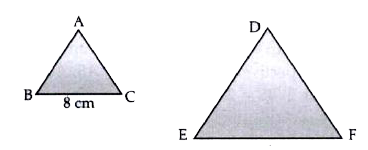 View Solution
View SolutionTwo triangles ABC and DEF such that ∠A=∠D,∠B=∠Eand∠C=∠F. Then
View Solution△ABC~△EDF and ar(△ABC):ar(△DEF)=4:9. If AB = 6 cm, BC = 8 cm and AC = 10 cm, then DF is equal to :
△ABC~△EDF और ar(△ABC):ar(△DEF)=4:9 है। यदि AB = 6 सेमी, BC = 8 सेमी तथा AC = 10 सेमी है, तो DF का मान क्या होगा ?View Solution△ABC~△EDF and ar(△ABC):ar(△DEF)=1:4. If AB =7cm, BC = 8 cm and CA = 9 cm, then DF is equal to :
△ABC~△EDF और ar(△ABC):ar(△DEF)=1:4 है। यदि AB = 7 सेमी, BC = 8 सेमी तथा CA = 9 सेमी है, तो DF का मान किसके बराबर होगा?View SolutionABC is a triangle and DE is drawn parallel to BC cutting the other sides at D and E. If AB=3.6 cm, AC=2.4 cm and AD=2.1 cm, then AE is equal to :
View Solution

