An aeroplane flies horizontally at height h with constant velocity v and drops a packet of food. The shortest distance between helicopter and person standing down when he catches the food packet?
To solve the problem, we need to analyze the motion of the food packet dropped from the airplane and the position of the airplane at the moment the packet is caught by the person on the ground.
1. Identify the Variables:
- Height of the airplane: h
- Velocity of the airplane (and the packet at the moment of release): v
- Acceleration due to gravity: g
2. Determine the Time of Flight:
The time t it takes for the packet to fall from height h can be found using the equation of motion:
h=12gt2
Rearranging gives:
t=√2hg
3. Calculate the Horizontal Distance Traveled by the Packet:
Since the packet is dropped with an initial horizontal velocity v, the horizontal distance x traveled by the packet during the time t is:
x=v⋅t
Substituting the expression for t:
x=v⋅√2hg
4. Determine the Position of the Airplane:
During the same time t, the airplane continues to move horizontally. The distance it travels in that time is also:
xplane=v⋅t=v⋅√2hg
5. Find the Shortest Distance Between the Airplane and the Person:
At the moment the packet reaches the person, the airplane will have moved horizontally the same distance x as the packet. The vertical distance between the airplane and the person on the ground remains h. Therefore, the shortest distance d between the airplane and the person when the packet is caught is simply the height h.
Final Answer:
The shortest distance between the helicopter (airplane) and the person standing down when he catches the food packet is:
h
- Height of the airplane: h
- Velocity of the airplane (and the packet at the moment of release): v
- Acceleration due to gravity: g
The time t it takes for the packet to fall from height h can be found using the equation of motion:
h=12gt2
Rearranging gives:
t=√2hg
Since the packet is dropped with an initial horizontal velocity v, the horizontal distance x traveled by the packet during the time t is:
x=v⋅t
Substituting the expression for t:
x=v⋅√2hg
During the same time t, the airplane continues to move horizontally. The distance it travels in that time is also:
xplane=v⋅t=v⋅√2hg
At the moment the packet reaches the person, the airplane will have moved horizontally the same distance x as the packet. The vertical distance between the airplane and the person on the ground remains h. Therefore, the shortest distance d between the airplane and the person when the packet is caught is simply the height h.
The shortest distance between the helicopter (airplane) and the person standing down when he catches the food packet is:
h
Topper's Solved these Questions
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A80videos
JEE MAIN 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B40videos
JEE MAIN 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions473videos
JEE MAIN
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question492videos
JEE MAIN 2022
Similar Questions
A helicopter is flying horizontally with a speed 'v' at an altitude V has to drop a food packet for a man on the ground. What is the distance of helicopter from the man when the food packet is dropped ?
View SolutionAn aeroplane is moving with a velocity u. It drops a packet from a height h. The time t taken by the packet in reaching the ground will be
View Solution
Knowledge Check
- Question 1 - Select One
An aeroplane is flying horizontally at a height of 980m with velocity 100ms−1 drops a food packet.A person on the ground is 414m ahead horizontally from the dropping point.At what velocity should he move so that he can catch the food packet.
A50√2ms−1B50√2ms−1C100ms−1D200ms−1 - Question 2 - Select One or More
An aeroplane moving horizontally from west to east with some velocity and with an acceleration 5m/s2 drops a food packet at some instant. Then:
AThe path of the packet is parabolic with respect to groundBA person sitting on the aeroplane shall see the packet is always vertically below the plane.CWith respect to plane the packet travels in a straight line making an angle tan1(1/2) east of vertical.DWith respect to plane the packet travels in a straight line making an angle tan−1(1/2) east of vertical. - Question 3 - Select One
An aeroplane is moving with horizontal velocity u at height h . The velocity of a packet dropped from it on the earth's surface will be ( g is acceleration due to gravity)
A√u2+2ghB√2ghC2ghD√u2−2gh
A boy on a train of height h1 projects a coin his friend of height h2 standing on the same train, with a velocity v relative to the train, at an angle θ with horizontal. If the train moves with a constant velocity v' in the direction of x - motion of the coin, find the
(a) distance between the boys so that the second boy can catch the coin,
(b) maximum height attained by the coin, and
( c) speed with which the second boy catches the coin relative to himself (train) and ground.View SolutionA helicopter files horizontally with constant velocity in a direction θ east of north between two points AandB, at distance d apart. Wind is blowing from south with constant speed u, the speed of helicopter relative to air is nu where n>1. find the speed of the helicopter along AB. The helicopter returns from B to A with same speed nu relative to air in same wind. Find the total time for the journeys.
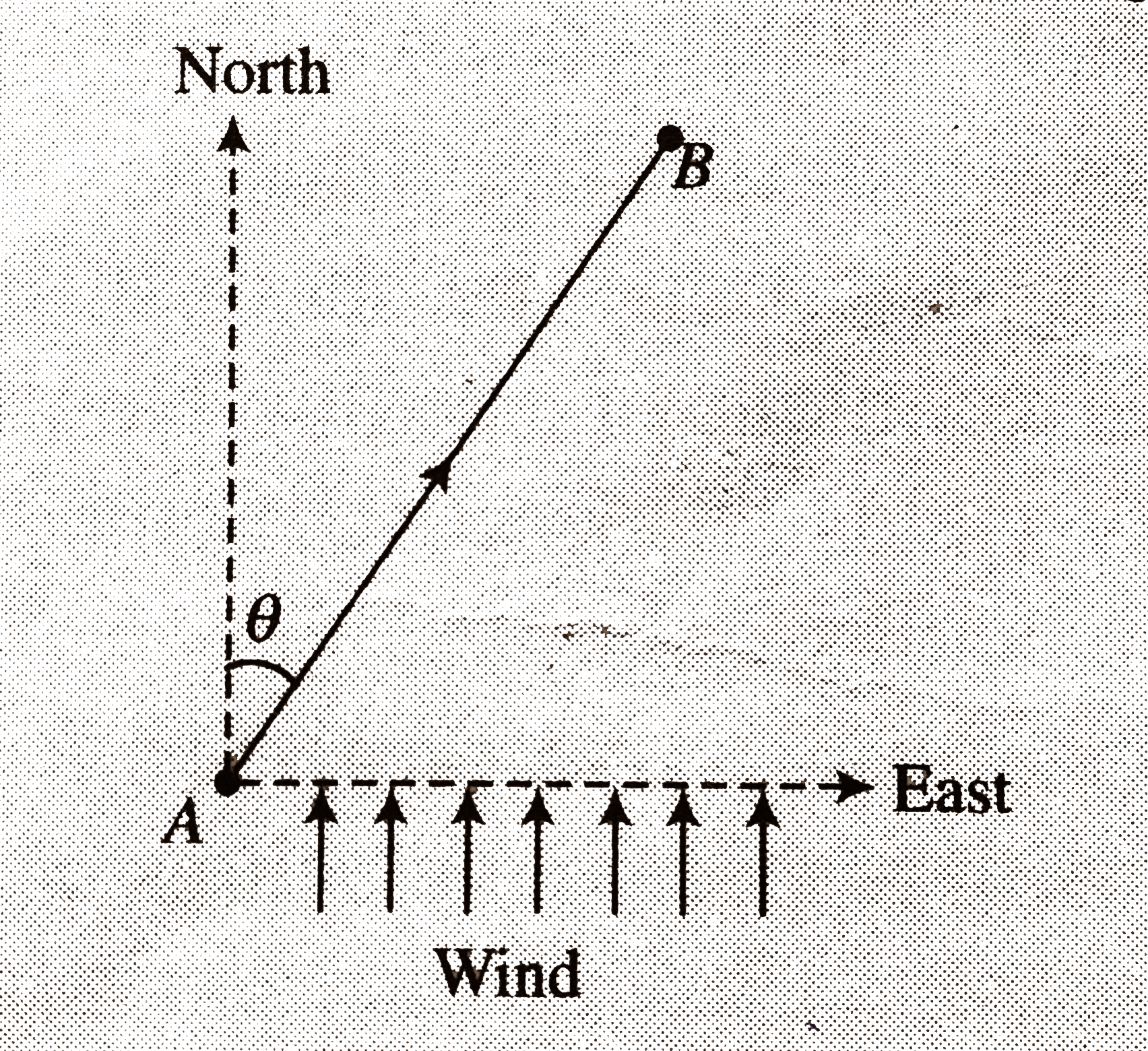 .View Solution
.View SolutionAn airplane is flying horizontally at a height of 490m with a velocity of 150ms−1. A bag containing food is to be dropped to the Jawans on the ground. How far (in meter) from them should the bag the dropped so that it directely reaches them?
View SolutionA helicopter is flying horizontally at an altitude of 2km with a speed of 100ms−1 . A packet is dropped from it. The horizontal distance between the point where the packet is dropped and the point where it hits the ground is (g=10ms−2)
View SolutionAn aeroplane is flying in a horizontal direction with a velocityu and at a height of 2000 m. When it is vertically below a point A on the ground a food packet is released from it. The packet strikes the ground at point B. If AB = 3 km and g =10 m/s2 , then the value of u is
View Solution

