यदि ⎧⎪⎨⎪⎩⎡⎢⎣514762135⎤⎥⎦⎡⎢⎣16−7624−743⎤⎥⎦⎡⎢⎣571163425⎤⎥⎦⎫⎪⎬⎪⎭=⎡⎢⎣a1a2a3b1b2b3c1c2c3⎤⎥⎦ तो 2|a2−b1|+3|a3−c1|+4|b3−c2| का मान बराबर है
0
1
2
3
The correct Answer is:A
Step by step video, text & image solution for यदि {[(5,1,4),(7,6,2),(1,3,5)][(1,6,-7),(6,2,4),(-7,4,3)][(5,7,1),(1,6,3),(4,2,5)]}=[(a_(1),a_(2),a_(3)),(b_(1),b_(2),b_(3)),(c_(1),c_(2),c_(3))] तो 2|a_(2)-b_(1)|+3|a_(3)-c_(1)|+4|b_(3)-c_(2)| का मान बराबर है by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
एक यादृच्छिक चर X का प्रायिकता बंटन निम्न रूप में दिया गया है:
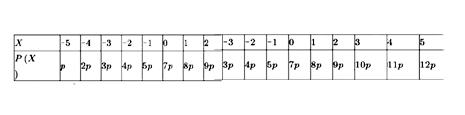
तब, p का मान है05:21View Solutionसमीकरण tan2x−sec10x+1=0 के अंतराल (0, 10) में हलों की संख्या है -
03:33View Solutionif quad /_=[[a_(1),b_(1),c_(1)a_(2),b_(2),c_(2)a_(3),b_(3),c_(3)]]
02:11View Solutionif Delta=det[[a_(1),b_(1),c_(1)a_(2),b_(2),c_(2)a_(3),b_(3),c_(3)]]
05:05View SolutionIf ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣=5, then the value of
Δ=∣∣ ∣∣b2c3−b3c2a3c2−a2c3a2b3−a3b2b3c1−b1c3a1c3−a3c1a3b1−a1b3b1c2−b2c1a2c1−a1c2a1b2−a2b1∣∣ ∣∣ is03:12View SolutionIf ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣≠0∣∣ ∣∣a1+b1xa1x+b1c1a2+b2xa2x+b2c2a3+b3xa3x+b3c3∣∣ ∣∣=0 then x=
01:54View SolutionIf ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣=Δ, then ∣∣ ∣∣A1B1C1A2B2C2A3B3C3∣∣ ∣∣=
04:28View SolutionShow that ∣∣ ∣∣a1b1−c1−a2−b2c2a3b3−c3∣∣ ∣∣=∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣
03:10View SolutionThe determinant
∣∣ ∣∣b1+c1c1+a1a1+b1b2+c2c2+a2a2+b2b3+c3c3+a3a3+b3∣∣ ∣∣02:06View SolutionIf ⎧⎪⎨⎪⎩⎡⎢⎣514762135⎤⎥⎦⎡⎢⎣16−7624−743⎤⎥⎦⎡⎢⎣571163425⎤⎥⎦⎫⎪⎬⎪⎭2020=⎡⎢⎣a1a2a3b1b2b3c1c2c3⎤⎥⎦, then the value of 2|a2−b1|+3|a3−c1|+4|b3−c2| is equal to
04:01View SolutionIf A=∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣andB=∣∣ ∣∣c1c2c3a1a2a3b1b2b3∣∣ ∣∣ then
07:25View SolutionIf ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣=5, then the value of ∣∣ ∣∣b2c3−b3c2a3c2−a2c3a2b3−a3b2b3c1−b1c3a1c3−a3c1a3b1−a1b3b1c2−b2c1a2c1−a1c2a1b2−a2b1∣∣ ∣∣ is
View SolutionIf Δ1=∣∣ ∣ ∣∣a21+b1+c1a1a2|b2|c2a1a3+b3+c3b1b2+c1b22+c2b2b3+c3c1c3c2c3c23∣∣ ∣ ∣∣ and Δ2=∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣, then find the value of Δ1Δ2.
04:56View SolutionIf ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣=5, then the value of ∣∣ ∣∣b2c3−b3c2a3c2−a2c3a2b3−a3b2b3c1−b1c3a1c3−a3c1a3b1−a1b3b1c2−b2c1a2c1−a1c2a1b2−a2b1∣∣ ∣∣ is
09:26View Solutionयदि ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣=5 है, तब का
∣∣ ∣∣b2c3−b3c2a3c2−a2c3a2b3−a3b2b3c1−b1c3a1c3−a3c1a3b1−a1b3b1c2−b2c1a2c1−a1c2a1b2−a2b1∣∣ ∣∣ मान है-03:52View SolutionThe determinant
∣∣ ∣∣b1+c1c1+a1a1+b1b2+c2c2+a2a2+b2b3+c3c3+a3a3+b3∣∣ ∣∣01:46View Solution

