Question

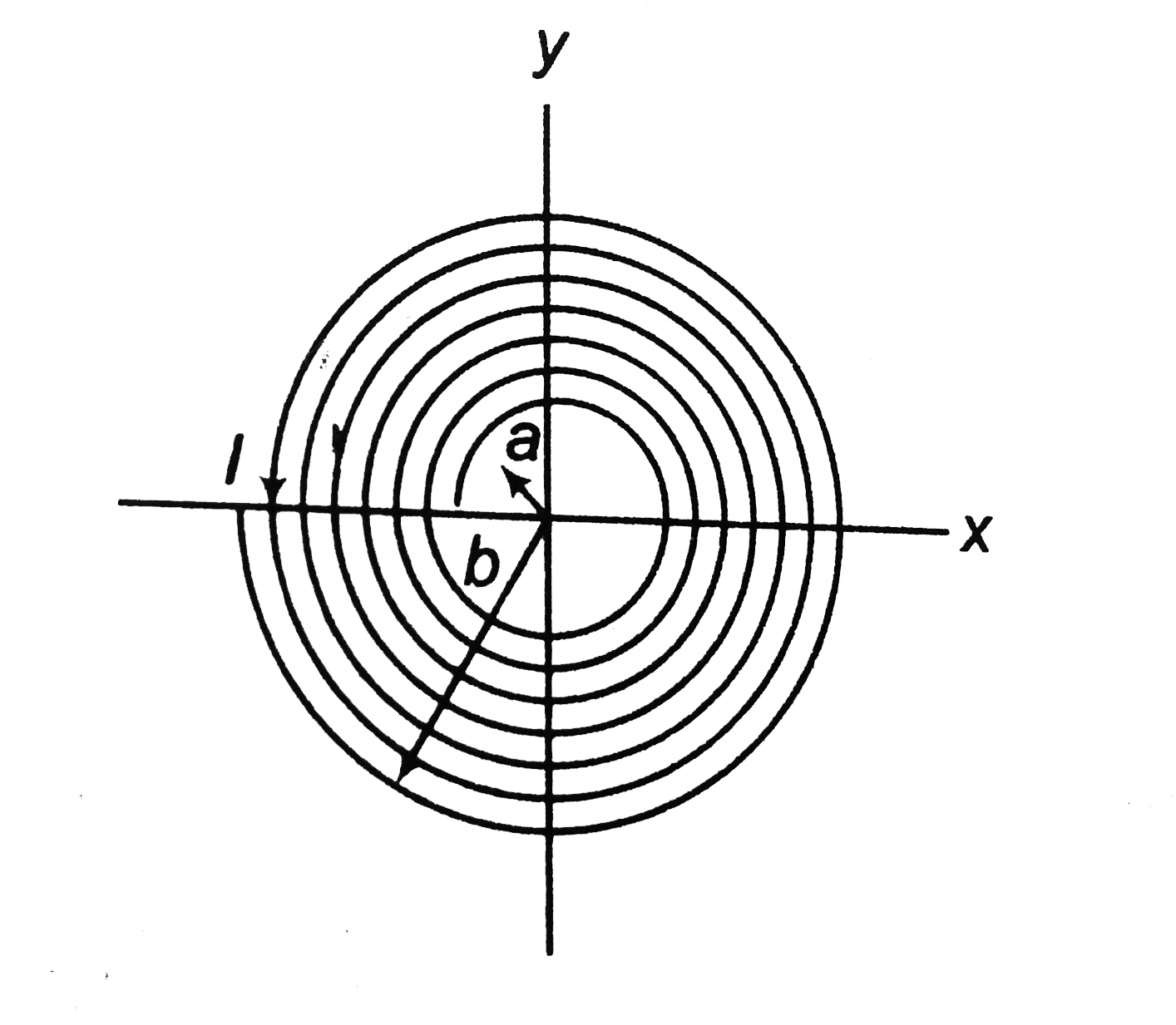
A coil having N turns is wound tightly in the form of a spiral with inner and outer radii 'a' and 'b' respectively. Find the magnetic field at centre, when a current I passes through coil:

Options

A. `(mu_(0)I)/(4(a-b))[(1)/(a)-(1)/(b)]`
B. `(mu_(0)I)/(8)[(a+b)/(a-b)]`
C. `(mu_(0)IN)/(2(b-a)) log_(e )(b)/(a)`
D. `(mu_(0)I)/(8)(a-b)/(a+b)`

Answer

C

Explanation

To find the magnetic field at the center of a tightly wound spiral coil with inner radius $$a$$, outer radius $$b$$, and $$N$$ turns carrying a current $$I$$, we can follow these steps:

1. Understanding the Coil Structure:
- The coil is wound in a spiral form with inner radius \( a \) and outer radius \( b \). The total number of turns is \( N \).

2. Determine the Width of a Small Section:
- Consider a small radial section of the coil with a width \( dR \). The radius of this section will be \( R \), where \( R \) varies from \( a \) to \( b \).

3. Calculate the Number of Turns in the Small Section:
- The total length of the coil at radius \( R \) can be approximated as \( 2\pi R \). The number of turns \( dN \) in the small section \( dR \) can be calculated as:
\( dN = \frac{N}{b - a} \cdot dR \)
- This is because the total number of turns \( N \) is uniformly distributed across the width \( b - a \).

4. Magnetic Field Contribution from the Small Section:
- The magnetic field \( dB \) at the center due to the small section can be expressed using the formula for the magnetic field due to a circular loop:
\( dB = \frac{\mu_0 I}{2R} dN \)
- Substituting \( dN \):
\( dB = \frac{\mu_0 I}{2R} \cdot \frac{N}{b - a} dR \)

5. Integrate to Find Total Magnetic Field:
- To find the total magnetic field \( B \) at the center, integrate \( dB \) from \( R = a \) to \( R = b \):
\( B = \int_a^b dB = \int_a^b \frac{\mu_0 I N}{2(b - a) R} dR \)
- This integral simplifies to:
\( B = \frac{\mu_0 I N}{2(b - a)} \int_a^b \frac{1}{R} dR \)

6. Evaluate the Integral:
- The integral \( \int \frac{1}{R} dR \) is \( \ln R \):
\( B = \frac{\mu_0 I N}{2(b - a)} \left( \ln R \right)_a^b = \frac{\mu_0 I N}{2(b - a)} (\ln b - \ln a) \)
- Using the property of logarithms, \( \ln b - \ln a = \ln \frac{b}{a} \):
\( B = \frac{\mu_0 I N}{2(b - a)} \ln \frac{b}{a} \)

Final Answer:
The magnetic field at the center of the spiral coil is given by:
\( B = \frac{\mu_0 I N}{2(b - a)} \ln \frac{b}{a} \)