Shows a cylindrical tube with adiabatic walls and fittled with a diathermic separetor. The separetor can be slid in the tube by and external mechanism. An ideal gas is injected into the two sides at equal pressures and equal temperatures. The separetor remaons in equilibrium at the middle. It is now slid to pisition where it devides the tube in the ratio of 1:3. Find the ratio of the pressures in the two parts of the vessel.
 Solution in Tamil
Solution in Tamil
According to question, tube divided in the
ration 1:3
From the formula PV= nRT
So(P2P1)=(V2V1)=1:3.

Topper's Solved these Questions
Similar Questions
Find the dimensions of
a. linear momentum
b. frequency and
c. pressure
View SolutionShould the internal energy of a system necessarily increase if heat is added to it?
View SolutionShow a cylindrical tube of volume V_(0) divided in two parts by a frictionless separator. The walls of the tube are adiabatic but the separator is conducting, Ideal gases ate filled in the two parts. When the swparator is kept in the middle, the pressure are p_(1) and p-(2) in the left part and the right part respectively. The separator is slowly slid and is released at a position where it can stay in equilibrium. Find the volumes if the two parts. (figure)
View Solutionshows a cylindrial tube of volume V with adiabatic walls containing an ideal gas. The internal energy of this ideal gas is given by 1.5nRT. The tube is divided into two equal parts by a fixed diathermic wall. Initially, the pressure and the tempreture are p1,T1 on the left and p2,T2 on the right. the system is left for sufficient time so that the tempreture becomes equal on the two sides. (a) how much work has been done by the gas on the left part ? (b) find the final pressures on the two sides. (c ) find the final equilibrium tempreture. (d) how much heat has flown from the gas on the right to the gas on the left ?
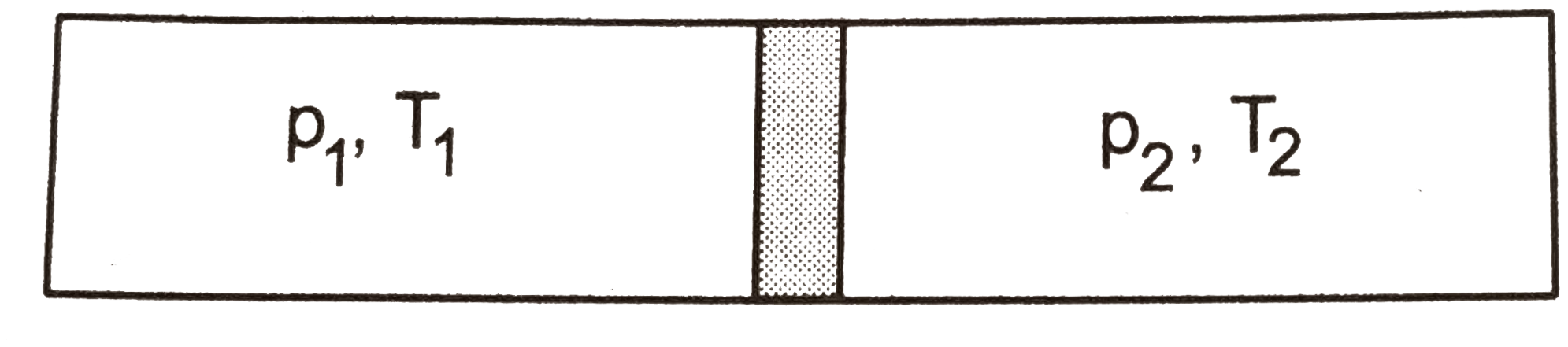
View SolutionFigure shows a cylindridcal tube with a adibatic walls and fitted with an adiabatic separtor. The separator can be slid into the tube by an external mechanism. An ideal gas(γ=1.5) is injected in the two sides at equal pressures and temperatures . The separator remains in equilibrium at the middel. It is now slid to a position where it divides the tube in the ratio 1:3 Find the ratio of the tempertures in the two parts of the vessel.
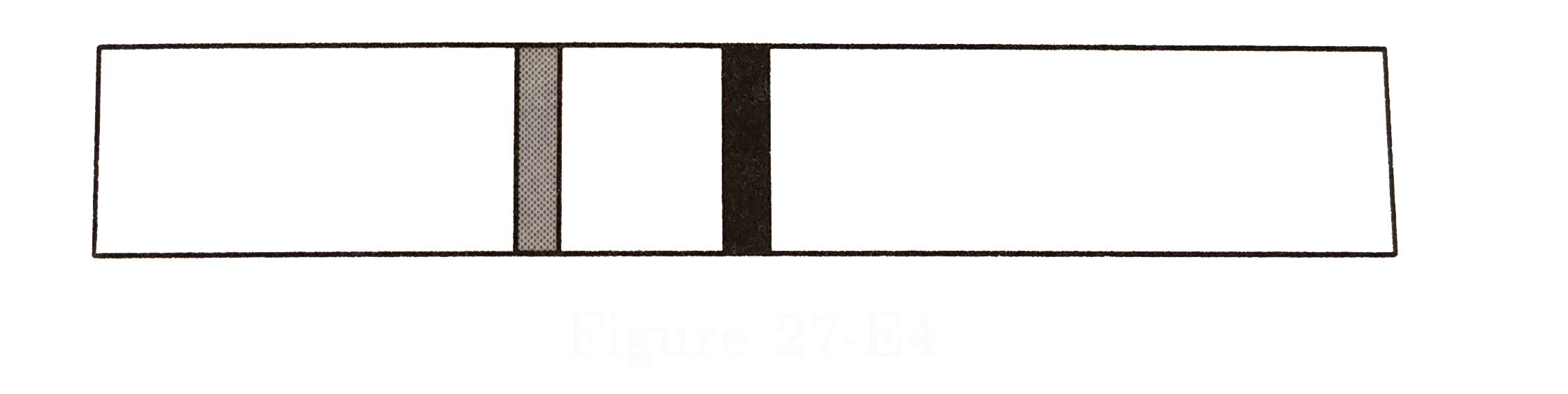
View SolutionFigure shows an adiabatic cylindrical tube of volume (V_0) divided in two parts by a frictionless adibatic separator . Initially, the separator is kept in the middle, an ideal gas at pressure (p_1) and the temperatures (T_1) is injected into the left part and the another ideal gas at pressures (P_2) and temperature (T_2) is injected into the right part. (C_p / C_v = gamma) is the same for both the gases. The separator is slid slowly and is released at a position where it can stay in equilibrium. Find (a) the volumes of the parts, (b) the heat given to the gas in the left part and (c) the final common pressure of the gases.
View SolutionWhat internal pressure (in the absence of an external presure) can be sustained (a) by a glass tube, (b) by a glass spherical flask, if in both cases the wall thickness is equal to Deltar=1.0mm and the radius of the tube and the flask equals r=25mm ?
View SolutionAn adiabatic cylindrical tube is fitted with an adiabatic separator as shown in figure. Initial separator is in equilibrium and divides a tube in two equal parts. The seperator can be slide into the tube by an extermal mechanism. An ideal gas (gamma = 1.5) is injected in the two slides at equal pressure and temperature. Now separator is slid to a piston where it divides the tube in the ratio 7 :3 The ratio of the temperature in the two parts of the vessel is sqrt(n) : sqrt(7) find n .
View SolutionIn given figure, an adiabatic cylindrical tube of volume 2V0 is divided in two equal parts by a frictionless adiabatic separator. An ideal gas in left side of a tube having pressure P1 and temperature T1 where as in the right side having pressure P2 and temperature T2.Cp/Cv=γ is the same for both the gases. The separator is slid slowly and is released at a position where it can stay in equilibrium. Find (a) the final volumes of the two parts (b) the heat given to the gas in the left part and (c) the final common pressure of the gases,
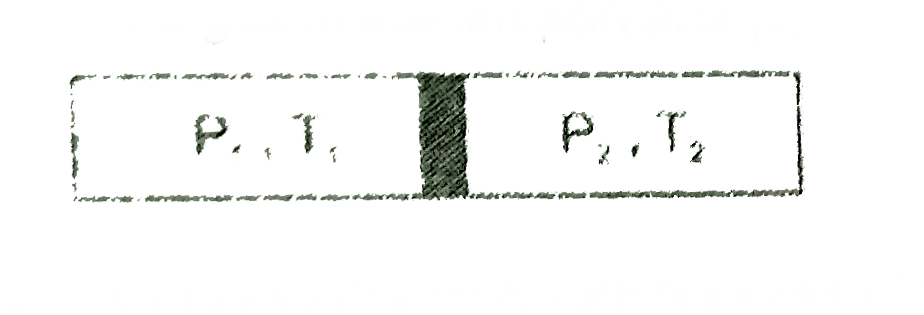
View SolutionShow that the adiabatic bulk modulus of an ideal gas is equal to the product of the pressure of the gas and the ratio of its two specific heat (gamma) .
View SolutionIs equal to the frequency of the origin of a closed and an open tube. Is the ratio of the lengths of the two tubes
View SolutionFig shows a cylindrical tube of volume V with adiabatic walls containing an ideal gas. The internal energy of this ideal gas is given by 1.5nRT. The tube is divided into two equal parts by a fixed diathermic wall. Initially, the pressure and the temperature are p1,T1 on the left and p2,T2 on the right. the system is left for sufficient time so that the temperature becomes equal on the two sides. (a) how much work has been done by the gas on the left part ? (b) find the final pressures on the two sides. (c ) find the final equilibrium temperature. (d) how much heat has flown from the gas on the right to the gas on the left ?

View SolutionFigure shows a cylindridcal tube with a adibatic walls and fitted with an adiabatic separtor. The separator can be slid into the tube by an external mechanism. An ideal gas(γ=1.5) is injected in the two sides at equal pressures and temperatures . The separator remains in equilibrium at the middel. It is now slid to a position where it divides the tube in the ratio 1:3 Find the ratio of the tempertures in the two parts of the vessel.

View SolutionTwo capillary tubes of lengths in .the ratio 2: 1 and radii in the ratio 1 :2 are connected in series. Assume the flow of the liquid through the tube is steady. Then, the ratio of pressure difference across the tubes is
View SolutionTwo capillary tubes of lengths in .the ratio 2: 1 and radii in the ratio 1 :2 are connected in series. Assume the flow of the liquid through the tube is steady. Then, the ratio of pressure difference across the tubes is
View SolutionFigure shows an adiabatic cylindrical tube of volume (V_0) divided in two parts by a frictionless adiabatic separator . Initially, the separator is kept in the middle, an ideal gas at pressure (P_1) and the temperatures (T_1) is injected into the left part and the another ideal gas at pressures (P_2) and temperature (T_2) is injected into the right part. (C_p / C_v = gamma) is the same for both the gases. The separator is slid slowly and is released at a position where it can stay in equilibrium. Find (a) the volumes of the parts, (b) the heat given to the gas in the left part and (c) the final common pressure of the gases.
View Solution
