Question

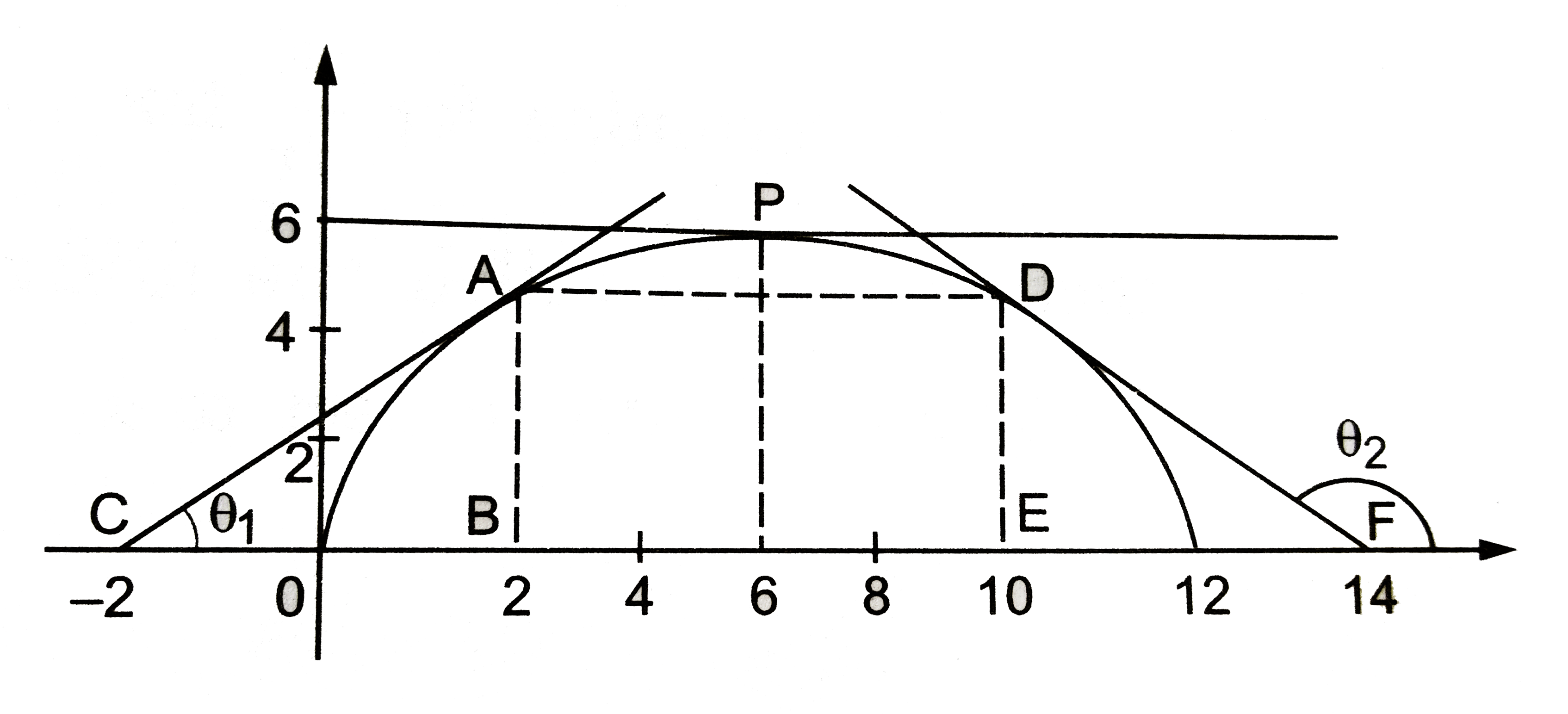
From the curve given in figure find $\frac{dy}{dx}$ at x=2, 6 and 10.

Explanation

The tangent to the curve at x=2 is AC. Its slope is `tantheta_1=(AB)/(BC)=5/4`
Thus, ` dy/dx= 5/4 at x=2`.
The tangent to the curve at x=6 is parallel to the X-axis.
Thus, `dy/dx=tantheta=0 at x=t`
The tangent to the curve at `x=10` is DF. Its slope is
`tantheta_2=DE/EF=-5/4` ltbr. Thus, `dy/dx=-5/4 at x=10`
If we are given the graph of y versus x, we can find `dy/dx` at any pintof the curve bydrawing the tangent at that point and finding its slope. Evwen if the graph is not drawn and the algebraic relation bettween y and x is given in the formof an equation, we can find `dy/dx` algebraically. Let us take an example.
The area A of a square of length L is `A=L^2`. ltbr. If we change L to `L+/_\L`, the area will change from A to `A+/_\A ure.


`A+/_\A=(L+/_\L)^2`
` = L^2+2L /_\L+(/_\L)^2`
or, `/_\A=2L(/_\L)+/_\L)^2`
or, `(/_\A)/ (/_\L)=2L+/_\L`
Now if `/_\L` is made smaller and smaller, `2L+/_\L` will appraoch 2L.
Thus, `(dA)/dL) = lim_(/_\Lrarr0)=(/_\A)/(/_\L)=2L`.