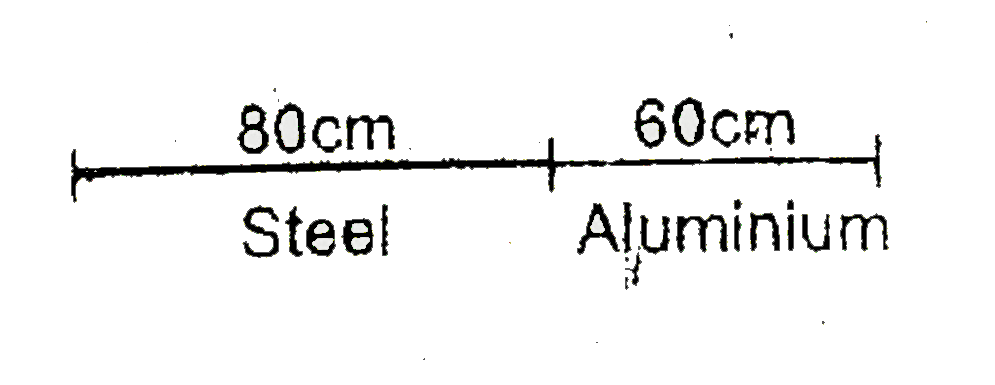
Figure shows an aluminium wire of length $60 c m$ joined to a steel wire of length $80 c m$ and stretched between two fixed supports. The tension produced is $40 N$ . The cross-sectional area of the steel wire is $1.0 m m^{2}$ and that of the aluminimum wire is $3.0 m m^{2}$ The minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node is $10 P$ (in $H z$ ) the find $P$ . Given density of aluminimum is $2.6 g / c m^{3}$ and that of steel is $7.8 g / c m^{3}$ .
Solution in Tamil

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

$P_{s} = 7.8 \frac{g m}{c} m^{3}$
$P_{A} = 2.6 \frac{g m}{c} m^{3}$
$m_{s} = P_{s} A_{s}$
$= 7.8 \times 10^{- 2} \frac{g m}{c} m$
(m=mass per unit length)
$7.8 \times 10^{- 3} k \frac{g}{m}$
$m_{A} = P_{A}$
$= 21.6 \times 10^{- 2} \times 3 \frac{g m}{c} m$
$= 7.8 \times 10^{- 2} \frac{g m}{c} m$
$= 7.8 \times 10^{- 3} \frac{k g}{m}$
A node is always placed in the joint. Since aluminium and steel rod has same mass per unit lenght, velocity of wave in both of them is same
$\to v = \sqrt{(\frac{T}{m})}$
$= \sqrt{{\frac{40}{(7.8 \times 10^{- 3})}}}$
$= \sqrt{\frac{((4 \times 10^{4}))}{7.8}}$
$= 71.6 \frac{m}{s}$
For minimum frequency there would be maximum wavelength. for maximu wavelength minimum no. of loops are to be produced.
$∴$ Maximum distance of a loop =20cm
$\to$ wavelength = $λ = 2 \times 20$
$= 40 c m = 0.4 m$ =180Hz

Updated on:21/07/2023

|

Class 11 PHYSICS TRAVELLING WAVES

Topper's Solved these Questions

Similar Questions

HC VERMA-WAVE MOTION AND WAVES ON A STRING-Exercises

Home
Ask Doubt
Profile