A body is to be slided without friction along an inclined plane of height 'h' so that it loops the loop of radius at the bottom. The value of height is :
h=2r
h=4r
h=5r
h=52.r
The correct Answer is:D
If the body starts from B, then the velocity at the point Av=√2gh. V2gh. If it is to loop round the circular track of radius r, the least velocity of projection at A is = √5gr. Hence, for looping the loop √5gr=√2gh or h=52r.
Topper's Solved these Questions
- UNIFORM CIRCULAR MOTIONBOOK - MODERN PUBLICATIONCHAPTER - UNIFORM CIRCULAR MOTIONEXERCISE - Multiple Choice Questions(LEVEL II )
1 Videos
- UNIFORM CIRCULAR MOTIONBOOK - MODERN PUBLICATIONCHAPTER - UNIFORM CIRCULAR MOTIONEXERCISE - Multiple Choice Questions
56 Videos
- TRANSFERENCE OF HEAT BOOK - MODERN PUBLICATIONCHAPTER - TRANSFERENCE OF HEAT EXERCISE - Recent Competitive Questions
4 Videos
- UNIT TEST -06BOOK - MODERN PUBLICATIONCHAPTER - UNIT TEST -06EXERCISE - Multiple Choice Questions
19 Videos
Similar Questions
A body slides without friction from a height H=60cm and then loops the loop of radius R=20cm at the bottom of an incline. Find the ratio of forces exerted on the body by the track at the positions A,B and C(g=10ms−2).
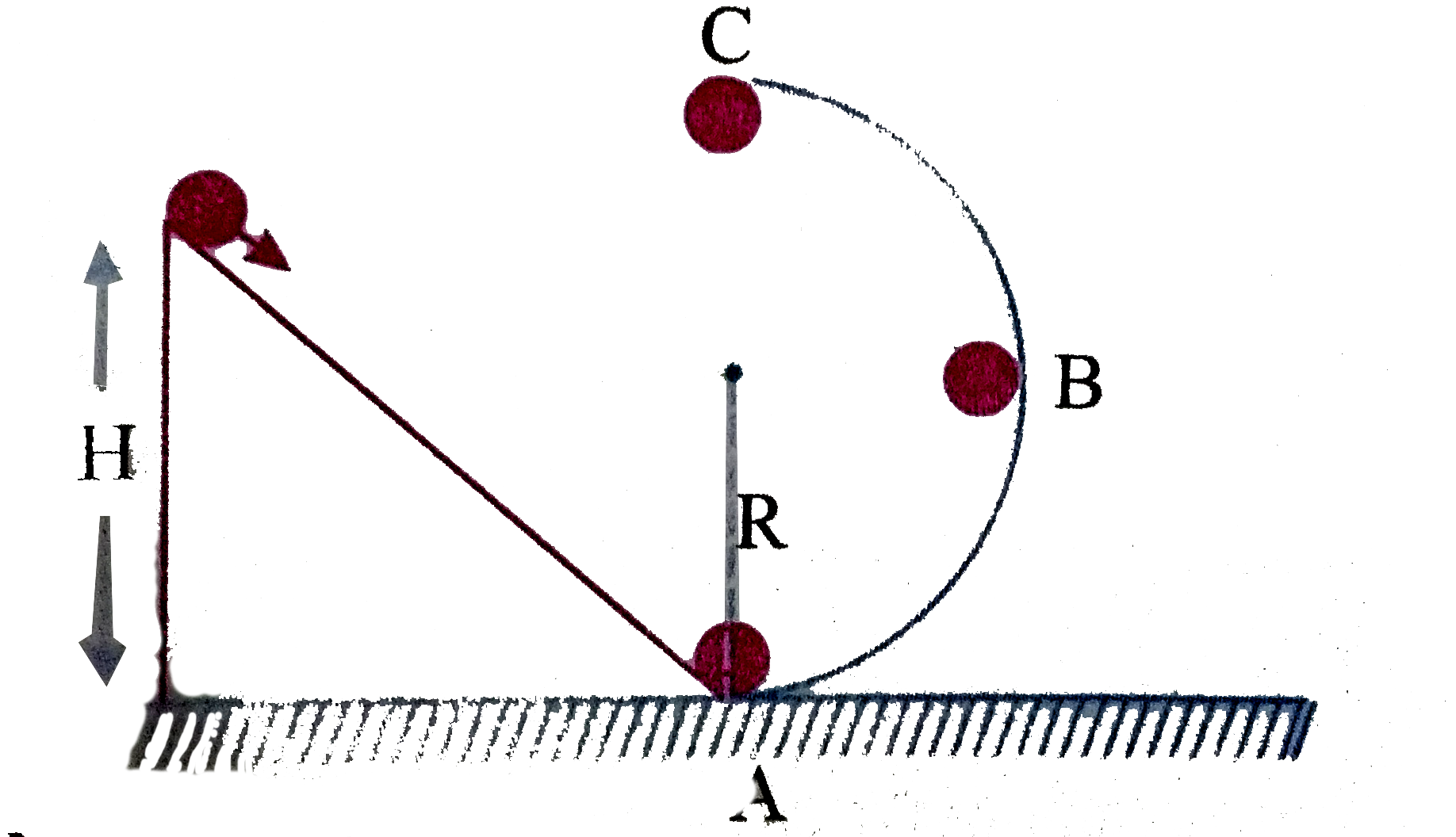 .
.
View SolutionWhen a body rolls without sliding up an inclined plane the frictional force is :
View Solution
Knowledge Check
A body slides without friction from a height H=60cm and then loops the loop of radius R=20cm at the bottom of an incline. Find the ratio of forces exerted on the body by the track at the positions A,B and C(g=10ms−2).
 .A7:3:1B5:3:1C7:4:1D1:4:7
.A7:3:1B5:3:1C7:4:1D1:4:7When a body rolls without sliding up an inclined plane the frictional force is :
Adirected up the planeBdirected down the planeCzeroDdependent on its velocityA body is slipping from an inclined plane of height h and length l . If the angle of inclination is θ , the time taken by the body to come from the top to the bottom of this inclined plane is
A√2hgB√2lgC1sinθ√2hgDsinθ√2hg
A body is slipping from an inclined plane of height h and length l . If the angle of inclination is θ , the time taken by the body to come from the top to the bottom of this inclined plane is
View SolutionA solid cylinder of radius r rolls down an inclined plane of height h and inclination θ. Calculate its speed at the bottom of the plane using energy method. Also calculate the time taken to reach of the bottom.
View SolutionA solid cylinder of mass M and radius R rolls down an inclined plane of height h without slipping. The speed of its centre when it reaches the bottom is.
View SolutionA solid cylinder of mass M and radius R rolls down an inclined plane of height h without slipping. The speed of its centre when it reaches the bottom is.
View SolutionA metal disc of radius R and mass M freely rolls down from the top of an inclined plane of height h without slipping. The speed of its centre of mass on reaching the bottom of the inclined plane is
View Solution
