A closed triangular box is kept in an electric field of magnitude E=2×103NC−1 as shown in the figure.
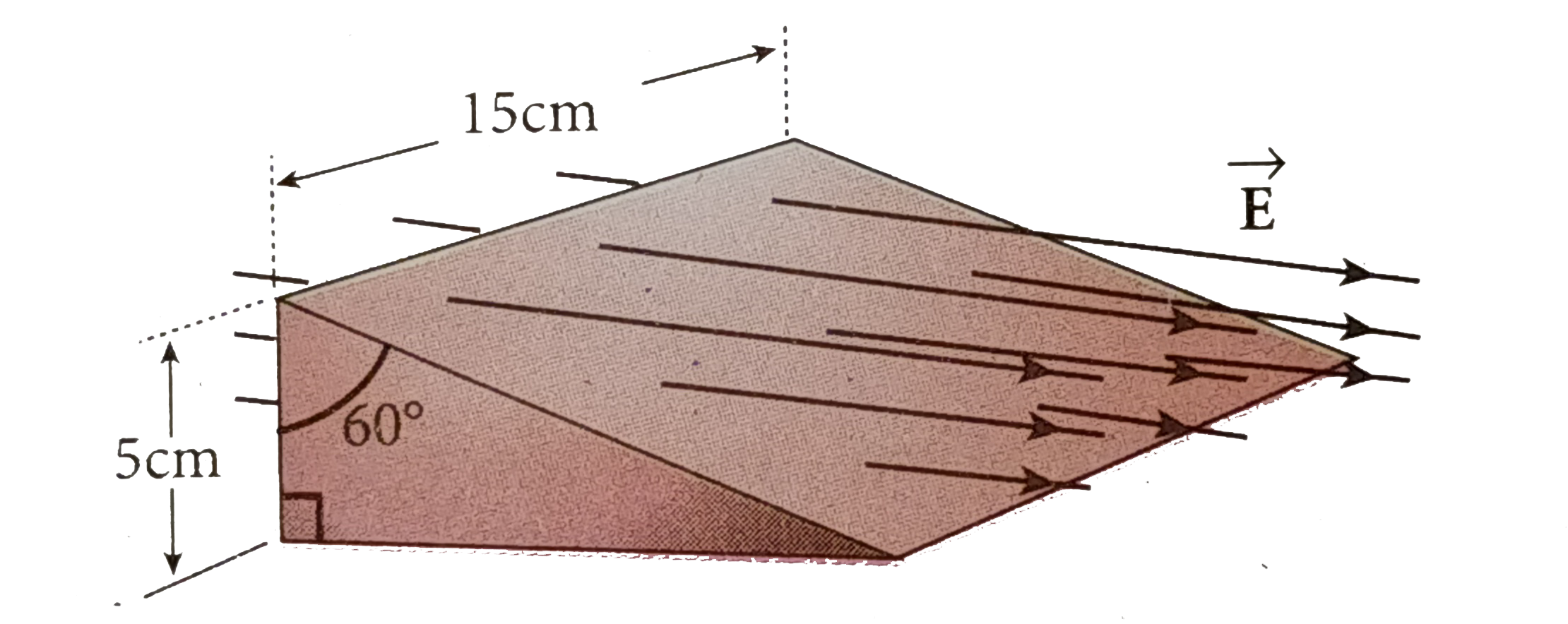
Calculate the electric flux through the vertical rectangular surface
The correct Answer is:The magnitude of electric field E=2×103NC−1 Area of the surface A=0.15×0.05
[From the diagram 1=15 cm =0.15 m, b=5 cm =0.05m]
The electric flux through
To find:
Vertical rectangular surface ϕvert=?
According to Gauss law ϕ=EAcosθ
ϕvertical surface=2×103×0.15×0.05×cosθ∘
=0.015×103
=15Nm2C−1
Similar Questions
Knowledge Check
A circular plane sheet of radius 10 cm is placed in a uniform electric field of 5×105NC−1, making an angle of 60∘ with the field. Calculate electric flux through the sheet.
A1.36×102Nm2C−1B1.36×104Nm2C−1C0.515×102Nm2C−1D0.515×104Nm2C−1An electron falls through a small distance in a uniform electric field of magnitude 2×104NC−1. The direction of the field reversed keeping the magnitude unchanged and a proton falls through the same distance. The time of fall will be
ASame in both casesBMore in the case of electronCMore in the case of protonDIndependent of charge
If the flux of the electric field through a closed surface is zero,
View SolutionAn electron falls through a small distance in a uniform electric field of magnitude 2×104NC−1. The direction of the field reversed keeping the magnitude unchanged and a proton falls through the same distance. The time of fall will be
View SolutionAn electron falls through a small distance in a uniform electric field of magnitude 2×104NC−1. The direction of the field reversed keeping the magnitude unchanged and a proton falls through the same distance. The time of fall will be
View SolutionA hemispherical surface of radius R is kept in a uniform electric field E as shown in figure. The flux through the curved surface is
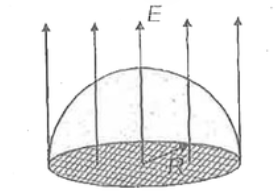
View SolutionAn electric dipole with dipole moment 4×10−9 C-m is aligned at 30∘ with the direction of a uniform electric field of magnitude 5×104NC−1.Calculate the magnitude of the torque acting on the dipole.
View SolutionIf electric flux passing through a close surface is zero then :-
View Solution
