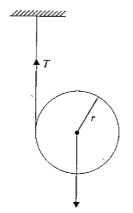
A solid cylinder of mass 50 kg and radius 0.5 m is free to rotate about the horizontal axis, A massless string is wound round the cylinder with one end attached to it and other hanging freely. What is the tension in the string required to produce an angular acceleration of 2 revolutions $s^{- 2}$ ?

Text Solution

Verified by Experts

Here, mass of the cylinder, M=50 kg
Radius of the cylinder, R=0.5 m
Angular acceleration, $α = 2 r e v s^{- 2} = 2 \times 2 π r a d s^{- 2} = 4 π r a d s^{- 2}$
Torque, $τ = T R$

Moment of inertia of the solid cylinder about its axis, $I = \frac{1}{2} M R^{2}$
$∴$ Angular acceleration of the cylinder $α = \frac{τ}{I} = \frac{T R}{\frac{1}{2} M R^{2}}$
$T = \frac{M R α}{2} = \frac{50 \times 0.5 \times 4 π}{2} = 157 N$

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Similar Questions

Knowledge Check

Question 1 - Select One
A solid cylinder of mass $50 k g$ and radius $0.5 m$ is free to rotate about the horizontal axis. A massless string is wound round the cylinder with one end attached to it and other end hanging freely. Tension in the string required to produce an angular acceleration of $2$ revolution $s^{- 2}$ is
A $25 N$
B $50 N$
C $78.5 N$
D $157 N$
Question 2 - Select One
A solid cylinder of mass 50 kg and radius 0.5 m is free to rotate about the horizontal axis . A massless string is wound around the cylinder with one end attached to it and other hanging freely . Tension in the string required to produce an angular acceleration of 2 revolutions $s^{- 2}$ is
A25 N
B50 N
C78.5 N
D157 N
Question 3 - Select One
A cylinder of mass $50 m g$ and radius $0.5 m$ is free to rotate about the horizontal axis. A massless string is wound round the cylinder with one end attached to it and other hanging freely. Tension in the string required to produce an angular acceleration of $2$ revolutions $s^{- 2}$ is
A25 N
B50 N
C78.5 N
D157 N

Recommended Questions

Home
Ask Doubt
Profile