If 'Driver' = 12
' Pedestrian' = 20.
'Accident = 16
Then 'Car' = ?
3
6
9
18
To solve the problem, we need to identify the pattern used to assign numerical values to the words 'Driver', 'Pedestrian', and 'Accident'.
1. Identify the Number of Letters in Each Word:
- For 'Driver': The number of letters is 6.
- For 'Pedestrian': The number of letters is 11.
- For 'Accident': The number of letters is 8.
- For 'Car': The number of letters is 3.
2. Determine the Relationship Between the Number of Letters and the Given Values:
- For 'Driver': 6 letters → 12 (which is 6 × 2).
- For 'Pedestrian': 11 letters → 20 (which is 11 × 2).
- For 'Accident': 8 letters → 16 (which is 8 × 2).
3. Apply the Same Pattern to 'Car':
- The word 'Car' has 3 letters.
- Following the established pattern, we multiply the number of letters by 2:
- 3 letters × 2 = 6.
4. Conclusion:
- Therefore, the value assigned to 'Car' is 6.
Final Answer:
Car = 6.
---
- For 'Driver': The number of letters is 6.
- For 'Pedestrian': The number of letters is 11.
- For 'Accident': The number of letters is 8.
- For 'Car': The number of letters is 3.
- For 'Driver': 6 letters → 12 (which is 6 × 2).
- For 'Pedestrian': 11 letters → 20 (which is 11 × 2).
- For 'Accident': 8 letters → 16 (which is 8 × 2).
- The word 'Car' has 3 letters.
- Following the established pattern, we multiply the number of letters by 2:
- 3 letters × 2 = 6.
- Therefore, the value assigned to 'Car' is 6.
Car = 6.
Similar Questions
The driver tried his best (a)/ to avert the accident by bringing the car (b)/ to a suddenly stop.(c)/ No error (d)
View SolutionA car is moving with speed u. Driver of the car sees red traffic light. His reaction time is t, then find out the distance travelled by the car after the instant when the driver decided to apply brakes. Assume uniform retardation 'a' after applying brakes.
View Solution
Knowledge Check
- Question 1 - Select One
If Driver = 12, PEDESTRAIN = 20, ACCIDENT = 16, Then CAR = ?
A3B6C8D10 - Question 2 - Select One
If d: driver is drunk, a: driver meets with an accident, translate the statement 'If the Driver is not drunk, then he cannot meet with an accident' into symbols
A~a→~dB~d→~aC~d∧aDa∧~d - Question 3 - Select One
A car driver, driving in a fog, passes a pedestrian who was walking at the rate of 2 km/hr in the same direction. The pedestrian could see the car for 6 minutes and it was visible to him upto a distance of 0.6 km. What was the speed of the car?
A15 km/hrB30 km/hrC20 km/hrD8 km/hr
Describe how Sonal’s car met with an accident?
View SolutionThe rear view mirror of a car is a plane mirror. The driver can see the image of a bus parked behind his car in the rear view mirror. If the driver reverses his car at a speed of 0.75 m/s, at which speed the image of bus will appear to approach the car driver ?
View SolutionOn a Saturday night, 20% of all drivers in U.S.A. are under the influence of alcohol. The probability that a driver under the influence of alcohol will have an accident is 0.001. The probability that a sober driver will have an accident is 0.0001. If a car on a Saturday night smashed into a tree, the probability that the driver was under the influence of alcohol, is p then 7P is equals to
View SolutionAn insurance company selected 2000 drivers at random (i.e., without any preference of one driver over another) in a particular city to find a relationship between age and accidents . The data obtained are given in following table :
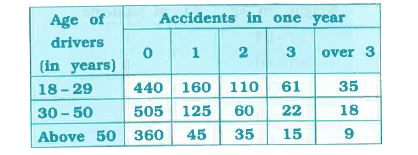
Find the probabilities of the following events for a driver chosen at random from the city.
having no accidents in one yearView SolutionA car driver, driving in a fog, passes a pedestrian who was walking at the rate of 2 km/hr in the same direction. The pedestrian could see the car for 6 minutes and it was visible to him up to a distance of 0.6 km. What was the speed of the car?
View Solution

