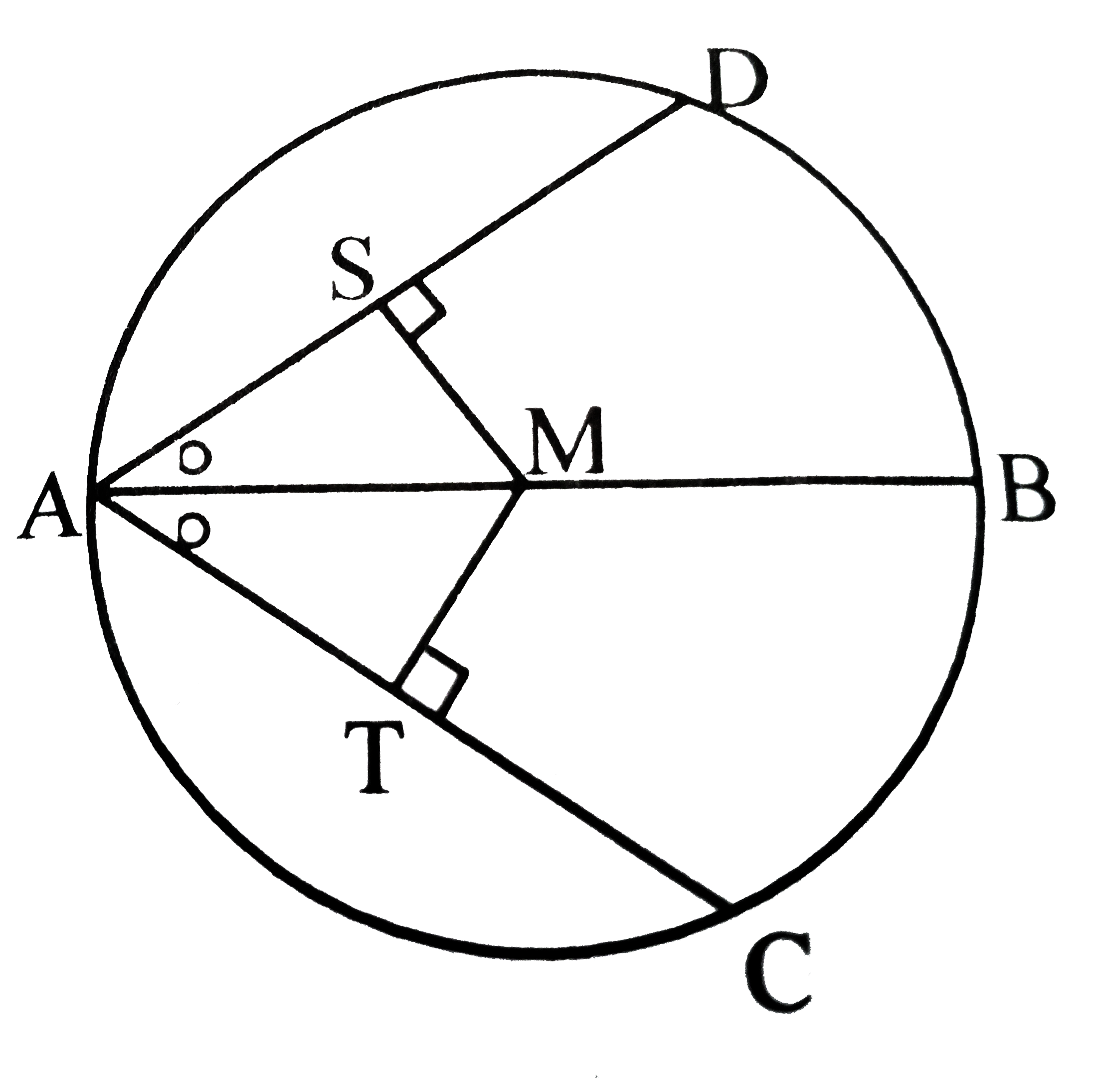
In the adjoining figure, M is the centre of the circle and seg AB is a diameter. Seg MS⊥ chord AD, seg MT ⊥ chord AC, ∠DAB≅∠CAB.
Prove that : chord AD≅ chord AC.
 Solution in Marathi
Solution in Marathi
Step by step video & image solution for In the adjoining figure, M is the centre of the circle and seg AB is a diameter. Seg MSbot chord AD, seg MT bot chord AC, angleDABcongangle CAB. Prove that : chord ADcong chord AC. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLE Book:BAL BHARTIChapter:CIRCLE Exercise:PRACTICE SET 3.4
Explore 3 Videos
- CIRCLE Book:BAL BHARTIChapter:CIRCLE Exercise:PROBLEM SET 3
Explore 13 Videos
- CIRCLE Book:BAL BHARTIChapter:CIRCLE Exercise:PRACTICE SET 3.2
Explore 4 Videos
- ARITHMETIC PROGRESSION Book:BAL BHARTIChapter:ARITHMETIC PROGRESSION Exercise:PROBLEM SET - 3
Explore 13 Videos
- CO-ORDINATE GEOMETRYBook:BAL BHARTIChapter:CO-ORDINATE GEOMETRYExercise:Problem set 5
Explore 27 Videos
Similar Questions
The radius of a circle is 9cm. Find the length of an arc of the ciircle which cuts off a chord of length equal to radius.
View SolutionTwo circles of radii 5.5 cm and 3.3 cm respectively touch each other.What is the distance between their centres?
View SolutionTwo circles having radii 3.5 cm and 4.8 cm touch each other internally.Find the distance between their centres.
View SolutionFind the 4th term from the end in an A.P, −11,−8,−5,….,49
View SolutionDetermine whether the given points are collinear.(i) A(0,2),B(1,-0.5),C(2,-3)
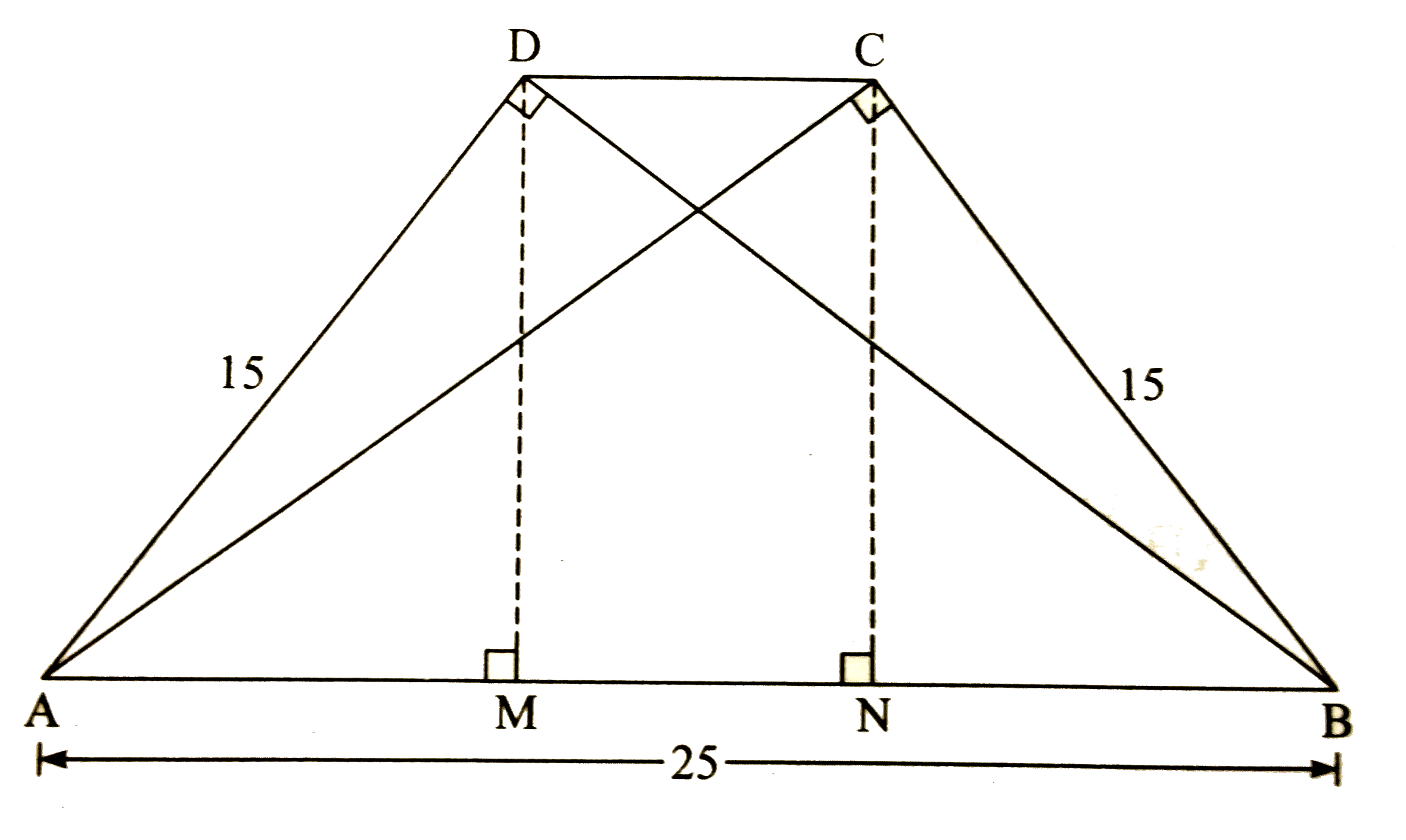
View SolutionIn a trapezium ABCD, seg AB∣∣ seg DC, seg BD⊥ seg AD, seg AC⊥ seg BC, if AD=15, BC=15 and AB=25. Find A(□ABCD).
View Solution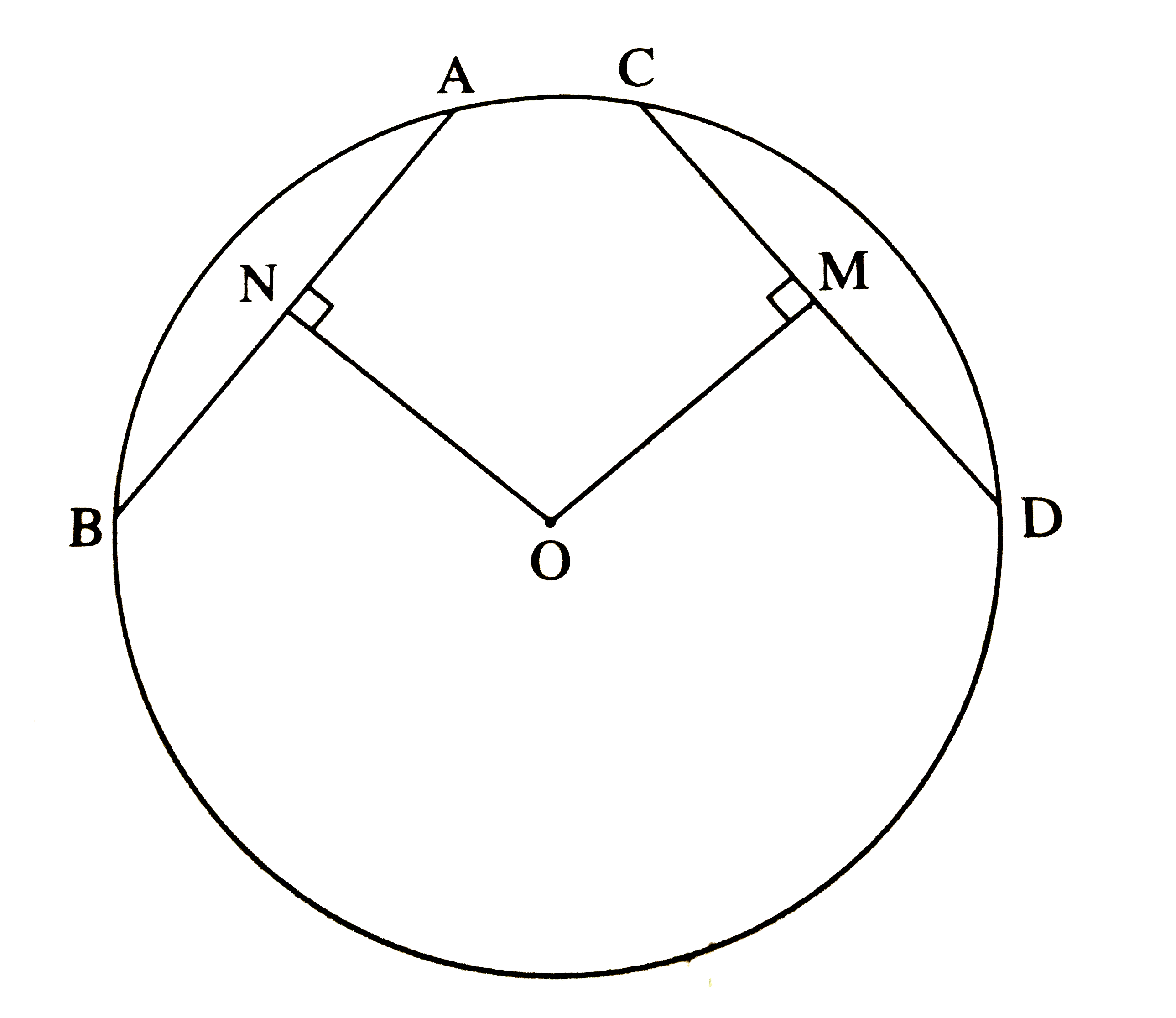
In the figure , O is the center of the circle.chord AB and CD are congruent . If seg ON ⊥ chord AB and seg OM ⊥ chord CD and OM =4cm , then find ON . Justify your answer .
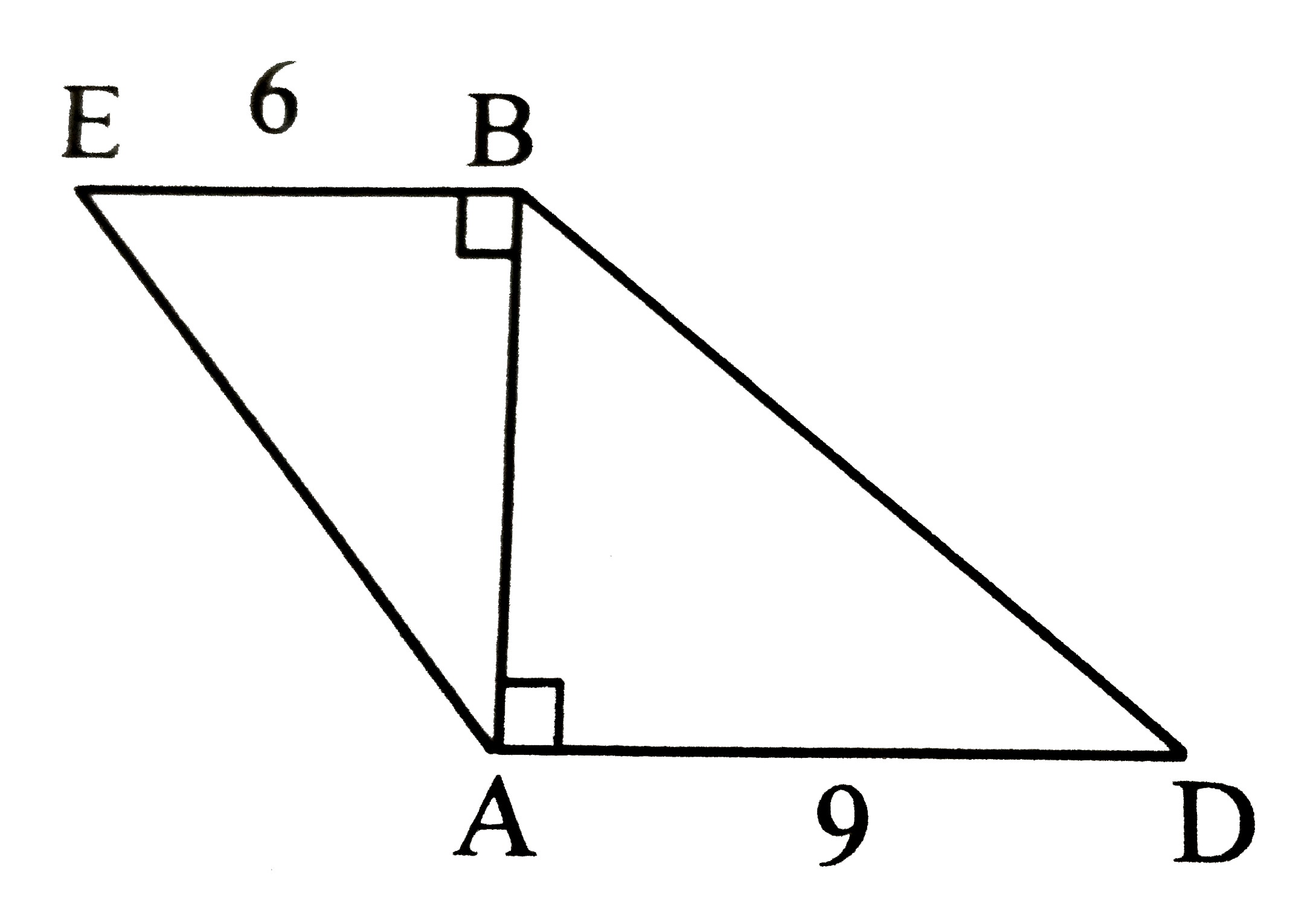
View SolutionIn the given figure, seg BE ⊥ seg AB and seg BA ⊥ seg AD. If BE = 6 and AD = 9, then find A(ΔABE)A(ΔBAD).
View SolutionIn the adjoining figure, M is the centre of the circle and seg AB is a diameter. Seg MS⊥ chord AD, seg MT ⊥ chord AC, ∠DAB≅∠CAB.
Prove that : chord AD≅ chord AC.

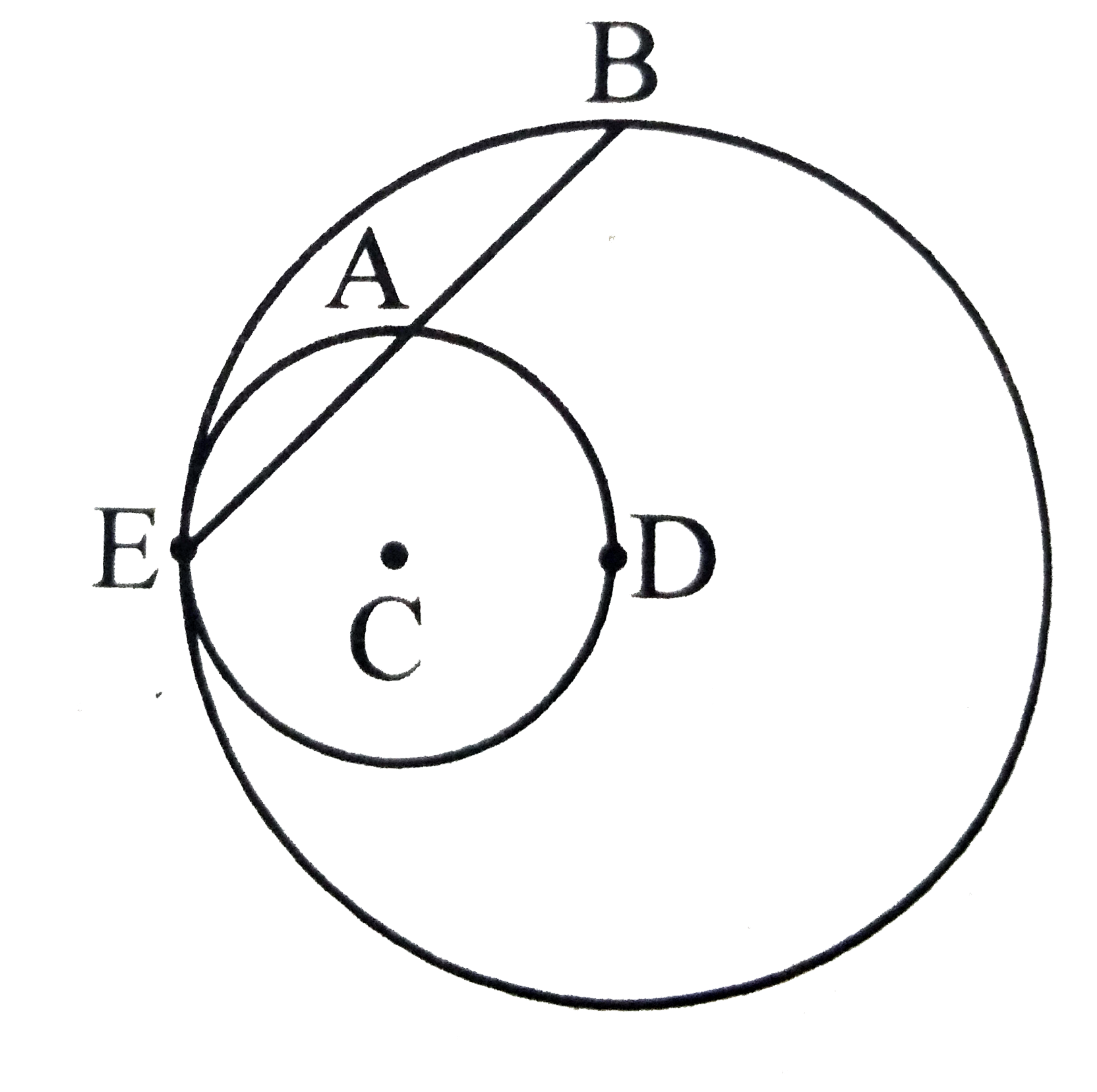
View SolutionIn the adjoining figure , circles with centres C and D touch internally at point E. D lies on the inner circle. Chord EB of the other circle intersects innet circle at point A. Prove that, seg EA≅seg AB.
View SolutionIn andsegBD bot sACandAD=DC then ……..
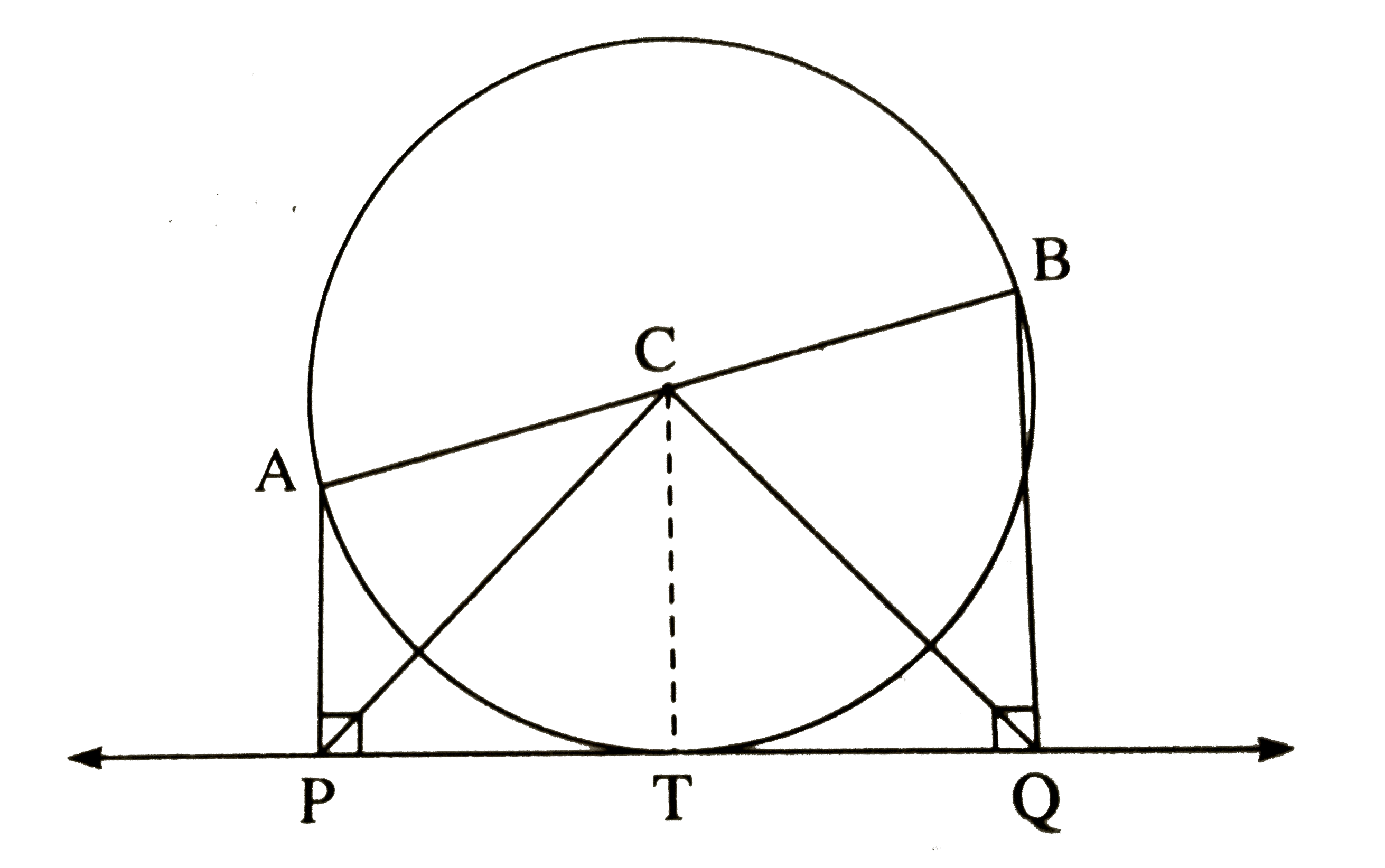
View SolutionIn the figure, seg AB is a diameter of a circle with centre C. Line PQ is a tangent, which touches the circle at point T. seg AP ⊥ line PQ and seg BQ ⊥ line PQ. Prove that, seg CP ≅ seg CQ.
View SolutionSeg AB is a diameter of a circle with centre P. Seg AC is a chord. A secant through P and parallel to seg AC intersects the tangent drawn at C in D. Prove that line DB is a tangent to the circle.
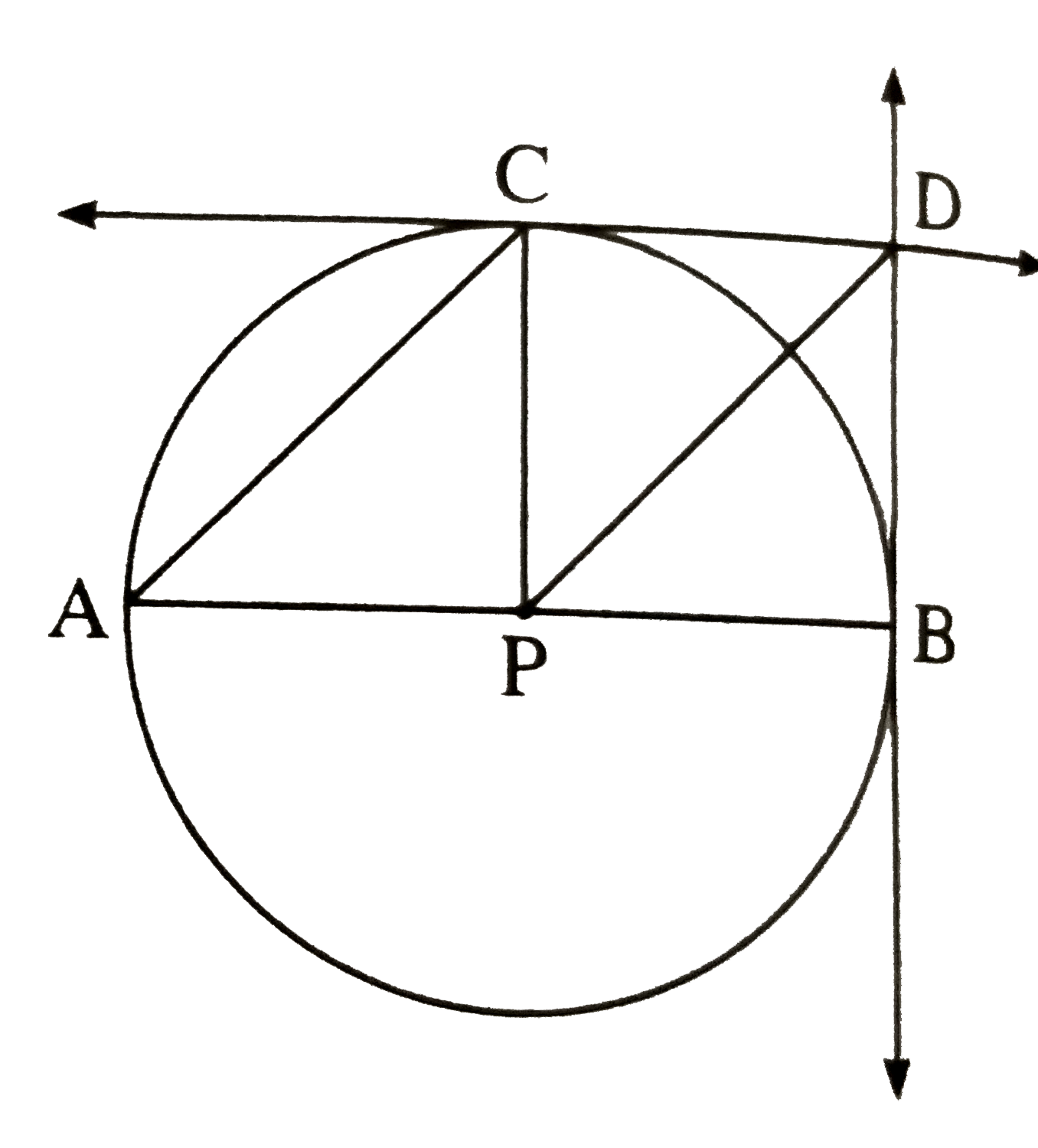
View SolutionIn the adjoining figure, seg AD ⊥ side BC, seg BE ⊥ side AC, segCf ⊥ side AB . Point O is the orthocentre. Prove that, point O is the incentre of ΔDEF
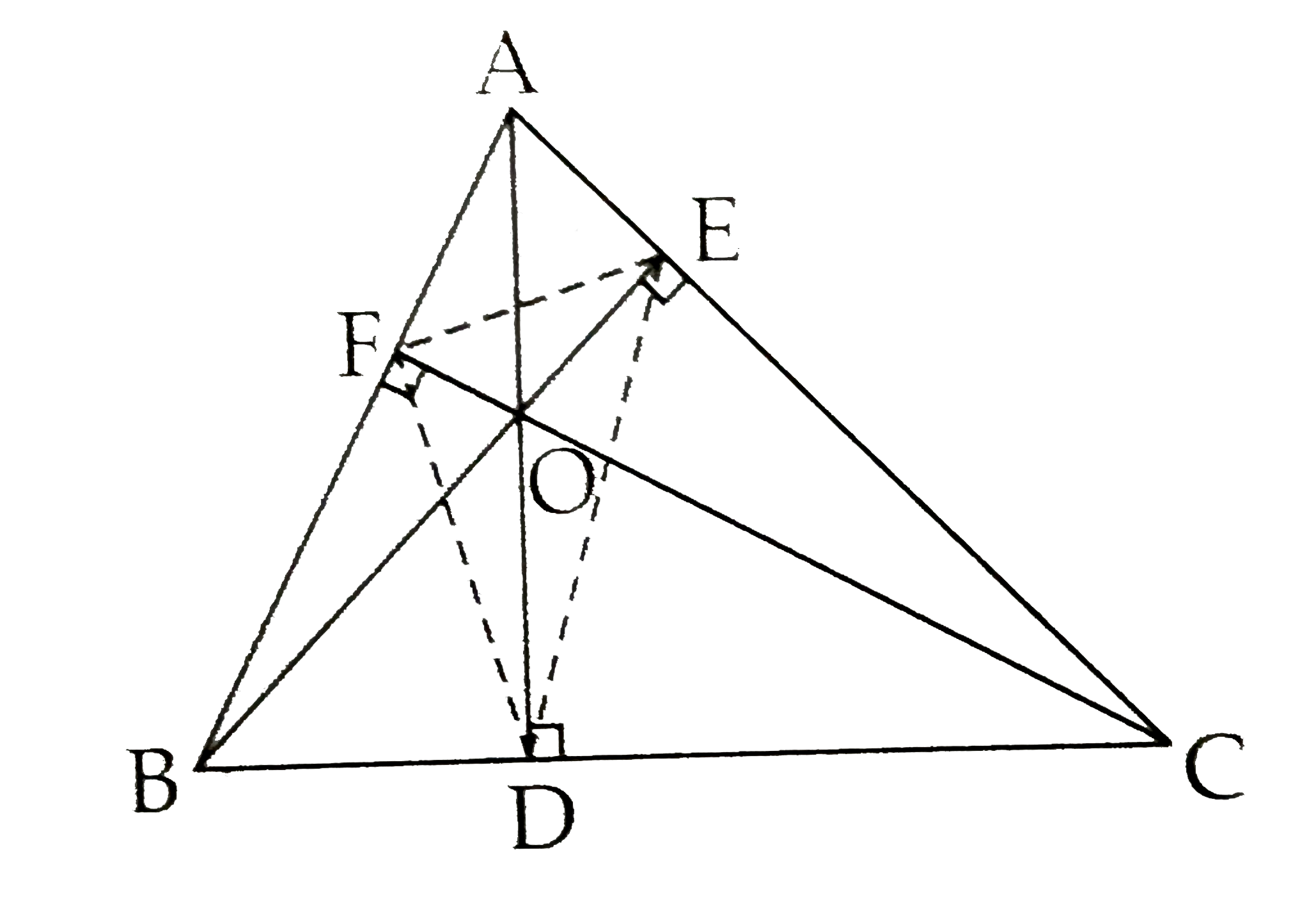
View SolutionIn trapezium ABCD, seg AB ||seg DC. Seg BD bot seg AD, seg AC bot seg BC. If AD = 15, BC = 15 and AB = 25, then find A ( square ABCD)
View SolutionIn the adjoining figure, seg AB is a chord of a circle with centre P. If PA=8cm and distance of the chord of from the centre P is 4cm. Find the area of the shaded portion.
View Solution