The number of students who take both the subjects Mathematics and Chemistry is 70. This represents 10% of the enrollment in Mathematics and 14% of the enrollment in Chemistry. The number of student take at least one of these two subjects is
1120
1130
1100
insufficient data
The correct Answer is:B
Topper's Solved these Questions
- SETS, RELATIONS AND FUNCTIONSBOOK - NCERT TAMILCHAPTER - SETS, RELATIONS AND FUNCTIONSEXERCISE - EXERCISE 1.3
21 Videos
- SETSBOOK - NCERT TAMILCHAPTER - SETSEXERCISE - MISCELLANEOUS EXERCISE ON CHAPTER 1
26 Videos
- STATISTICSBOOK - NCERT TAMILCHAPTER - STATISTICSEXERCISE - Miscellaneous Exercise On Chapter 15
7 Videos
Similar Questions
A class has 175 students. The following table shows the number of students studying one or more of the following subjects in this case.
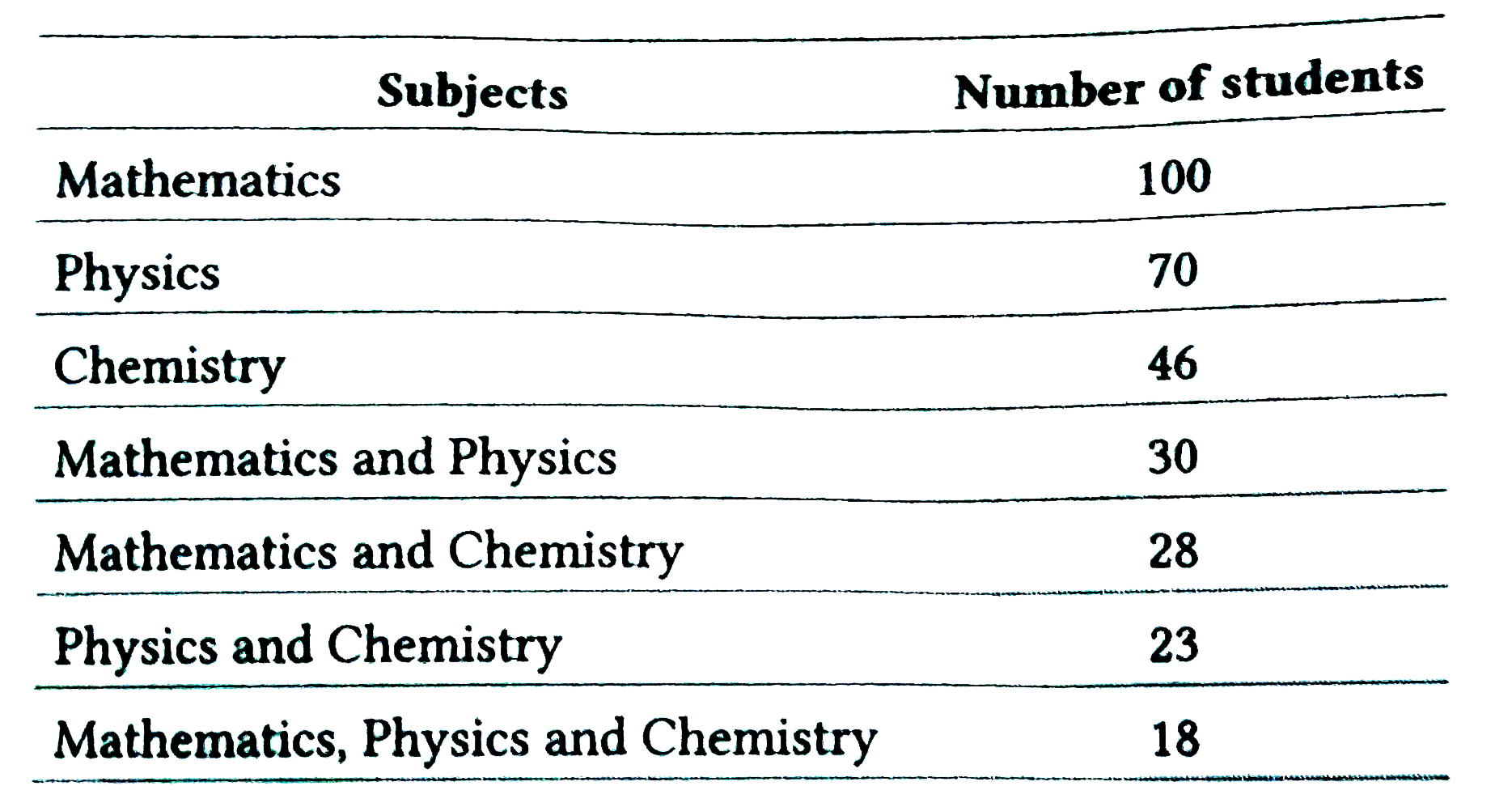
How many students are enrolled in Mathematics alone, Physics alone and Chemistry alone? Are there students who have not offered any one of these subjects?
View SolutionA class has 175 students. The following table shows the number of students studying one or more of the following subjects in this case.

How many students are enrolled in Mathematics alone, Physics alone and Chemistry alone? Are there students who have not offered any one of these subjects?
View Solution
Knowledge Check
The number of students who take both the subjects mathematics and chemistry is 30. This represents 10% of the enrolment in mathematics and 12% of the enrolment in chemistry. How many students take at least one of these two subjects?
A520B490C560D480A class has 172 students. The following data shows the number of students opting one or more subjects: Mathematics 125, Physics 95, Chemistry 65 Mathematics and Physics 55
Mathematics and Chemistry 53
Physics and Chemistry 48 Mathematics, Physics and Chemistry 43
The number of students who opted only Physics isA35B45C29D37In the class of 175 students, the following data shows the number of students opting one or more subjects.
Mathematics 100, Physics 70, Chemistry 40, Mathematics and Physics 30, Mathematics and Chemistry 28, Physics and Chemistry 23, Mathematics, Physics and Chemistry 18.
Than, number of students opted Mathematics alone isA35B48C60D20
In a class of 80 students, 55 students passed in mathematics and 60 passed in chemistry. 10 stu-dents failed in both mathematics and chemistry.
(i) How many students passed in both the subjects ?
(ii) How many students passed in exactly one of the two subjects ?
View SolutionA class has 175 students, The following description gives the number of students studying one or more of the subjects in this class, Mathematics 100, physics 70, chemistry 46, Mathematics and physics 30, mathematics and chemistry 28, physics and chemistry 23, mathematics, physics and chemistry alone 18,
(i) how many students are enrolled in mathematics alone, physics alone and children alone,
(ii) the number of students who have not offered any of these subjects.
View SolutionIn a survey of 200 students of a school, it was found that 120 study Mathematics, 90 study Physics and 70 study Chemistry , 40 study Mathematics and Physics, 30 study Physics and Chemistry, 50 study Chemistry and Mathematics and 20 none of these subjects. Find the number of students who study all the three subjects.
View SolutionIn a survey of 200 students of a school, it was found that 120 study Mathematics, 90 study Physics and 70 study Chemistry , 40 study Mathematics and Physics, 30 study Physics and Chemistry, 50 study Chemistry and Mathematics and 20 none of these subjects. Find the number of students who study all the three subjects.
View SolutionIn a survey of 25 students, it was found that 15 had taken Mathematics, 12 had taken Physics and 11 had taken Chemistry, 5 had taken Mathematics and Chemistry, 9 had taken Mathematics and Physics, 4 had taken Physics and Chemistry and 3 had taken all the three subjects.
Consider the following statements :
1. The number of students who had taken only one subject is equal to the number of students who had taken only two subjects.
2. The number of students who had taken at least two subjects is four times the number of students who had taken all the three subjects.
Which of the above statements is/are correct ?
View Solution
