The function f:R→R is defined by f(x)=sinx+cosx is
an odd function
neither an odd function nor an even function
an even function
both odd function and even function.
The correct Answer is:B
Topper's Solved these Questions
- SETS, RELATIONS AND FUNCTIONSBOOK - NCERT TAMILCHAPTER - SETS, RELATIONS AND FUNCTIONSEXERCISE - EXERCISE 1.3
21 Videos
- SETSBOOK - NCERT TAMILCHAPTER - SETSEXERCISE - MISCELLANEOUS EXERCISE ON CHAPTER 1
26 Videos
- STATISTICSBOOK - NCERT TAMILCHAPTER - STATISTICSEXERCISE - Miscellaneous Exercise On Chapter 15
7 Videos
Similar Questions
Knowledge Check
Which one of the following is correct in respect of the function f:R→R+ defined as f(x)=|x+1|?
Af(x)2=[f(x)]2Bf(|x|)=|f(x)|Cf(x+y)=f(x)+f(y)DNone of the aboveThe function f defined by f(x)=4sinx−2x−xcosx2+cosx,0≤x≤2π is :
Aincreasing in (0,π2) and decreasing in (3π2,2π).Bincreasing in (−π2,0) and decreasing in (π2,3π2)∪(3π2,2π)Cincreasing in (3π2,2π) and decreasing in (0,π2)∩(π2,3π2).Dincreasing in (0,π2)∪(3π2,2π)and decreasing in (π2,3π2).The function f(x)=sinx+cosx will be
Aan even functionBan odd functionCa constant functionDNone of these
Let f:X→Y be a function defined by f(x)=sinx.
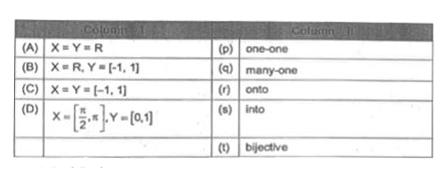
View SolutionSometimes functions are defined like f(x)=max{sinx,cosx}, then f(x) is splitted like f(x)=⎧⎪⎨⎪⎩cosxx∈(0,π4]sinxx∈(π4,π2] etc.
If f(x)=max{12,sinx}, then f(x)=12 is defined when x∈
View SolutionSometimes functions are defined like f(x)=max{sinx,cosx}, then f(x) is splitted like f(x)=⎧⎪⎨⎪⎩cosxx∈(0,π4]sinxx∈(π4,π2] etc.
If f(x)=max{x2,2x},then if x∈(0,1), f(x)=
View SolutionSometimes functions are defined like f(x)=max{sinx,cosx}, then f(x) is splitted like f(x)=⎧⎪⎨⎪⎩cosxx∈(0,π4]sinxx∈(π4,π2] etc.
If f(x)=max{12,sinx}, then f(x)=12 is defined when x∈
View SolutionSometimes functions are defined like f(x)=max{sinx,cosx}, then f(x) is splitted like f(x)=⎧⎪⎨⎪⎩cosxx∈(0,π4]sinxx∈(π4,π2] etc.
If f(x)=max{x2,2x},then if x∈(0,1), f(x)=
View Solution
