If E , F G and H are respectively the mid -points of the sides of a parallelogram ABCD , show that ar (EFGH) =12 ar (ABCD).Solution in Punjabi
The correct Answer is:=12 ar(ABCD)
Step by step video, text & image solution for If E , F G and H are respectively the mid -points of the sides of a parallelogram ABCD , show that ar (EFGH) =(1)/(2) ar (ABCD). by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- AREAS OF PARALLELOGRAMS AND TRIANGLES BOOK - SWAN PUBLICATIONCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLES EXERCISE - EXERCISE 9.3
21 Videos
- AREAS OF PARALLELOGRAMS AND TRIANGLES BOOK - SWAN PUBLICATIONCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLES EXERCISE - EXERCISE 9.4
21 Videos
- AREAS OF PARALLELOGRAMS AND TRIANGLES BOOK - SWAN PUBLICATIONCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLES EXERCISE - OBJECTIVE TYPE QUESTIONS
20 Videos
- CIRCLESBOOK - SWAN PUBLICATIONCHAPTER - CIRCLESEXERCISE - Objective Type Questions (Fill in the Blanks :)
10 Videos
Similar Questions
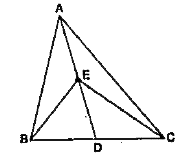
In Fig ., E is any point on median AD of a ΔABC . Show that ar (ABE = ar (ACE) .
View SolutionParallelogram ABCD and rectangle ABEF are on the same base AB and have equal areas. Show that the perimeter of the parallelogram is greater than that of the rectangle.
View SolutionState whether the following statements are true (T) or false (F) :
If A and B are two congruent figures then their areas will be equal .
View Solution............... is the longest chord of the circle.
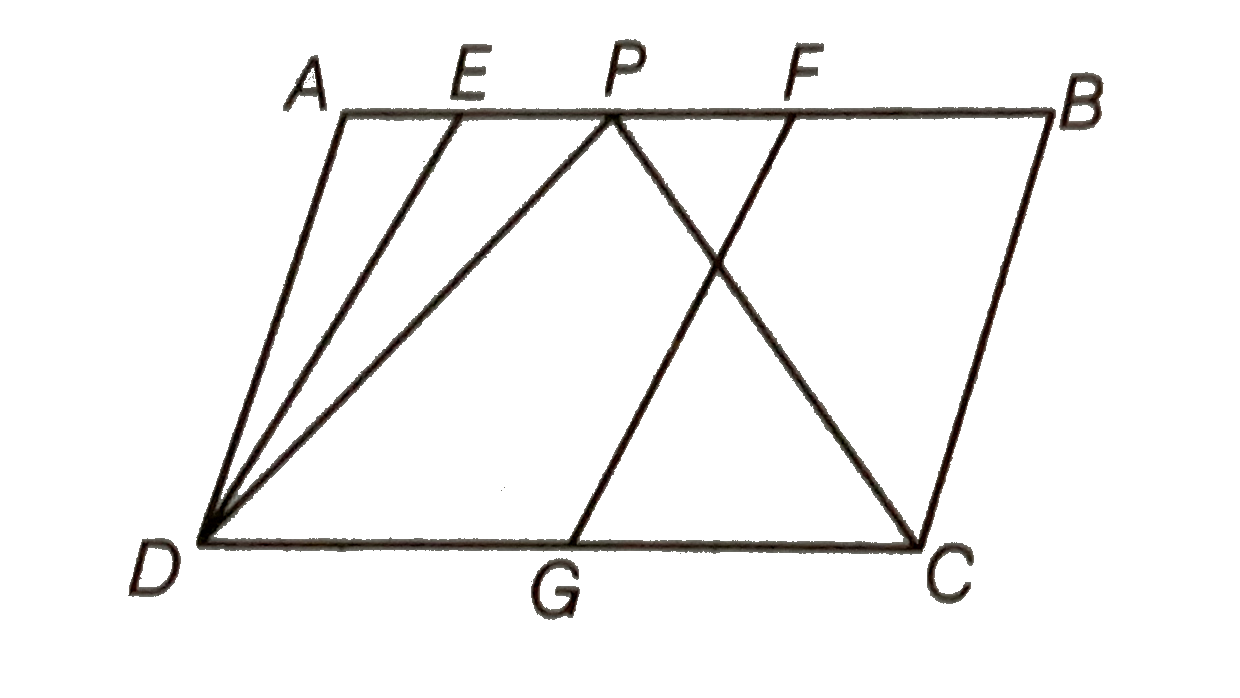
View SolutionIn the figure, ABCD and EFGD are two parallelograms and G is the mid-point of CD. Then, ar(ΔDPC)=ar(EFGD).
View Solutionयदि E,F,G और H क्रमश : समांतर चतुर्भुज ABCD कि भुजाओ के मध्य - बिंदु है, तो दर्शाइए कि ar(EFGH)=12ar(ABCD) है।
View Solutionयदि E, F, G और H क्रमशः समांतर चतुर्भुज ABCD की भुजाओ के मध्य-बिन्दु है, तो दर्शाइए कि (EFGH) 12 ar (ABCD) है।
View SolutionP और Q क्रमशः समांतर चतुर्भुज ABCD की भुजाओं DC और AD पर बिन्दु है। दर्शाइए कि ar (APB) = ar (BQC) है।
View Solutionसमांतर चतुर्भुज ABCD में भुजाओं AB तथा BC पर कोई दो बिंदु क्रमशः E तथा F है, सिद्ध कीजिए कि ar(ΔADF)=ar(ΔDCE).
View SolutionABCD एक समांतर चतुर्भुज है भुजाओं BC तथा CD के मध्य-बिंदु क्रमशः X तथा Y है। सिद्ध कीजिए कि ar(ΔAXY)=38ar(।।gmABCD)
View Solutionयदि E, F, G और H क्रमशः समान्तर चतुर्भुज ABCD की भुजाओं के मध्य-बिन्दु है, तो दर्शाइए कि ar(EFGH)=12ar(ABCD)
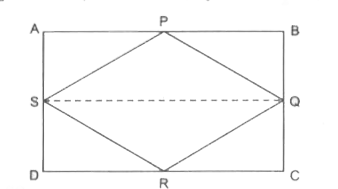
View SolutionIF P,Q,R and S are respectively the mid-points of the sides of a parallelogram ABCD show are (PQRS) = 12 ar (ABCD)
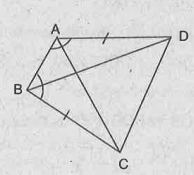
View SolutionABCD చతుర్భుజంలో AD=BC మరియు ∠DAB=∠CBA అయిన BD=AC
View SolutionIf E, F G and H are respectively the midpoints of the sides AB, BC, CD and AD of a parallelogram ABCD, show that ar(EFGH) =12 ar (ABCD) .
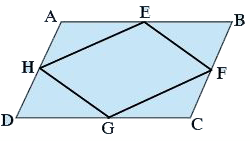
View SolutionIf E, F G and H are respectively the midpoints of the sides AB, BC, CD and AD of a parallelogram ABCD, show that ar(EFGH) =12 ar (ABCD) .
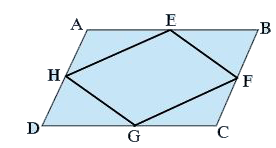
View SolutionIf E, F G and H are respectively the midpoints of the sides AB, BC, CD and AD of a parallelogram ABCD, show that ar(EFGH) =12 ar (ABCD) .
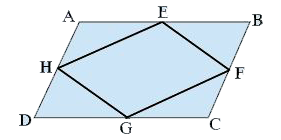
View Solution
