A network of resistors is connected to a 15 V battery with internal resistance 1Ω as shown in the circuit diagram.
Current in each resistor,
Current in each resistor :
Total current I in the circuit is,
I=εRT+r=146+1=2
Consider resistors between A and B.
Let I1 be the current through one of the 4Ω resistors and I2 be the current in the other resistor
I1×4=I2×4
that is, I1=I2 from symmetry of the two arms.
But I1+I2=I=2A
∴I1=I2=1A
∴I1=I2=1A
that is, the current in each 4Ω resistor is 1A, the current in 1Ω resistor between B and C would be 2A.
Now, consider the resistances between C and D
Let I3 be the current through one of the 6 Ω resistors and I4 be the current in the other resistor.
I3×6=I4×6
∴I3=I4=1A
That is, current in each 6Ω resistor is 1A
Similar Questions
A network of resistors is connected to a 5 V battery with internal resistance of 1Ω as shown in Fig. (a) Compute the equivalent resistance of the network. (6) Obtain the current in each resistor. (c) Obtain the voltage drops VBC and VCD
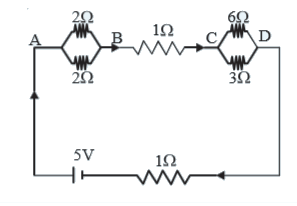
View SolutionA network of resistors is connected to a 5 V battery with internal resistance of 1Ω as shown in Fig. (a) Compute the equivalent resistance of the network. (6) Obtain the current in each resistor. (c) Obtain the voltage drops VBC and VCD

View Solution
Knowledge Check
A series combination of two resistors 1Ω each is connected to a 12 V battery of internal resistance 0.4Ω. The current flowing through it will be
A3.5AB5AC6AD10AA 5V battery with internal resistance 2Ω and a 2V battery with internal resistance1Ω are connected to a 10Ω resistor as shown in the figure.
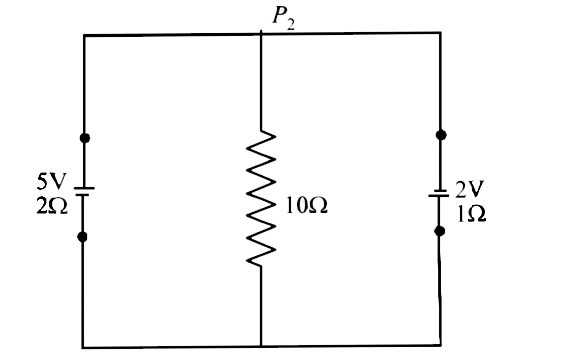
The current in the 10Ω resistor isA0.27AP2→P1B0.03AP1→P2C0.03AP2→P1D0.27AP1→P2
A network of resistances is connected to a 16 V battery with internal resistance of 1Ω as shown in figure. (a) Compute the equivlaent resistance of the network. (b) Obtain the current in each resistor and (c ) obtain the voltage dropVAB,VBC and VCD.
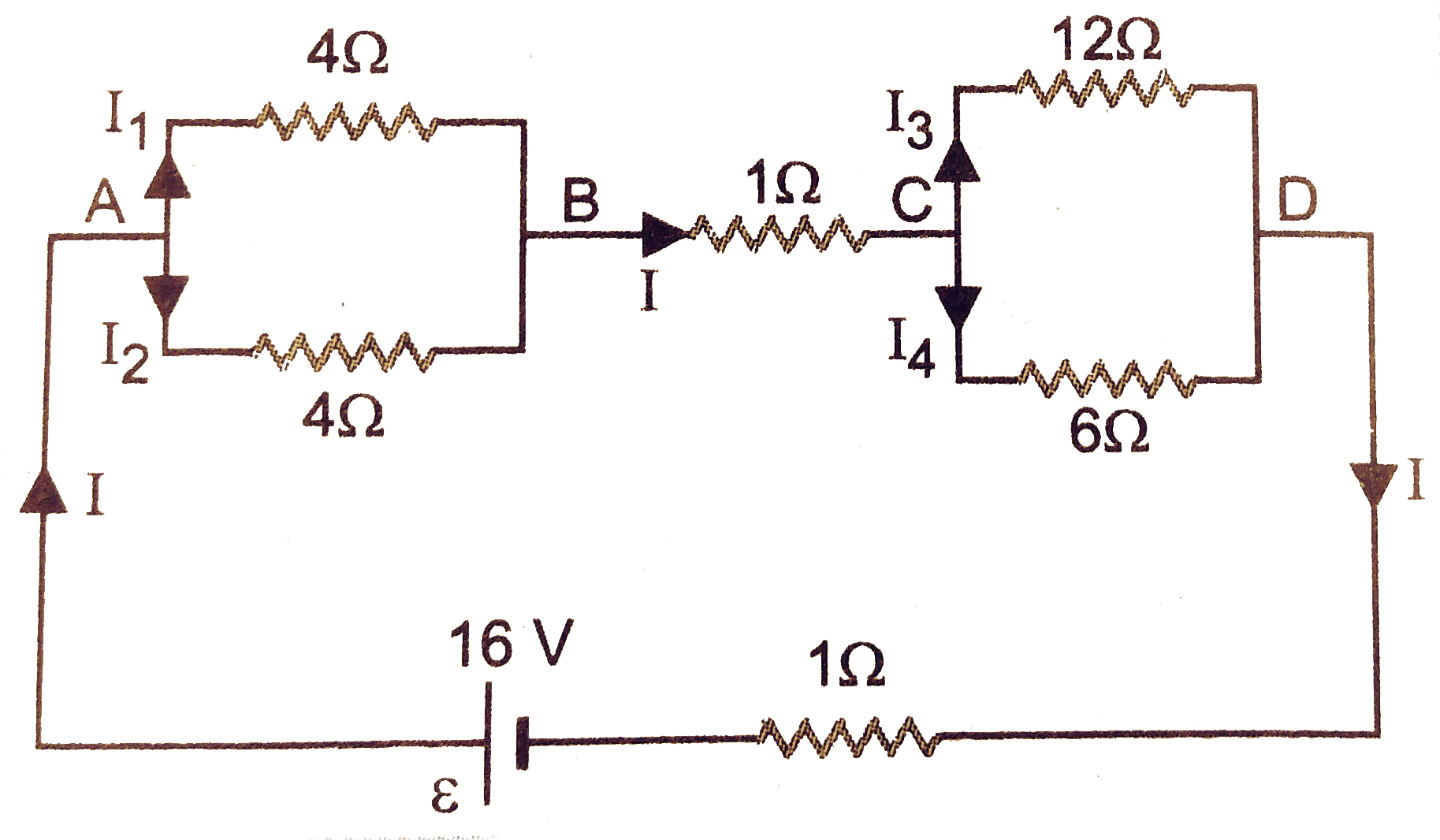
View SolutionA network of resistances is connected to a 16 V battery with internal resistance of 1Ω, as shown in Fig. 4.33.

(a) Compute epuivalent resistance of the network,
(b) obtain the current In in each resistor, and
(c ) obtain the voltage drops VAB,VBC and VCD.
View SolutionA network of resistances is connected to a 16 V battery with internal resistance of 1Ω, as shown in Fig. 4.33.

(a) Compute epuivalent resistance of the network,
(b) obtain the current In in each resistor, and
(c ) obtain the voltage drops VAB,VBC and VCD.
View SolutionA 5V battery with internal resistance 2Ω and a 2V battery with internal resistance1Ω are connected to a 10Ω resistor as shown in the figure.

The current in the 10Ω resistor is
View SolutionA resistor is connected to a battery of emf 10 V and internal resistance 0.3Ω. What is the resistance of the resistor to be inserted in the circuit for the circuit current 1.2A?
View SolutionTwo resistors of 4 Ω and 6 Ω are connected in parallel. The combination is connected across a 6 V battery of negligible resistance. (i) Draw a circuit diagram. (ii) Calculate :the current through each resistor
View Solution
