Let X be a continuous random variable whose probability density function is f(x)=x34 for an interval 0<x<x . What is the value of the constant c that makes f (x) a valid probability density function ?
Text Solution
The correct Answer is:2 since c must be positive
|
Similar Questions
Knowledge Check
X is continuous random variable with probability density function f(x)=x28,0≤x≤1. Then, the value of P(0.2≤X≤0.5) is
A0.11724B0.11224C0.11336D0.11236Let X is a continuous random variable with probability density function
f(x)={x6+k0≤x≤30 otherwise
The value of k is equal toA112B13C14D16If a c.r.v. X has the probability density function
f(x)=C(9−x2),0<x<3
= 0 , otherwise
Then, the value of C isA116B115C218D118
Following is the probability density function f(x)=pxe−4x2,0≤x≤∞ . Then the value of p is
View SolutionIf the probability density function of a random variable X is f(x)=x2 in 0≤x≤2, then P(X>1.5∣X>1) is equal to
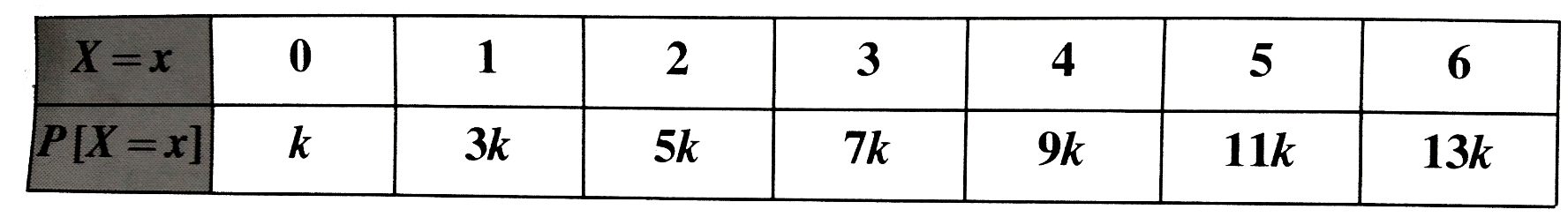
View SolutionA random variable X has the probability distribution

For the events E = { X is prime number} and F={x<4} then P(E∪F) is
View SolutionThe p.d.f of a continuous random variable X is f(x)=x23,−1<x<2 0 = otherwise Then the c.d.f of X is
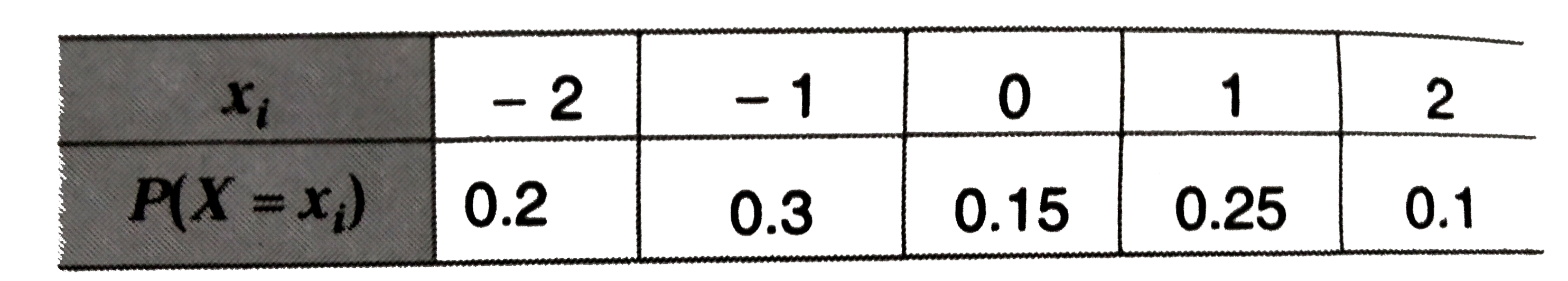
View SolutionA random variable X has the probability distribution:

For the events E = (X is prime number) and F = {X < 4}, the probability P(E∪F) is
View Solution