ABC is any triangle, D, E, F are the mid points of the sides ¯¯¯¯¯¯AB,¯¯¯¯¯¯BCand¯¯¯¯¯¯CA respectively. Then areΔDEF:arΔABC is
A
1:2
B
1:3
C
2:3
D
1:4
Text Solution
The correct Answer is:D
|
Similar Questions
Knowledge Check
ABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :
A4:1B5:1C3:1Dcan't be determindABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :
A4:1B5:1C3:1Dcan't be determinedIn a triangle ABC, D, E, F are the mid-points of the sides BC, CA and AB respectively , the vector →AD is equal to
A→BE+→CFB→BE−→CFC→CF−→BED−→BE−→CF
D, E and F are the mid-points of the sides BC, CA and AB respectively of triangle ABC. Prove that:
area of BDEF is half the area of ΔABC.
View SolutionIf D, E, F are the mid-points of the sides BC, CA and AB respectively of △ABC, then ¯¯¯¯¯¯AD+23¯¯¯¯¯¯BE+13¯¯¯¯¯¯CF=
View SolutionABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
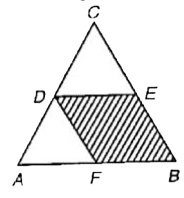
View SolutionIn a ΔABC,D,E,F are the mid -points of the sides BC, CA and AB respectively, the vector AD is equal to
View SolutionIf A=B=C=1 and X=¯¯¯¯¯¯¯¯¯ABC+B¯¯¯¯¯¯BC+C¯¯¯¯¯¯AB, then X=
View Solution
