Read the following text and answer the following questions on the basis of the same :

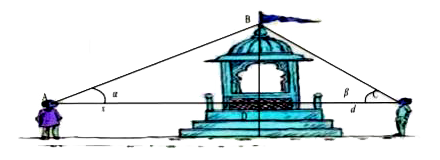
Two men on either sider of a temple of 30 metres high observe its top at the angles of elevation α and β respectively, (as shown in the figure above).
The distance between the tow men is 40√3 metres and the distance between the first person A and the temple is 30√3 meters.
∠BCA=β=
tan−1(12)
tan−1(2)
tan−1(1√3)
tan−1(√3)
The correct Answer is:D
Step by step video & image solution for Read the following text and answer the following questions on the basis of the same : Two men on either sider of a temple of 30 metres high observe its top at the angles of elevation alpha and beta respectively, (as shown in the figure above). The distance between the tow men is 40sqrt3 metres and the distance between the first person A and the temple is 30sqrt3 meters. angleBCA=beta= by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Read the following text and answer the following questions on the basis of the same :

Two men on either sider of a temple of 30 metres high observe its top at the angles of elevation α and β respectively, (as shown in the figure above).
The distance between the tow men is 40√3 metres and the distance between the first person A and the temple is 30√3 meters.
∠CAB=α=
View SolutionRead the following text and answer the following questions on the basis of the same :

Two men on either sider of a temple of 30 metres high observe its top at the angles of elevation α and β respectively, (as shown in the figure above).
The distance between the tow men is 40√3 metres and the distance between the first person A and the temple is 30√3 meters.
∠CAB=α=
View Solution
Knowledge Check
If the shadow of a pole 3 metre high is 3√3 metre long then the angle of elevation of the sun is
A30∘B45∘C60∘D75∘
Read the following text and answer the following questions on the basis of the same :

Two men on either sider of a temple of 30 metres high observe its top at the angles of elevation α and β respectively, (as shown in the figure above).
The distance between the tow men is 40√3 metres and the distance between the first person A and the temple is 30√3 meters.
Domain and Range of cos−1x=
View SolutionTwo men on either side of a temple of 30 meters high observe its top at the angles of elevation α and β? respectively. (as shown in the figure above). The distance between the two men is 40√3 meters and the distance between the first person A and the temple is30√3 meters. Based on the above information answer the following:

∠BCA=β=
View SolutionTwo men on either side of a temple of 30 meters high observe its top at the angles of elevation? α and beta respectively. (as shown in the figure above). The distance between the two men is 40√3 meters and the distance between the first person A and the temple is30√3 meters. Based on the above information answer the following:

∠CAB=α=
View SolutionTwo men on either side of a temple of 30 meters high observe its top at the angles of elevation α and β respectively. (as shown in the figure above). The distance between the two men is 40√3 meters and the distance between the first person A and the temple is30√3 meters. Based on the above information answer the following:

∠CAB=α=
View SolutionTwo men on either side of a temple of 30 meters high observe its top at the angles of elevation α and β? respectively. (as shown in the figure above). The distance between the two men is 40√3 meters and the distance between the first person A and the temple is30√3 meters. Based on the above information answer the following:

∠ABC=
View SolutionRead of the following text and answer the following questions on the basis of the same:
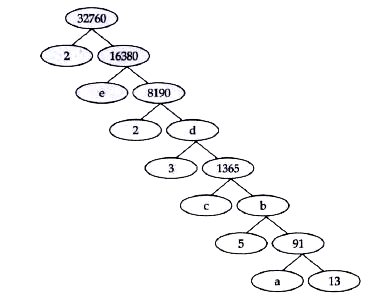
Find the value of a
View SolutionTwo men on either side of a temple of 30 meters high observe its top at the angles of elevation α and β? respectively. (as shown in the figure above). The distance between the two men is 40√3 meters and the distance between the first person A and the temple is30√3 meters. Based on the above information answer the following:
Domain and Range of cos−1x=
View Solution
