Form the pair of linear equations in the following problems, and find their solutions graphically.
10 students of Class X took part in a Mathematics quiz. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quiz.
To solve the problem, we need to form a pair of linear equations based on the information given and then find their solutions graphically.
Step 1: Define Variables
Let:
- x = number of girls
- y = number of boys
Step 2: Form the Equations
From the problem, we have two pieces of information:
1. The total number of students (girls + boys) is 10.
2. The number of girls is 4 more than the number of boys.
We can express these statements as equations:
1. x+y=10 (Equation 1)
2. x=y+4 (Equation 2)
Step 3: Substitute Equation 2 into Equation 1
Now, we can substitute Equation 2 into Equation 1 to find the values of x and y:
(y+4)+y=10
2y+4=10
2y=10−4
2y=6
y=3
Step 4: Find the Value of x
Now that we have y, we can find x using Equation 2:
x=y+4
x=3+4
x=7
Step 5: Conclusion
Thus, the number of boys is y=3 and the number of girls is x=7.
Step 6: Graphical Representation
To represent the equations graphically, we can rewrite them in slope-intercept form:
1. From x+y=10:
y=10−x
2. From x=y+4:
y=x−4
Step 7: Plot the Equations
- For y=10−x:
- If x=0, then y=10 (point (0, 10))
- If y=0, then x=10 (point (10, 0))
- For y=x−4:
- If x=4, then y=0 (point (4, 0))
- If y=0, then x=4 (point (4, 0))
Step 8: Find the Intersection Point
Plotting these two lines on a graph will show that they intersect at the point (7, 3), confirming our solution.
Final Answer
The number of boys is 3 and the number of girls is 7.
---
Let:
- x = number of girls
- y = number of boys
From the problem, we have two pieces of information:
1. The total number of students (girls + boys) is 10.
2. The number of girls is 4 more than the number of boys.
1. x+y=10 (Equation 1)
2. x=y+4 (Equation 2)
Now, we can substitute Equation 2 into Equation 1 to find the values of x and y:
(y+4)+y=10
2y+4=10
2y=10−4
2y=6
y=3
Now that we have y, we can find x using Equation 2:
x=y+4
x=3+4
x=7
Thus, the number of boys is y=3 and the number of girls is x=7.
To represent the equations graphically, we can rewrite them in slope-intercept form:
y=10−x
y=x−4
- For y=10−x:
- If x=0, then y=10 (point (0, 10))
- If y=0, then x=10 (point (10, 0))
- If x=4, then y=0 (point (4, 0))
- If y=0, then x=4 (point (4, 0))
Plotting these two lines on a graph will show that they intersect at the point (7, 3), confirming our solution.
The number of boys is 3 and the number of girls is 7.
Topper's Solved these Questions
- PAIR OF LINEAR EQUATIONS IN TWO VARIABLESBook:OSWAL PUBLICATIONChapter:PAIR OF LINEAR EQUATIONS IN TWO VARIABLESExercise:NCERT CORNER (Exercise 3.3)
Explore 13 Videos
- PAIR OF LINEAR EQUATIONS IN TWO VARIABLESBook:OSWAL PUBLICATIONChapter:PAIR OF LINEAR EQUATIONS IN TWO VARIABLESExercise:NCERT CORNER (Exercise -3.4)
Explore 9 Videos
- PAIR OF LINEAR EQUATIONS IN TWO VARIABLESBook:OSWAL PUBLICATIONChapter:PAIR OF LINEAR EQUATIONS IN TWO VARIABLESExercise:NCERT CORNER (Exercise -3.1)
Explore 3 Videos
- OLYMPIAD 2019-20Book:OSWAL PUBLICATIONChapter:OLYMPIAD 2019-20Exercise:15. Probability
Explore 1 Video
- PAIR OF LINEAR EQUTIONS IN TWO VARIABLESBook:OSWAL PUBLICATIONChapter:PAIR OF LINEAR EQUTIONS IN TWO VARIABLESExercise:Case-Based MCQs
Explore 15 Videos
Similar Questions
Form the pair of linear equations in the following problems, and find their solutions graphically. (i) 10 students of Class X took part in a Mathematics quiz. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quiz.
View SolutionFrom the pair of linear equations in the following problems, and find their solutions graphically.
(i)10 students of Class X took pan in a Mathematics quiz. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quiz.
(ii)5 pencils and 7 pens together cost Rs 50, whereas 7 pencils and 5 pens together cost Rs 46. Find the cost of one pencil and that of one pen.
View Solution
Knowledge Check
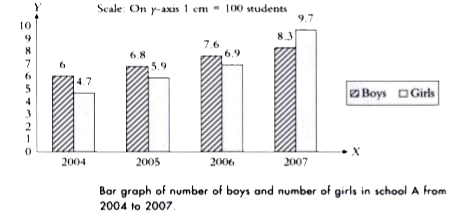
In which year the number of girls is more than the number of boys?A2004B2005C2006D2007
Form the pair of linear equations in the following problem, and find the solution graphically: 10 students of class X took part in Mathematics quiz. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quiz.
View SolutionFrom the pairs of linear equation in this problem and find its solution graphically 10 students class x took park in mathematics quize. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quize
View SolutionIn class VI, there are 30 students. If the number of girls is 3 more than twice the number of boys, then the number of boys and girls are :
View SolutionTen students of class X took part in Mathematics quiz. If the number of girls is 4 more than the number of boys. Represent this situation algebraically and graphically.
View SolutionIn a class of 60 students, the number of girls is one third the number of boys. Find the number of girls and boys in the class.
View SolutionThe number of boys and girls in a class are in the ratio 5:4. If the number of boys is 9 more than the number of girls, then number of boys is 9.
View SolutionIn a school, the number of girls is 50 more than the number of boy The total number of students is 1070. Find the number of girls.
View Solution