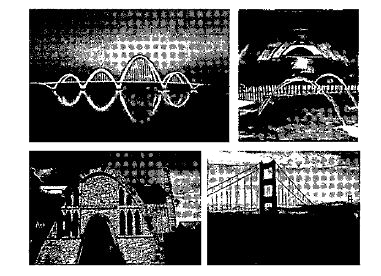
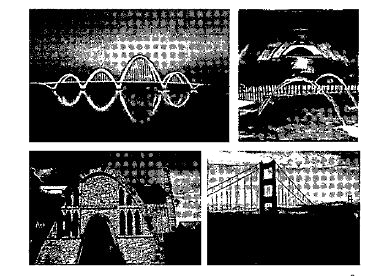
The below pictures are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arc is an arc in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and the architecture in a variety of forms.
If the sum of the roots is −p and the product of the roots is −1p then the quadratic polynomial is

k(−px2+xp+1)
k(px2−xp−1)
k(x2+px−1p)
k(x2−px+1p)
The correct Answer is:C
Step by step video & image solution for The below pictures are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arc is an arc in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and the architecture in a variety of forms. If the sum of the roots is -p and the product of the roots is (-1)/p then the quadratic polynomial is by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Similar Questions
The below picture are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.

If the sum of the roots is -p and product of the roots is −1p , then the quadratic polynomial is
View SolutionThe below pictures are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arc is an arc in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and the architecture in a variety of forms
The graph of x2+1
View SolutionThe below picture are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.

The graph of x2+1=0
View SolutionThe below pictures are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arc is an arc in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and the architechture in a variety of forms.
In the standard form of quadratic polynomial ax2+bx+c where a,b and c are

View SolutionThe below picture are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.

If a and 1a are the zeroes of the qudratic polynomial 2x2−x+8k then k is
View SolutionThe below pictures are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arc is an arc in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and the architecture in a variety of forms
If α and 1α are the zeroes of the quadratic polynomial 2x2−x+8k then k is
View SolutionThe below pictures are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arc is an arc in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and the architechture in a variety of forms.
If the zeroes of the quadratic polynomial are equal, where the discriminant D=b2−4ac, then

View SolutionThe below picture are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.

In the standard form of quadratic polynomial, ax2+bx+c a,b and c are
View SolutionThe below picture are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.

If the roots of the quadratic polynomial are equal, where the discriminant D=b2−4ac, then
View SolutionForm the quadratic equation, if the sum of the roots is 24 and the product of the roots is 140.
View Solution
