The point A is on the y-axis at a distance of 4 units above from the origin. If the coordinates of the point B are (-3,0). Then length of AB is ____
To find the length of line segment AB, we will follow these steps:
Step 1: Identify the coordinates of points A and B.
Point A is located on the y-axis at a distance of 4 units above the origin, which gives us the coordinates of A as (0, 4). Point B has coordinates (-3, 0).
Step 2: Use the distance formula.
The distance AB between two points A(x1,y1) and B(x2,y2) is given by the formula:
AB=√(x2−x1)2+(y2−y1)2
Substituting the coordinates of points A and B into the formula:
- A(0,4) and B(−3,0)
- x1=0,y1=4,x2=−3,y2=0
Step 3: Calculate the differences in coordinates.
Calculate x2−x1 and y2−y1:
x2−x1=−3−0=−3
y2−y1=0−4=−4
Step 4: Substitute the differences into the distance formula.
Now substitute these values into the distance formula:
AB=√(−3)2+(−4)2
Step 5: Calculate the squares.
Calculate (−3)2 and (−4)2:
(−3)2=9
(−4)2=16
Step 6: Add the squares.
Now add these values:
AB=√9+16=√25
Step 7: Calculate the square root.
Finally, calculate the square root of 25:
AB=5
Thus, the length of AB is 5 units.
---
Point A is located on the y-axis at a distance of 4 units above the origin, which gives us the coordinates of A as (0, 4). Point B has coordinates (-3, 0).
The distance AB between two points A(x1,y1) and B(x2,y2) is given by the formula:
AB=√(x2−x1)2+(y2−y1)2
Substituting the coordinates of points A and B into the formula:
- A(0,4) and B(−3,0)
- x1=0,y1=4,x2=−3,y2=0
Calculate x2−x1 and y2−y1:
x2−x1=−3−0=−3
y2−y1=0−4=−4
Now substitute these values into the distance formula:
AB=√(−3)2+(−4)2
Calculate (−3)2 and (−4)2:
(−3)2=9
(−4)2=16
Now add these values:
AB=√9+16=√25
Finally, calculate the square root of 25:
AB=5
Topper's Solved these Questions
- LINES (IN TWO DIMENSIONS) Book:OSWAL PUBLICATIONChapter:LINES (IN TWO DIMENSIONS) Exercise:CASE - BASED MCQs
Explore 15 Videos
- LINES (IN TWO DIMENSIONS) Book:OSWAL PUBLICATIONChapter:LINES (IN TWO DIMENSIONS) Exercise:CASE - BASED MCQs
Explore 15 Videos
- LINES (IN TWO - DIMENSIONS)Book:OSWAL PUBLICATIONChapter:LINES (IN TWO - DIMENSIONS)Exercise:BOARD CORNER (LONG ANSWER TYPE QUESTION)
Explore 2 Videos
- MAT STAGE 2 (2019-20)Book:OSWAL PUBLICATIONChapter:MAT STAGE 2 (2019-20)Exercise:M.C.Q
Explore 97 Videos
Similar Questions
Knowledge Check
What point on the x-axis are at a distance of 4 units from the line 3x−4y−5=0
A(13,0)B(0,253)C(5,1)D(253,0)The distance of the point p(3,4) from the origin is
A3B4C7D5Distance of point (-3,4) from the origin is ……….
A7B1C5D−5
A point lies on Y-axis at a distance 4 units from X-axis. What are its coordinates ?
View SolutionO is the origin and B is a point on the x-axis at a distance of 2 units from the origin. Match the following lists.
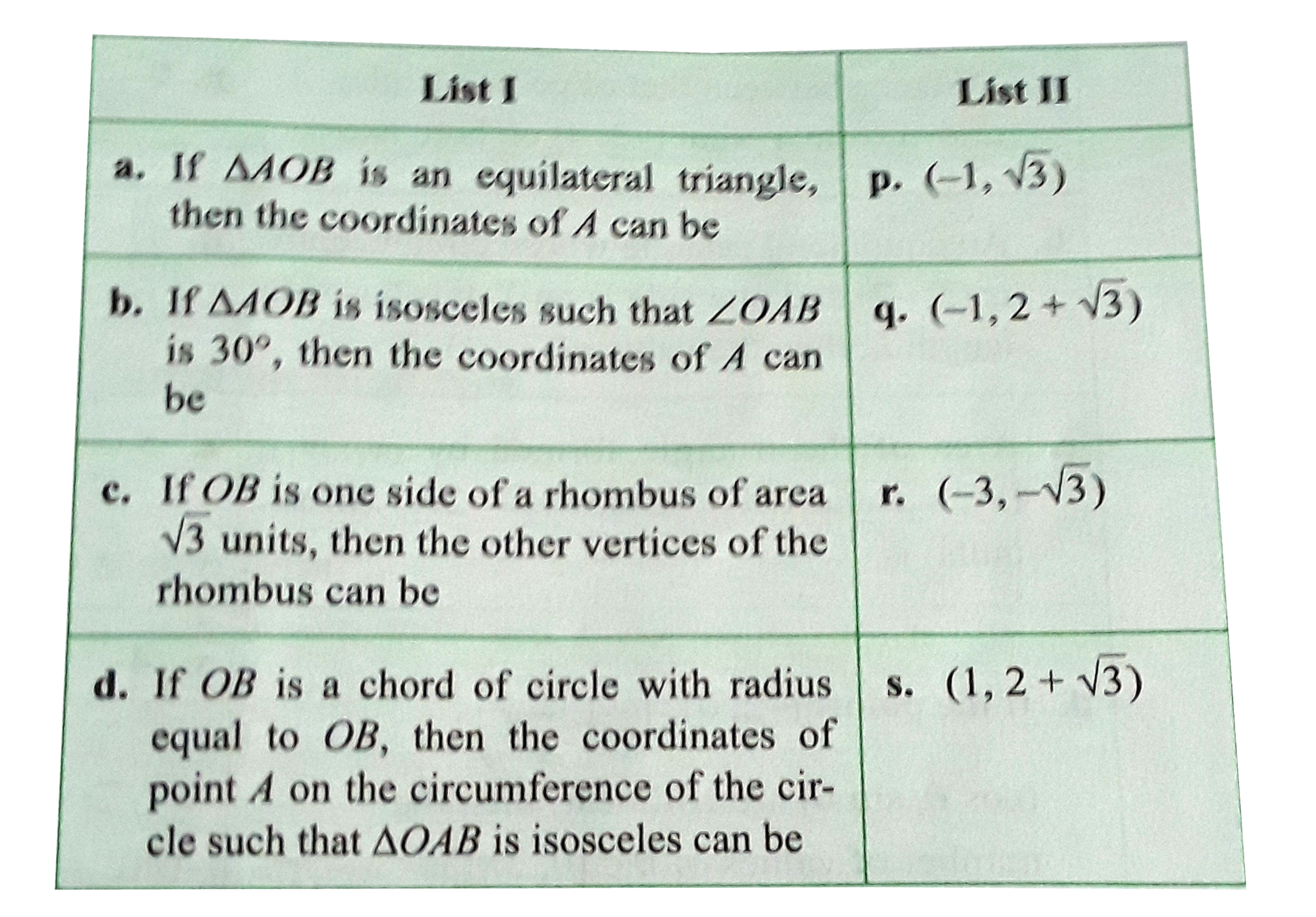
View SolutionFind the distance of the point (4,−3) from the origin .
View SolutionA point lies on X-axis at a distance 5 units from Y-axis. What are its coordinates ?
View SolutionThe points on the Y-axis which are at a distance of 5 units from (4,-1) are _______.
View Solution