Assertion (A): The angle of elevation of the top of the tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘. The height of the tower is 10 m.
Reason (R): The angle of depression from B to A and Angle of elevation from A to B are equal.
Both A and R are true and R is the correct explanation of A.
Both A and R are true and R is not correct explanation of A.
A is true but R is false.
A is false but R is true.
The correct Answer is:D
To solve the given problem, we need to analyze the assertion and the reason provided.
Step 1: Understanding the Assertion
The assertion states that the angle of elevation of the top of the tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘ and that the height of the tower is 10 m.
Step 2: Visualizing the Situation
Let’s denote:
- Point C as the foot of the tower.
- Point B as the top of the tower.
- Point A as the point on the ground 30 m away from C.
We have a right triangle formed by points A, C, and B, where:
- AC = 30 m (distance from the foot of the tower to point A)
- BC = h (height of the tower)
- Angle CAB = 30∘ (angle of elevation)
Step 3: Applying Trigonometry
Using the tangent function for the angle of elevation:
tan(30∘)=BCAC
Substituting the known values:
tan(30∘)=h30
We know that tan(30∘)=1√3, so:
1√3=h30
Step 4: Solving for h
Cross-multiplying gives:
h=30⋅1√3=30√3=10√3 m
Calculating 10√3:
10√3≈10×1.732=17.32 m
Step 5: Conclusion for Assertion
The height of the tower is approximately 17.32 m, which contradicts the assertion that the height is 10 m. Therefore, the assertion is false.
Step 6: Analyzing the Reason
The reason states that the angle of depression from point B to point A and the angle of elevation from point A to point B are equal. This is a true statement in trigonometry because the angle of depression and angle of elevation between two points are equal when measured from a horizontal line.
Final Conclusion
- Assertion (A) is false.
- Reason (R) is true.
The assertion states that the angle of elevation of the top of the tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘ and that the height of the tower is 10 m.
Let’s denote:
- Point C as the foot of the tower.
- Point B as the top of the tower.
- Point A as the point on the ground 30 m away from C.
- AC = 30 m (distance from the foot of the tower to point A)
- BC = h (height of the tower)
- Angle CAB = 30∘ (angle of elevation)
Using the tangent function for the angle of elevation:
tan(30∘)=BCAC
Substituting the known values:
tan(30∘)=h30
We know that tan(30∘)=1√3, so:
1√3=h30
Cross-multiplying gives:
h=30⋅1√3=30√3=10√3 m
Calculating 10√3:
10√3≈10×1.732=17.32 m
The height of the tower is approximately 17.32 m, which contradicts the assertion that the height is 10 m. Therefore, the assertion is false.
The reason states that the angle of depression from point B to point A and the angle of elevation from point A to point B are equal. This is a true statement in trigonometry because the angle of depression and angle of elevation between two points are equal when measured from a horizontal line.
- Assertion (A) is false.
- Reason (R) is true.
Topper's Solved these Questions
- BOOK - OSWAL PUBLICATIONCHAPTER - SOME APPLICATIONS OF TRIGONOMETRY (HEIGHTS AND DISTANCES) EXERCISE - CASE-BASED MCQs15videos
SOME APPLICATIONS OF TRIGONOMETRY (HEIGHTS AND DISTANCES)
- BOOK - OSWAL PUBLICATIONCHAPTER - SOME APPLICATIONS OF TRIGONOMETRY (HEIGHTS AND DISTANCES) EXERCISE - CASE-BASED MCQs15videos
SOME APPLICATIONS OF TRIGONOMETRY (HEIGHTS AND DISTANCES)
- BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE QUESTION PAPEREXERCISE - Section -C5videos
SAMPLE QUESTION PAPER
- BOOK - OSWAL PUBLICATIONCHAPTER - STATISTICSEXERCISE - Assertion and Reasoning Based Questions2videos
STATISTICS
Similar Questions
The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘. Find the height of the tower.
View Solution
Knowledge Check
- Question 1 - Select One
The angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower , is 30∘ . Then , the height ( in m) of the tower is .
A10√3B30√3C10√3D10
The angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.
 View Solution
View Solution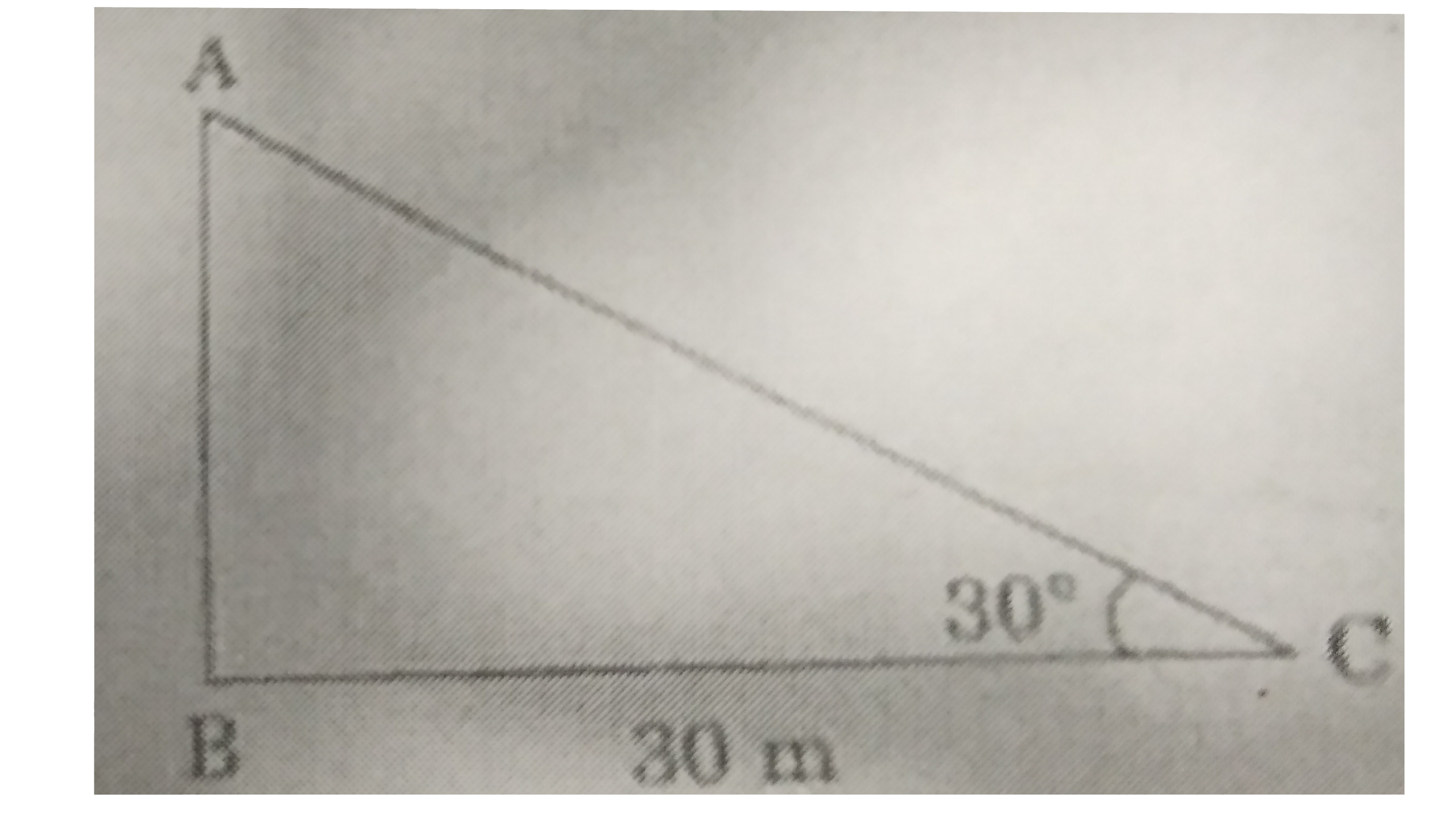
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.View SolutionIf the angle of elevation of top of a tower from a point on the ground which is 20√3 m away from the foot of the tower is 30∘, then find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 25 m away from the foot of between the ship and tower.
View Solution

