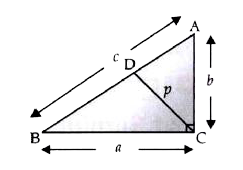
ABC is a triangle right angled at C with BC = a and AC = b. If p is the length of the perpendicular from C on AB, then which one of the following is correct ?

$a^{2} b^{2} = p^{2} (a^{2} + b^{2})$

$a^{2} b^{2} = p^{2} (b^{2} - a^{2})$

$2 a^{2} b^{2} = p^{2} (a^{2} + b^{2})$

$a^{2} b^{2} = 2 p^{2} (a^{2} + b^{2})$

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem, we need to find the relationship between the lengths of the sides of the triangle ABC, which is right-angled at C, and the length of the perpendicular from C to the hypotenuse AB.
1. Identify the Triangle:
- We have a right triangle ABC with the right angle at C.
- Let BC = a and AC = b.
2. Use the Pythagorean Theorem:
- According to the Pythagorean theorem, the length of the hypotenuse AB can be calculated as:
$A B = \sqrt{B C^{2} + A C^{2}} = \sqrt{a^{2} + b^{2}}$
3. Define the Perpendicular:
- Let $p$ be the length of the perpendicular from point C to line AB.
4. Use the Area of the Triangle:
- The area of triangle ABC can be calculated in two ways:
- Using the base and height (where BC is the base and AC is the height):
$Area = \frac{1}{2} \times B C \times A C = \frac{1}{2} \times a \times b$
- Using the hypotenuse AB and the height p:
$Area = \frac{1}{2} \times A B \times p = \frac{1}{2} \times \sqrt{a^{2} + b^{2}} \times p$
5. Set the Areas Equal:
- Since both expressions represent the area of the same triangle, we can set them equal to each other:
$\frac{1}{2} \times a \times b = \frac{1}{2} \times \sqrt{a^{2} + b^{2}} \times p$
6. Simplify the Equation:
- Cancel the $\frac{1}{2}$ from both sides:
$a \times b = \sqrt{a^{2} + b^{2}} \times p$
7. Solve for p:
- Rearranging gives us:
$p = \frac{a \times b}{\sqrt{a^{2} + b^{2}}}$
8. Square Both Sides:
- To eliminate the square root, we can square both sides:
$p^{2} = \frac{a^{2} \times b^{2}}{a^{2} + b^{2}}$
Final Result:
The relationship derived from the above steps is:
$p^{2} = \frac{a^{2} \times b^{2}}{a^{2} + b^{2}}$

Updated on:8/8/2024