If x varies as yz, then y varies inversely as
xz
xz
zx
1(xz)
To solve the problem, we start with the given information and follow the steps logically.
1. Understanding the Variation:
We are given that x varies as yz. This can be expressed mathematically as:
x=k⋅yz
where k is a constant of proportionality.
Hint: Remember that "varies as" means there is a direct proportionality.
2. Expressing y in terms of x and z:
From the equation x=k⋅yz, we can rearrange it to express y:
y=xkz
Hint: When you want to isolate a variable, you can rearrange the equation by dividing or multiplying both sides.
3. Identifying Inverse Variation:
We need to find how y varies inversely. If y varies inversely with respect to some variable, it can be expressed as:
y∝1(some variable)
In our case, since y is expressed in terms of x and z, we can say:
y∝1zandy∝1x
Hint: Inverse variation means that as one variable increases, the other decreases.
4. Combining the Relationships:
Since y varies inversely with both z and x, we can express this as:
1y∝xz
This indicates that y varies inversely as the product xz.
Hint: When combining inverse relationships, think about how they relate to each other multiplicatively.
5. Final Expression:
Thus, we conclude that y varies inversely as xz. In terms of the original variables, we can summarize:
y varies inversely as xz
Hint: Always check if your final expression matches the form of inverse variation.
Conclusion:
The answer is that y varies inversely as xz.
We are given that x varies as yz. This can be expressed mathematically as:
x=k⋅yz
where k is a constant of proportionality.
From the equation x=k⋅yz, we can rearrange it to express y:
y=xkz
We need to find how y varies inversely. If y varies inversely with respect to some variable, it can be expressed as:
y∝1(some variable)
In our case, since y is expressed in terms of x and z, we can say:
y∝1zandy∝1x
Since y varies inversely with both z and x, we can express this as:
1y∝xz
This indicates that y varies inversely as the product xz.
Thus, we conclude that y varies inversely as xz. In terms of the original variables, we can summarize:
y varies inversely as xz
The answer is that y varies inversely as xz.
Similar Questions
Knowledge Check
If x varies as mth power of y, y varies as nth power of z and x varies as pth power of z then which one of the following is true?
Ap=m+nBp=m−nCp=mnDNone of theseThe number of α-particless scattered per unit area N (θ) at scattering angle θ varies inversely as
Acos4(θ2)Bsin4(θ2)Ctan4(θ2)Dcot4(θ2)Assertion : Mean free path of a gas molecule varies inversely as density of the gas.
Reason : Mean free path varies inversely as pressure of the gas.AIf both assertion and reason are true and the reason is correct explanation of the assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true, but the reason is false.DIf assertion is false but the reason is true
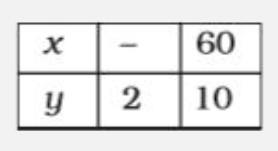
If x varies inversely as y, then
View SolutionIf the area of circle increases at a uniform rate, then prove that the perimeter varies inversely as the radius.
View SolutionVolume (v) of a gas of given mass, varies inversely as the pressure (p), then fill up the blanks
View SolutionIf x varies as y, then which of the following is/are correct?
1. x2+y2 varies as x2−y2
2. xy2 varies inversely as y
3. n√x2y varies as 2n√x4y2
Select the correct answer using the code given below :
View SolutionInside a hollow charged spherical conductor the potential Is constant Varies directly as the distance from the centre Varies inversely as the distance from the centre Varies inversely as the square of the distance from the centre
View Solution
