A right circular cylinder has height as 18 cm and radius as 7 cm. The cylinder is cut in three equal parts (by 2 cuts parallel to base). What is the percentage increase in total surface area?
62
56
48
52
The correct Answer is:B
To solve the problem step by step, we will calculate the total surface area of the cylinder before and after it is cut into three equal parts. Then, we will find the percentage increase in the total surface area.
Step 1: Calculate the Total Surface Area of the Cylinder Before Cutting
The formula for the total surface area (TSA) of a right circular cylinder is given by:
TSA=2πrh+2πr2
Where:
- r is the radius,
- h is the height.
Given:
- Height h=18 cm,
- Radius r=7 cm.
Substituting the values into the formula:
TSA=2π(7)(18)+2π(72)
Calculating each part:
1. Calculate 2πrh:
2π(7)(18)=252π
2. Calculate 2πr2:
2π(72)=98π
Now, add both parts together:
TSA=252π+98π=350π
Using π≈227:
TSA=350×227=1100 cm2
Step 2: Calculate the Total Surface Area After Cutting
When the cylinder is cut into three equal parts, we make two cuts. Each cut introduces a new circular surface area.
- Each circular base has an area of πr2.
- After two cuts, there will be 4 circular surfaces (2 bases from the original cylinder and 2 new bases from the cuts).
The area contributed by the bases after cutting is:
Area from bases=4πr2
Substituting r=7:
Area from bases=4π(72)=4π(49)=196π
Now, the total surface area after cutting is:
New TSA=Original TSA+Area from bases
New TSA=350π+196π=546π
Using π≈227:
New TSA=546×227=1716 cm2
Step 3: Calculate the Percentage Increase in Total Surface Area
The percentage increase in total surface area is calculated as follows:
Percentage Increase=(New TSA−Original TSAOriginal TSA)×100
Substituting the values:
Percentage Increase=(1716−11001100)×100
=(6161100)×100
=56%
Final Answer:
The percentage increase in total surface area after cutting the cylinder into three equal parts is 56%.
- r is the radius,
- h is the height.
- Height h=18 cm,
- Radius r=7 cm.
2π(7)(18)=252π
2π(72)=98π
- After two cuts, there will be 4 circular surfaces (2 bases from the original cylinder and 2 new bases from the cuts).
New TSA=350π+196π=546π
=(6161100)×100
=56%
The percentage increase in total surface area after cutting the cylinder into three equal parts is 56%.
Similar Questions
A right circular cylinder has height as 18cm and radius as 7cm. The cylinder is cut in three equal parts (by 2 cuts parallel to base). What is the percentage increase in total surface area?
एक लम्बवत गोलकार बेलन की लम्बाई 18 से.मी. तथा त्रिज्या 7 से.मी. हैं बेलन को तीन बराबर भागों में काटा जाता हैं (आधार के समांतर 2 कटाव द्वारा) कुल पृष्ठाय क्षेत्रफल में कितने प्रतिशत की वृद्धि हुई है?
View SolutionA right circular cylinder has height 28cm and radius of base 14cm. Two hemispheres of radius 7 cm each are cut from each of the two bases of the cylinder. What is the total surface area (in cm2) of the remaining part?
View Solution
Knowledge Check
A solid cylinder having radius of base as 7 cm. and length as 20 cm is bisected from its height to get two identical cylinders. What will be the percentage increase in the total surface area ?
A29.78B25.93C27.62D32.83A solid cylinder having radius of base as 7 cm. and length as 20 cm is bisected from its height to get two identical cylinders. What will be the percentage increase in the total surface area ?
A(a) 29.78B(b) 25.93C(c) 27.62D(d) 32.83A right circular solid cylinder has radius of base 7 cm and height is 28 cm. It is melted to form a cuboid such that the ratio of its side is 2 : 3 : 6. What is the total surface area (incm2) cuboid ?
A72x(1078)23B36x(2078)23C72x(2078)23D36x(1078)23
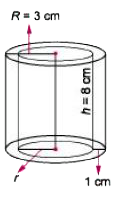
A hollow cylinder made of wood has thickness 1 cm while its external radius is 3 cm. If the height of the cylinder is 8 cm, then find the volume, curved surface area and total surface area of the cylinder.
View SolutionIf the height of cylinder is increased by 35% and radius is increased by 10%, then what will be the percentage increase in curved surface area of cylinder?
View SolutionThe total surface area of a right circular cylinder whose height is 15 cm and the radius of the base is 7 cm, is
View SolutionThe total surface area of a right circular cylinder whose height is 15 cm and the radius of the base is 7 cm, is
View SolutionA right circular cone and a right circular cylinder have equal base and equal height. If the radius of the base and the hight are in the ratio 5:12, then the ratio of the total surface area of the cylinder to that of the cone is
View Solution
