Which of the following graphs shows the variation of de-Broglie wavelength with potential through which a particle of charge q and mass m is accelerated ?


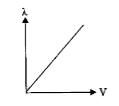
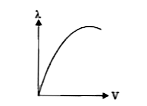
The correct Answer is:B
To solve the problem of determining the variation of de-Broglie wavelength (λ) with the potential (V) through which a charged particle (with charge q and mass m) is accelerated, we can follow these steps:
Step 1: Understand the relationship between kinetic energy and potential
When a particle with charge q is accelerated through a potential difference V, its kinetic energy (KE) can be expressed as:
KE=qV
Step 2: Relate kinetic energy to momentum
The kinetic energy can also be expressed in terms of momentum (p):
KE=p22m
Equating the two expressions for kinetic energy gives:
qV=p22m
Step 3: Solve for momentum
Rearranging the equation to find momentum p:
p2=2mqV
p=√2mqV
Step 4: Use the de-Broglie wavelength formula
The de-Broglie wavelength (λ) is given by:
λ=hp
Substituting the expression for momentum we derived:
λ=h√2mqV
Step 5: Analyze the relationship between λ and V
From the equation λ=h√2mqV, we can see that:
λ∝1√V
This indicates that as the potential V increases, the de-Broglie wavelength λ decreases.
Step 6: Determine the nature of the graph
Since λ is inversely proportional to the square root of V, we can conclude that the graph of λ versus V will not be a straight line. Instead, it will be a curve that decreases as V increases.
Step 7: Identify the correct graph
From the analysis, we can infer that:
- As V approaches 0, λ approaches infinity.
- As V increases, λ decreases towards 0.
This behavior corresponds to a graph that starts high (when V is low) and decreases as V increases, which is characteristic of a hyperbolic or inverse relationship.
Conclusion
Based on the analysis, the correct graph that shows the variation of de-Broglie wavelength with potential is Option 2.
---
When a particle with charge q is accelerated through a potential difference V, its kinetic energy (KE) can be expressed as:
KE=qV
The kinetic energy can also be expressed in terms of momentum (p):
KE=p22m
Equating the two expressions for kinetic energy gives:
qV=p22m
Rearranging the equation to find momentum p:
p2=2mqV
p=√2mqV
The de-Broglie wavelength (λ) is given by:
λ=hp
Substituting the expression for momentum we derived:
λ=h√2mqV
From the equation λ=h√2mqV, we can see that:
λ∝1√V
This indicates that as the potential V increases, the de-Broglie wavelength λ decreases.
Since λ is inversely proportional to the square root of V, we can conclude that the graph of λ versus V will not be a straight line. Instead, it will be a curve that decreases as V increases.
From the analysis, we can infer that:
- As V approaches 0, λ approaches infinity.
- As V increases, λ decreases towards 0.
Based on the analysis, the correct graph that shows the variation of de-Broglie wavelength with potential is Option 2.
Similar Questions
Show graphically, the variation of the de-Broglie wavelength (λ) with the potential (V) through which an electron is accelerated from rest.
View SolutionShow graphically, the variation of the de-Broglie wavelength (λ) with the potential (V) through which an electron is accelerated from rest.
View Solution
Knowledge Check
The de-Broglie wavelength of a particle of charge q and mass m is λ. If its kinetic energy be K, then
Aλ=h√mKBλ=h√mqKCλ=√2mKhDλ=√mqKh.The de-Broglie wavelength λ associated with charged particle of charge q, mass m and potential difference V is
Ah√2mqVBh2√2mqVCh√mqVDh√2qV
Draw a graph showing variation of de-Broglie wavelength with the momentum of an electron.
View Solution(a) Draw a graph showing the variation of de-Broglie wavelength of a particle of charge 'q' and mass 'm' with accelerating potential 'V'.
(b) Proton and deuteron have the same de-Broglie wavelength. Explain which has more kinetic enegy.
View SolutionDraw a graph showing the variation of de Broglie wavelength of a particle of charge q and mass m with accelerating potential. Proton and deuteron have the same de Broglie wavelengths. Explain which has more kinetic energy.
View SolutionWrite the expression for the de-Broglie wavelength associated with a charge particle having charge 'q' and mass 'm', when it is accelerated by a potential V.
View SolutionWrite the expression for the de-Broglie wavelength associated with a charged particle having charge 'q' and mass 'm' when it is accelerated by a potential V.
View SolutionWhich of following graphs correctly represents the variation of particle momentum with de-Broglie wavelength?
View Solution
