Assertion (A): The binding energy per nucleon, for nuclei with mass number (A)>56 decreases with A.
Reason (R): Nuclear force is weak in heavier nuclei.
Both A and R are true and R is the correct explanation of A
Both A and R are true but R is NOT the correct explanation of A
A is true but R is false
A is false and R is true
The correct Answer is:C
To analyze the assertion and reason provided in the question, we will break down the concepts of binding energy per nucleon and nuclear forces step by step.
Step 1: Understanding Binding Energy per Nucleon
The binding energy per nucleon is defined as the energy required to disassemble a nucleus into its constituent nucleons (protons and neutrons) divided by the total number of nucleons. It is a measure of the stability of a nucleus.
Step 2: Behavior of Binding Energy with Mass Number
For nuclei with mass number A less than or equal to 56, the binding energy per nucleon generally increases with increasing A. This is due to the strong nuclear force, which binds nucleons together more effectively as more nucleons are added up to a certain point.
Step 3: Binding Energy for Mass Number Greater than 56
However, for nuclei with mass number A greater than 56, the binding energy per nucleon tends to decrease. This is because, in heavier nuclei, the repulsive forces between protons (due to electrostatic repulsion) start to dominate over the attractive nuclear forces. As a result, the stability of the nucleus decreases, leading to a lower binding energy per nucleon.
Step 4: Evaluating the Assertion
The assertion states that "the binding energy per nucleon, for nuclei with mass number A>56, decreases with A." This statement is true based on the explanation above.
Step 5: Understanding Nuclear Forces in Heavier Nuclei
The reason provided states that "nuclear force is weak in heavier nuclei." While it is true that the effective binding in heavier nuclei is less efficient due to the increasing repulsive forces, it is not entirely accurate to say that the nuclear force itself is weak; rather, it is the balance between attractive and repulsive forces that changes.
Step 6: Evaluating the Reason
The reason given is somewhat misleading. While the repulsive forces do increase in heavier nuclei, the nuclear force itself does not become weak; it is the overall effect of the forces that leads to a decrease in binding energy per nucleon. Therefore, the reason is not entirely correct.
Conclusion
- Assertion (A) is True.
- Reason (R) is False.
Final Answer:
- Assertion (A): True
- Reason (R): False
---
The binding energy per nucleon is defined as the energy required to disassemble a nucleus into its constituent nucleons (protons and neutrons) divided by the total number of nucleons. It is a measure of the stability of a nucleus.
For nuclei with mass number A less than or equal to 56, the binding energy per nucleon generally increases with increasing A. This is due to the strong nuclear force, which binds nucleons together more effectively as more nucleons are added up to a certain point.
However, for nuclei with mass number A greater than 56, the binding energy per nucleon tends to decrease. This is because, in heavier nuclei, the repulsive forces between protons (due to electrostatic repulsion) start to dominate over the attractive nuclear forces. As a result, the stability of the nucleus decreases, leading to a lower binding energy per nucleon.
The assertion states that "the binding energy per nucleon, for nuclei with mass number A>56, decreases with A." This statement is true based on the explanation above.
The reason provided states that "nuclear force is weak in heavier nuclei." While it is true that the effective binding in heavier nuclei is less efficient due to the increasing repulsive forces, it is not entirely accurate to say that the nuclear force itself is weak; rather, it is the balance between attractive and repulsive forces that changes.
The reason given is somewhat misleading. While the repulsive forces do increase in heavier nuclei, the nuclear force itself does not become weak; it is the overall effect of the forces that leads to a decrease in binding energy per nucleon. Therefore, the reason is not entirely correct.
- Assertion (A) is True.
- Reason (R) is False.
- Assertion (A): True
- Reason (R): False
Similar Questions
The dependence of binding energy per nucleon, BN on the mass number, A, is represented by
View SolutionDraw the graph showing thervariation of binding energy per nucleon with mass number. Give therason for the decrease of binding energy per nucleon for nuclei with high mass numbers.
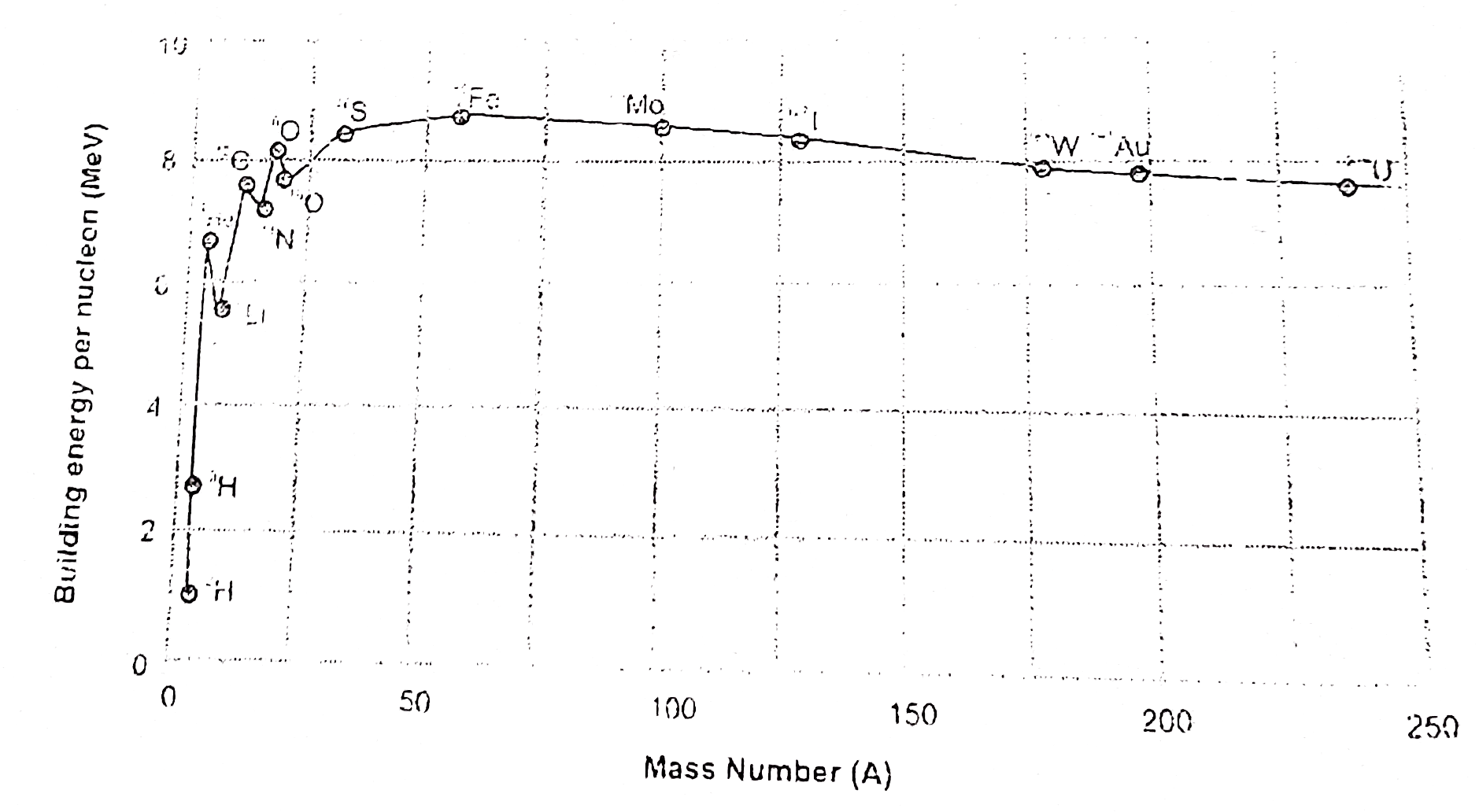
The binding energy per nucleon for nuclei with high mass number is low due to the large coulomb repulsion between the protons inside these nuclei.View Solution
Knowledge Check
- Question 1 - Select One
The binding energy per nucleon, for nuclei with atomic mass number A>100, decreases with A.
The nuclear forces are weak for heavier nuclei.AIf both assertion and reason are true and the reason is the correct explanation of the assertionBIf both assertion and reason are true but reason is not the correct explanantion of the assertionCIf assertion is true but reason is falseDIf the assertion and reason both are false - Question 2 - Select One
Binding energy per nucleon relation with mass number
Afirst decreases then increasesBfirst increases then decreasesCincreasesDdecreases - Question 3 - Select One
The binding energy per nucleon, for nuclei with atomic mass number A>100, decreases with A.
The nuclear forces are weak for heavier nuclei.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is falseDIf assertion is false but reason is true.
Variation Of Binding Energy Of Per Nucleon With Mass Number
View SolutionThe graph between the binding energy per nucleon (E) and atomic mass number (A) is as-
View SolutionFor atomic nuclei, the binding energy per nucleon
View SolutionMaximum value of binding energy per nucleon for most stable nuclei is
View SolutionAs the mass number increase binding energy per nucleon :
View Solution

