A man standing under a street lamp of height ‘H’ above the ground starts running with a constant speed ‘v’ in a constant direction. The light from the lamp falling on the man form a shadow of him. Find the velocity with which the edge of the shadow of the man’s head moves over the ground if his height is “h”.

Text Solution

Verified by Experts

Here we need to compare the motions of the man and the edge of the shadow of the man’s head. To solve this problem, a common starting point is necessary. Let this point be ‘O’ which is taken exactly under the lamp as shown in the fig-13.
Let ‘s’ and ‘S’ be the distances covered in a time interval ‘t’ from the point ‘O’ by the man and the edge of the shadow of the man’s head respectively.

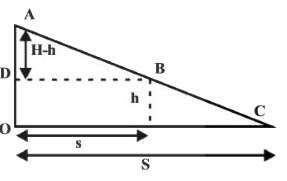
As shown in figure we get two similar triangles namely $Δ A B D$ and $Δ A C O$ From these two similar triangles
$\frac{D B}{A D} = \frac{O C}{A O}$
$\frac{s}{H - h} = \frac{S}{H}$
But we know, s=vt
$\frac{v t}{H - h} = \frac{S}{H}$
We know that S/t is the speed of the shadow of the man’s head,
Then,
$V = \frac{H v}{H - h}$

A man of height h walks in a straight path towards a lamp post of height H with uniform velocity u. Then , the velocity of the edge of the shadow on the ground will be
A $\frac{h u}{H - h}$
B $\frac{H u}{H + h}$
C $\frac{H - h}{H u}$
D $\frac{H + h}{H u}$
A man of height h walks in a straight path towards a lamp post of height H with uniform velocity u. Then , the velocity of the edge of the shadow on the ground will be
A $\frac{h u}{H - h}$
B $\frac{H u}{H + h}$
C $\frac{H - h}{H u}$
D $\frac{H + h}{H u}$
man of height h walks in a straight path towards a lamp post of height H with uniform velocity u. Then the velocity of the edge of the shadow on the ground will be :-
A $\frac{H u}{(H - h)}$
B $\frac{H u}{(H + h)}$
C $\frac{(H - h)}{H u}$
D $\frac{(H + h)}{H u}$