Question

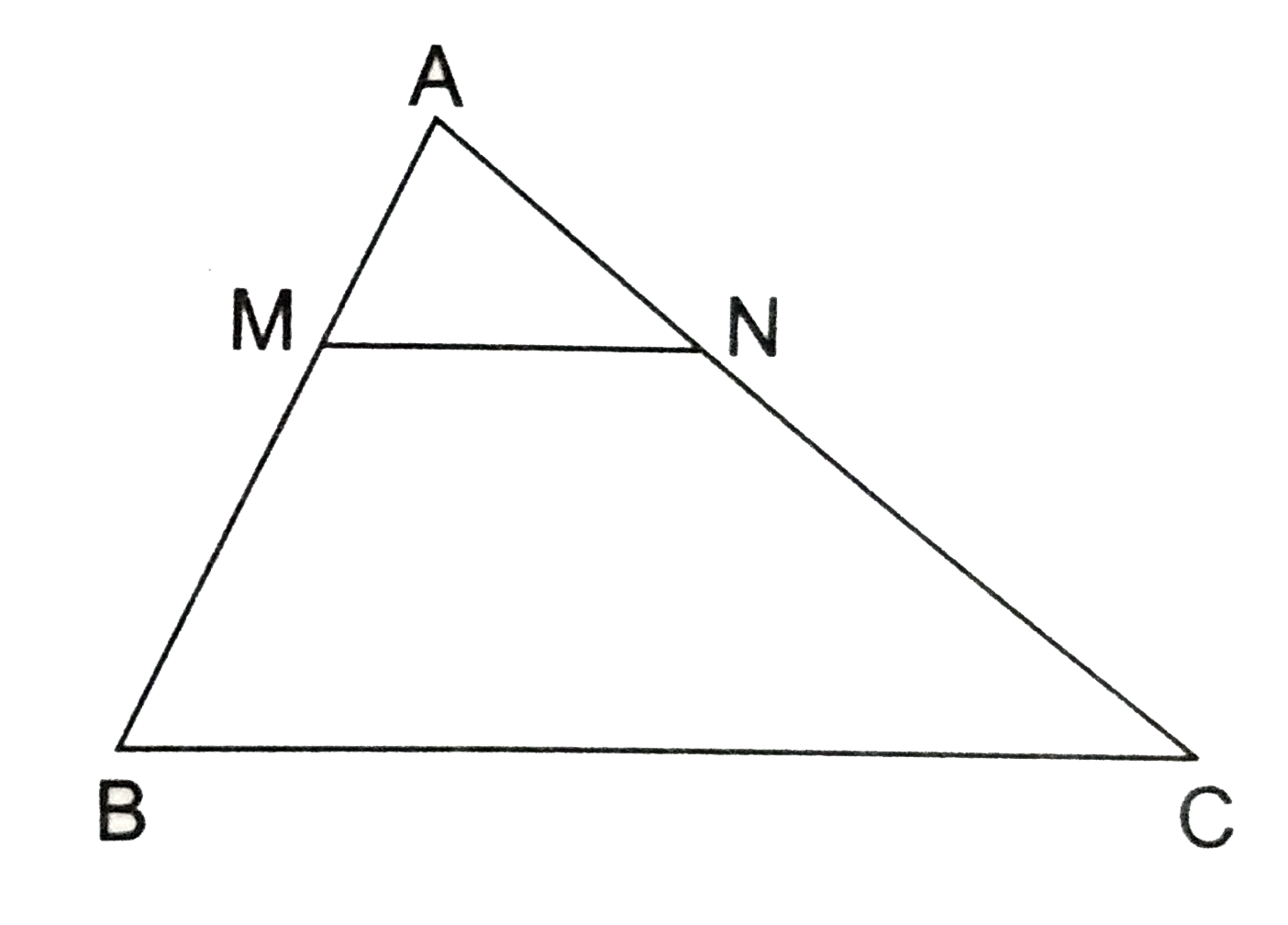
In the $\Delta ABC$ , MN||BC and AM : : MB = $\frac{1}{3}$.
Then, $\frac{ar(\Delta AMN)}{ar(\Delta ABC)}$=?

Options

A. `9:1`
B. `1:16`
C. `2:3`
D. `3:2`

Answer

B

Explanation

To solve the problem, we need to find the ratio of the areas of triangles AMN and ABC given that MN is parallel to BC and the ratio AM:MB = 1:3.

1. Understanding the Given Information:
- We have triangle ABC.
- MN is a line parallel to BC.
- The ratio of segments AM to MB is given as 1:3.

2. Using the Property of Similar Triangles:
- Since MN is parallel to BC, triangles AMN and ABC are similar by the Basic Proportionality Theorem (or Thales' theorem).
- This means that the corresponding angles of triangles AMN and ABC are equal.

3. Setting Up the Ratios:
- From the given ratio AM:MB = 1:3, we can express the lengths of AM and MB in terms of a variable. Let AM = x and MB = 3x.
- Therefore, the total length AB = AM + MB = x + 3x = 4x.

4. Finding the Ratio of Corresponding Sides:
- The ratio of the corresponding sides of triangles AMN and ABC is:
\( \frac{AM}{AB} = \frac{x}{4x} = \frac{1}{4} \)

5. Calculating the Ratio of Areas:
- The ratio of the areas of similar triangles is equal to the square of the ratio of their corresponding sides. Therefore, we have:
\( \frac{ar(ΔAMN)}{ar(ΔABC)} = \left(\frac{AM}{AB}\right)^2 = \left(\frac{1}{4}\right)^2 = \frac{1}{16} \)

6. Final Answer:
- Thus, the ratio of the area of triangle AMN to the area of triangle ABC is:
\( \frac{ar(ΔAMN)}{ar(ΔABC)} = \frac{1}{16} \)