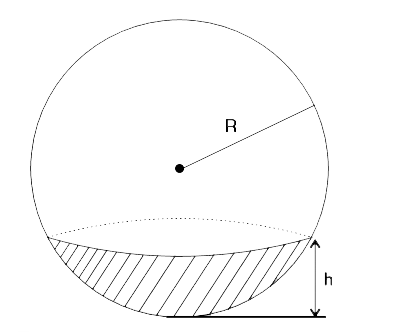
The volume of a spherical cap of height h cut off from a sphere of radius a is equal to :
π3h2(3a−h)
π(a−h)(2a2−h2−ah)
4π3h2
none of the above
The correct Answer is:A
Similar Questions
Show that the volume of a segment of height h of a sphere of radius R is
V=13πh2(3R−h)
View SolutionThe radius of a sphere is equal to the radius of the base of a right circular cone, and the volume of the sphere is double the volume of the cone. The ratio of the height of the cone to the radius of its base is
View Solution
Knowledge Check
The volumes of a sphere and a right circular cylinder havIng the same radius are equal , The ratio of the diameter of the sphere to the height of the cylinder is
A3:2B2:3C1:2D2:1The volumes of a sphere and a right circular cylinder having the same radius are equal. The ratio of the diameter of the sphere to the height of the cylinder is
A1:2B2:1C2:3D3:2Volume of a sphere is equal to the volume of a hemisphere. If the radius of the hemisphere is 3√32 cm, then the radius of the sphere is equal to
Aa) 93√2 cmBb) 63√2 cmCc) 3√16 cmDd) 3√64 cm
The volume of a cone is equal to that of sphere. If the diameter of base of cone is equal to the diameter of the sphere, what is the ratio of height of cone to the diameter of the sphere ?
View SolutionA sphere of maximum volume is cut out from a solid hemisphere of radius r . The ratio of the volume of the hemisphere to that of the cut out sphere is: (a) 3 : 2 (b) 4 : 1 (c) 4 : 3 (d) 7 : 4
View SolutionIf the height of a cone is half the radius of a sphere then the radius of the base of the cone, which has the same volume as a sphere of radius 7 cm is :
View SolutionIf the volume of a sphere is numerrically equal to its surface area. Then radius of sphere is
View SolutionIf the height of a cone is half the radius of a sphere then the radius of the base of the cone, which has the same volume as a sphere of radius 7 cm is :
View Solution
