If A={(x,y):x2+y2≤1:x,y∈R} and B={(x,y):x2+y2≥4,x,y∈R}, then
A−B=ϕ
B−A=ϕ
A∩B≠ϕ
A∩B=ϕ
The correct Answer is:D
Similar Questions
Knowledge Check
ifA={(x,y):x2+y2≤1,x,y∈R]and
B={(x,y):x2+y2≤x2+y2≤4,x,y∈R]thenAA−B=ABB−A=BCA−B=ϕDB−A=ϕifA={(x,y):x2+y2=4,x,y∈R}and
B={(x,y):Y=|x|,x,y∈R} thenAA∩B=ϕBA∩B is singlcton setCA∩B contains two elements onlyDA∩B contains three elements only .If R = {(x,y) : x,y ∈ Z , x2+y2≤4 } is a relation, then domain of R is
A{0,1,2}B{0,-1,-2}C{-2,-1,0,1,2}DNone of the above
In some question of sets, we have to make the use of graphs
For example
A={(x,y):y=ex,x∈R}
B={{x,y}:y=−x.x∈R}
Find n(A∩B)
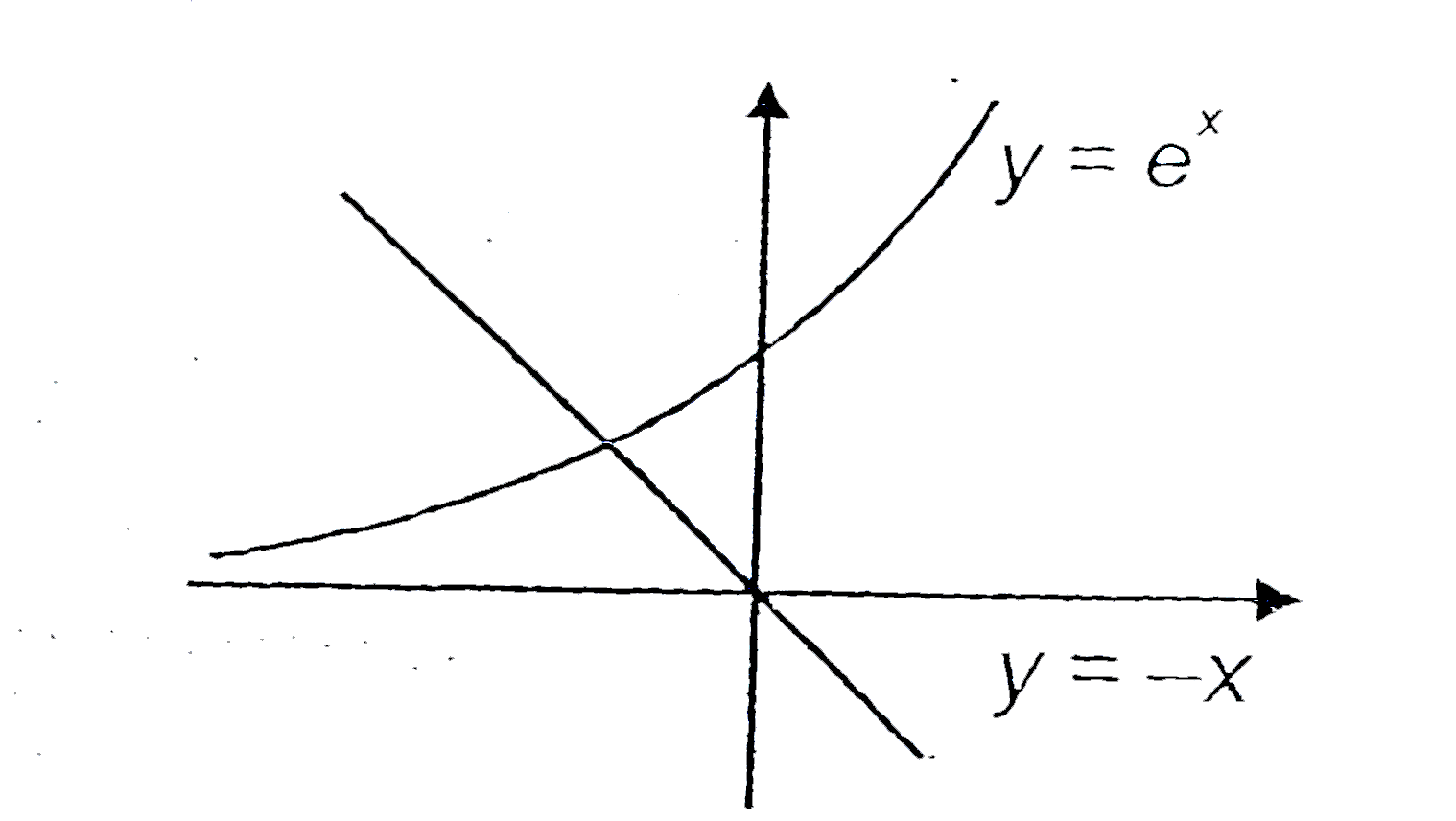
It is clear that y=exandy=−x intersect at one pont. Hence n(A∩B)=1
A={(x,y):x2+y2≤2,x,y∈R}
B={{x,y):y≥x2,x,y∈R}
Then domain of A∩B is
View SolutionIn some question of sets, we have to make the use of graphs
For example
A={(x,y):y=ex,x∈R}
B={{x,y}:y=−x.x∈R}
Find n(A∩B)

It is clear that y=exandy=−x intersect at one pont. Hence n(A∩B)=1
A={(x,y):x2+y2≤2,x,y∈R}
B={{x,y):y≥x2,x,y∈R}
View SolutionIf A={(x,y):y=ex,x∈R} and B={(x,y):y=e−2x,x∈R}, then
View SolutionCalculate the area enclosed in the region
(i) {(x,y):x2+y2≤1≤x+y}
(ii) {(x,y):x2+y2≤4x,4x2+4y2≤9}
(iii) {(x,y):x2+y2≤16,x2≤6y}
(iv) {(x,y):y2≤6ax,x2+y2≤16a2}
(v) {(x,y):x2+y2≤8,x2≤2y}.
View SolutionWhich one of the following is not a function? {(x,y):x,y∈R,x2=y} b. {(x,y):x,y∈R,y2=x} c. {(x,y):x,y∈R,x=y3} d. {(x,y):x,y∈R,y=x3}
View Solution
