The shortest height of a vertical mirror required to see the entire image of a man, will be:

A

one-third the man's height

B

half the man's height

C

two-third the man's height

D

data insufficient

Text Solution
Verified by Experts

The correct Answer is:B

Let MN = height of eye
E = position of eye
As , ∠NGQandΔQGE are similar.
ΔESPandΔPSM are cogruent.
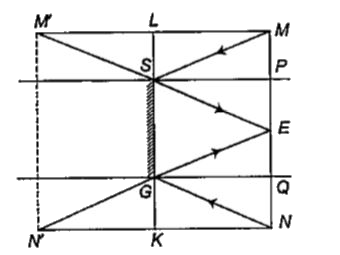
ocr_image
MP = PE = EQ = QN
Now, SG=PQ=PE+EQ
SG=ME2+EN2
SG=12MN

Updated on:21/07/2023
Doubtnut Promotions Banner
|
Class 12PHYSICSGEOMETRICAL OPTICS

Similar Questions

Knowledge Check

  • Assertion : When the object moves with a velocity →v , its image in the plane mirror moves with a velocity of −5→v. With respect to the object ,
    Reason : the minimum height of the mirror to be required to see the full image of man of height h is h3

    AIf both Assertion & Reason are True & Reason is a correct explanation of the Assertion.
    BIf both Assertion & Reason are True but Reason is not a correct explanation of the Assertion.
    CIf Assertion is True but the Reason is False.
    DIf both Assertion & Reason are False.

  • The minimum height of a plane mirror to see the full size image of a person is equal to

    Athe height of the person
    Bhalf the height of the person
    Cone-fourth the height of the person
    Ddouble the height of the person

  • A man holds a rectangular card in front of and parallel to a plane mirror. In order for him to see the entire image of the card, the least mirror area needed is

    Athat of the whole mirror, regardless of its size
    Bthat of the pupil of his eye
    Cone-half that of the card
    Done-fourth that of the card