Find the minimum height of a mirror where one can see his full image.
The correct Answer is:B
To find the minimum height of a mirror required for a person to see their full image, we can follow these steps:
1. Understand the Concept of Reflection:
When light reflects off a mirror, the angle of incidence equals the angle of reflection. This means that the light from the top of the object (the person) must reach the mirror and then reflect back to the eyes of the observer.
2. Identify the Height of the Object:
Let’s denote the height of the person (the object) as h.
3. Determine the Required Height of the Mirror:
For a person to see their full image in the mirror, the mirror must be positioned such that it reflects light from both the top of the person's head and their feet back to their eyes.
4. Calculate the Minimum Height of the Mirror:
The minimum height of the mirror required can be derived from the geometry of the situation. The distance from the eyes to the top of the head and the feet must be equal when reflected. Therefore, the minimum height of the mirror hm is given by:
hm=h2
This means that the mirror needs to be at least half the height of the person.
5. Example Calculation:
If the height of the person is 6 meters, then the minimum height of the mirror would be:
hm=62=3 meters
Final Answer:
The minimum height of the mirror required for a person to see their full image is half of their height.
When light reflects off a mirror, the angle of incidence equals the angle of reflection. This means that the light from the top of the object (the person) must reach the mirror and then reflect back to the eyes of the observer.
Let’s denote the height of the person (the object) as h.
For a person to see their full image in the mirror, the mirror must be positioned such that it reflects light from both the top of the person's head and their feet back to their eyes.
The minimum height of the mirror required can be derived from the geometry of the situation. The distance from the eyes to the top of the head and the feet must be equal when reflected. Therefore, the minimum height of the mirror hm is given by:
hm=h2
This means that the mirror needs to be at least half the height of the person.
If the height of the person is 6 meters, then the minimum height of the mirror would be:
hm=62=3 meters
The minimum height of the mirror required for a person to see their full image is half of their height.
Similar Questions
Show that the minimum size of a plane mirror required to see the full image of an observer is half the size of that observer.
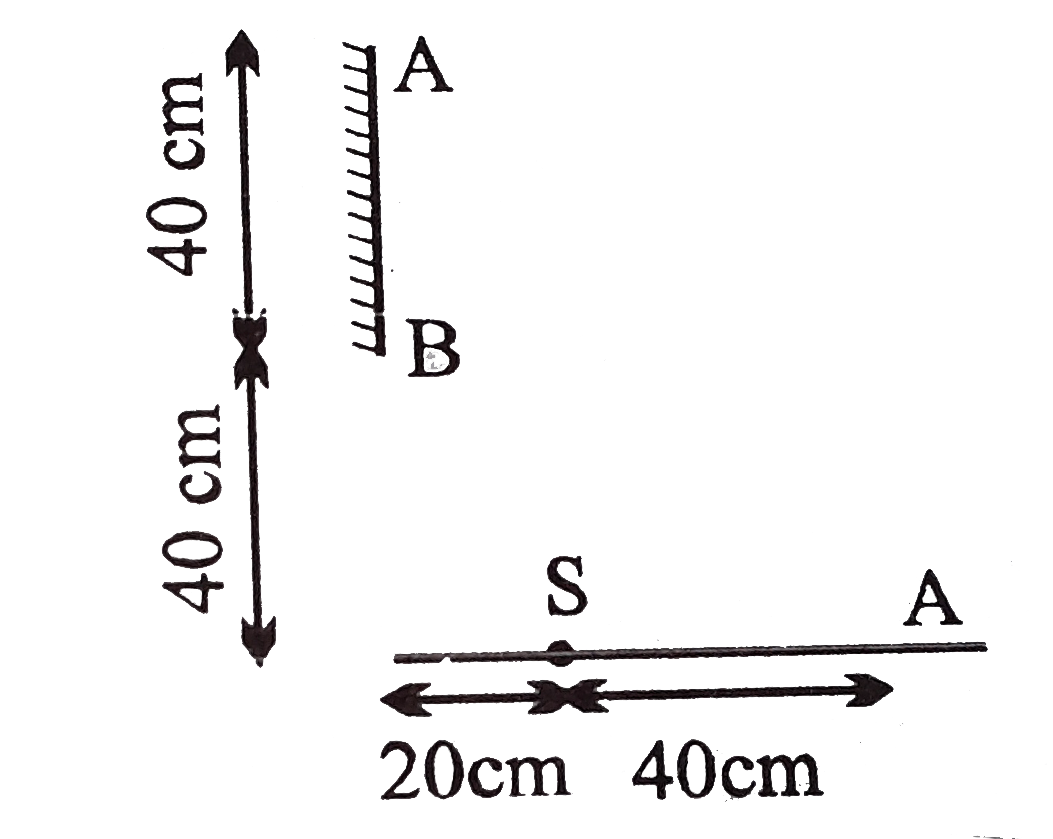
View Solutionin figure shown AB is a plane mirror of length 40cm placed at a height 40cm from ground . There is a light source S at a point on the ground ,Find the maximum and minimum height of a man(eye height)required to see the image of the source If he is standing at a pointA on ground shown in figure.
View SolutionIf your height is h and you want to see your complete image in a plane mirror, what should be the minimum size of the mirror ?
Strategy : You can see your complete image if light rays from the top of your head and light rays from your feet reach your eyes after reflection.
View SolutionA man of height H is standing in front of an inclined plane mirror at an angle θ from horizontal. The vertical separation between man and inclined plane is x. Man can see its complete image in length H(H+x)sinθH+2x of mirror .
(Given : x = H = 1m and θ=45∘)
_E01_114_Q01.png)
If man starts moving with velocity 1ms−1 horizontally, find out length of mirror at t=1s in which he can see his complete image.
View SolutionFind the minimum height of the obstacle so, that the sphere can stay in equilibrium.
View SolutionFind the height of image in the following diagram.
View SolutionA man of height H is standing in front of an inclined plane mirror at an angle θ from horizontal. The vertical separation between man and inclined plane is x. Man can see its complete image in length H(H+x)sinθH+2x of mirror .
(Given : x = H = 1m and θ=45∘)
_E01_113_Q01.png)
If man starts moving with velocity 1ms−1 along vertical, find out length of mirror at t = 1s in which he can see his complete image.
View SolutionA man is sitting in a room at 2m from a wall W1, wants to see the full height of the wall W2 behind him 4m high and 6m away from the facing wall W1. What is the minimum vertical length of mirror on the facing wall required for the purpose?
View SolutionA person 1.6 m tall is standing at the center between two walls three meter high. What is the minimum size of a plane mirror fixed on the wall in front of him, if he is to see the full height of the wall behind him?
View SolutionA man of height H is standing in front of an inclined plane mirror at an angle θ from horizontal. The vertical separation between man and inclined plane is x. Man can see its complete image in length H(H+x)sinθH+2x of mirror .
(Given : x = H = 1m and θ=45∘)
_E01_112_Q01.png)
If man starts moving with velocity √2ms−1 along inclined plane, find out length of mirror at t = 1s in which he can see his complete image,
View Solution
