The volume of the solid generated by the revolution of the curve y=a3a2+x2 about x-axis is :
12π3a2
π3a2
12π2a3
π2a3
The correct Answer is:C
Similar Questions
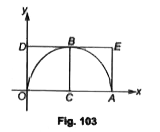
Given: the cycloid x=a(t−sint),y=a(1−cost),0≤t≤2π
Compute. (a) the areas of the surface formed by revolving the arc OBA about the x and y-axis,
(b) the volumes of the solids generated by revolving the figure OBAO about the y-axis and the axis BC,
(c ) the area of the surface generated by revolving the are BA about the axis BC,
(d) the volume of the solid generated by revolving the figure ODBEABO about the tangent line DE touching the figure at the vertex B,
(e ) the area of the surface formed by revolving the are of the cycloid (see item (d)]
View SolutionCompute the volume of the solid generated by revolving about the x-axis the area bounded by the axes of coordinates and the parabola x12+y12=a12.
View Solution
Knowledge Check
The area bounded by the curve a2y=x2(x+a) and the x-axis is
Aa2/3 sq. unitsBa2/4 sq. unitsC3a2/4 sq. unitsDa2/12 sq. units
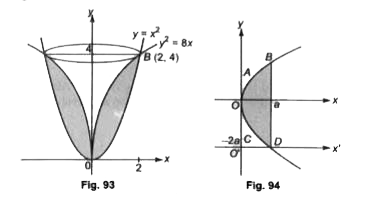
Compute the volume of the solid generated by revolving about the y-axis the figure bounded by the parabolas y=x2and8x=y2
View SolutionFind the volume of the solid generated by revolving about the x-axis the figure enclosed by the astroid : x=acos3t,y=asin3t.
View SolutionFind the volume of the solid generated by revolving about the line y=−2a the figure bounded by the parabola y2=4ax and the straight line x=a
View SolutionThe area bounded by the curve a2y=x2(x+a) and the x-axis is
View SolutionThe area bounded by the curve a2y=x2(x+a) and the x-axis is
View SolutionFind the volume of the solid generated by revolving about the x-axis the figure bounded by the straight lines y=x+1,y=2x+1andx=2.
View SolutionCompute the volume of the solid generated by revolving about the x-axis the figure bounded by the parabola y=0.25x2+2 and the straight line 5x−8y+14=0
View Solution
