Given: the cycloid
Compute. (a) the areas of the surface formed by revolving the arc OBA about the x and y-axis,
(b) the volumes of the solids generated by revolving the figure OBAO about the y-axis and the axis BC,
(c ) the area of the surface generated by revolving the are BA about the axis BC,
(d) the volume of the solid generated by revolving the figure ODBEABO about the tangent line DE touching the figure at the vertex B,
(e ) the area of the surface formed by revolving the are of the cycloid (see item (d)]
![Given: the cycloid x=a (t- sin t),y = a (1- cos t), 0 le t le 2pi Compute. (a) the areas of the surface formed by revolving the arc OBA about the x and y-axis, (b) the volumes of the solids generated by revolving the figure OBAO about the y-axis and the axis BC, (c ) the area of the surface generated by revolving the are BA about the axis BC, (d) the volume of the solid generated by revolving the figure ODBEABO about the tangent line DE touching the figure at the vertex B, (e ) the area of the surface formed by revolving the are of the cycloid (see item (d)]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/ARH_IAM_CAL_MAT_C07_E11_001_Q01.png)
The correct Answer is:(a) (b) (c ) (d) (e )
Topper's Solved these Questions
- APPLICATIONS OF THE DEFINITE INTEGRALBOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Computing Pressure, Work and Other Physical Quantities by the Definite Integrals
7 Videos
- APPLICATIONS OF THE DEFINITE INTEGRALBOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Computing Static Moments and Moments of Inertia. Determining Coordinates of the Centre of Gravity
15 Videos
- APPLICATIONS OF THE DEFINITE INTEGRALBOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Area of Surface of Revolution
10 Videos
- APPLICATION OF DIFFERENTIAL CALCULUS TO INVESTIGATION OF FUNCTIONSBOOK - IA MARONCHAPTER - APPLICATION OF DIFFERENTIAL CALCULUS TO INVESTIGATION OF FUNCTIONSEXERCISE - ADDITIONAL PROBLEMS
9 Videos
- BASIC CLASSES OF INTEGRABLE FUNCTIONS BOOK - IA MARONCHAPTER - BASIC CLASSES OF INTEGRABLE FUNCTIONS EXERCISE - 5.8 INTEGRATION OF OTHER TRANSCENDENTAL FUNCTIONS
5 Videos
Similar Questions
Knowledge Check
The volume of solid generated by revolving about the y-axis the figure bounded by the parabola y=x2andx=y2 is
A215πB245πC215πD524π
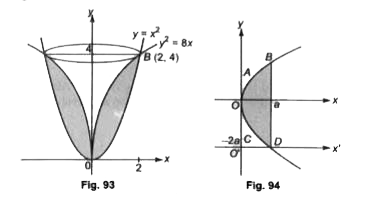
Compute the volume of the solid generated by revolving about the y-axis the figure bounded by the parabolas y=x2and8x=y2
View SolutionFind the area of the surface formed by revolving the astroid x2/3+y2/3=a2/3 about the x-axis
View SolutionFind the area of the surface generated by revolving about the x-axis a closed contour OABCO formed by the curves y=x2andx=y2
View SolutionFind the volume of the solid generated by revolving, about the x-axis, the infinite branch of the curve y=2(1x−1x2)forx≥1.
View SolutionFind the volume of the solid generated by revolving about the x-axis the figure bounded by the straight lines y=x+1,y=2x+1andx=2.
View SolutionFind the volume of the solid generated by revolving about the line y=−2a the figure bounded by the parabola y2=4ax and the straight line x=a
View SolutionFind the area of the surface obtained by revolving a loop of the curve 9ax2=y(3a−y)2 about the y-axis
View Solution
