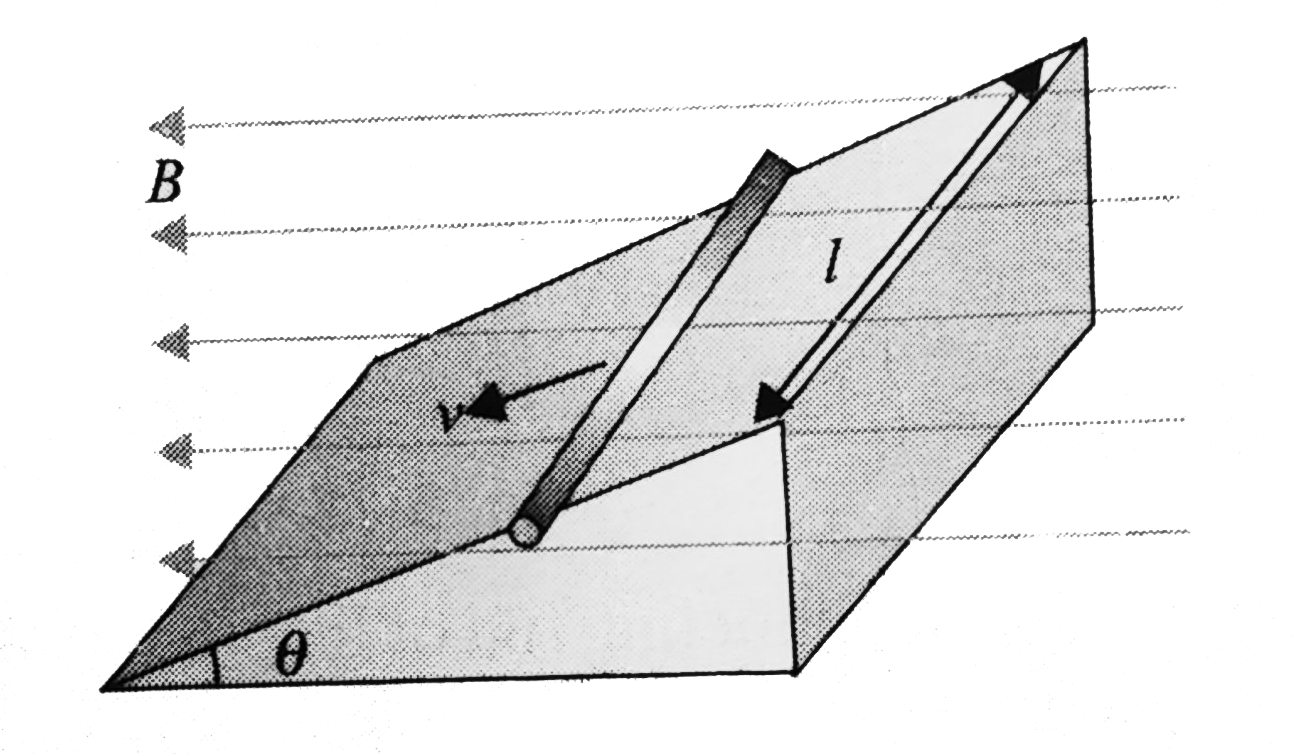
A conducting rod of length l and mass m is moving down a smooth inclined plane of inclination θ with constant velocity V as shown in figure. A current I is flowing in the conductor in a direction perpendicular to paper inwards. Let a vertically upward magnetic field →B be existent in space. Then, what is the magnitude of magnetic field →B?
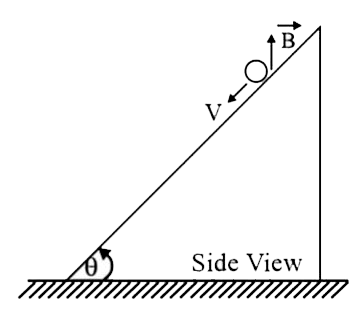
mgilsin(θ)
mhiltan(θ)
mgcos(θ)il
mgilsin(θ)
The correct Answer is:B
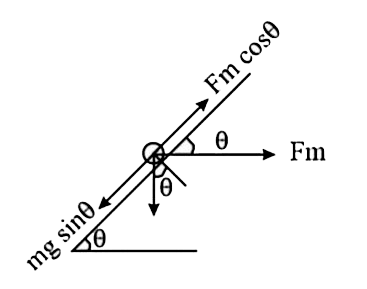
The magnetic force due to the conducting rod of length I and mass m is,
Fm=i(→l×→B), the angle between l and B is 90∘
Fm=ilB
The horizontal component of Fm is
F1=FmCosθ
The force due to gravity is F=mg
The horizontal component of force due to gravity is
FH=mgsinθ
Rod will move downward with constant velocity if,
F1=FH
⇒FmCosθ=mgSinθ
⇒ilBCosθ=mgSinθ
⇒B=mgilSinθCosθ=mgiltanθ
Topper's Solved these Questions
Similar Questions
A conducting rod of length l and mass m is moving down a smooth inclined plane of inclination θ with constant i is flowing in the conductor in a direction perpendicular to paper inwards. A vertically upward magnetic field →B exists in space. Then, magnitude of magnetic field →B is

View SolutionA conducting rod of length l and mass m is moving down a smooth inclined plane of inclination θ with constant i is flowing in the conductor in a direction perpendicular to paper inwards. A vertically upward magnetic field →B exists in space. Then, magnitude of magnetic field →B is

View Solution
Knowledge Check
A conducting rod of length l and mass m is moving down a smooth inclined plane of inclination θ with constant i is flowing in the conductor in a direction perpendicular to paper inwards. A vertically upward magnetic field →B exists in space. Then, magnitude of magnetic field →B is
 AmgsinθIlBmgtanθIlCmgcosθIlDmgsinθI
AmgsinθIlBmgtanθIlCmgcosθIlDmgsinθIA conducting rod of length l and mass m is moving down a smooth inclined plane of inclination θ with constant i is flowing in the conductor in a direction perpendicular to paper inwards. A vertically upward magnetic field →B exists in space. Then, magnitude of magnetic field →B is
 AmgilsinθBmgiltanθCmgcosθilDmgilsinθ
AmgilsinθBmgiltanθCmgcosθilDmgilsinθA conducting stick of length 2L and mass m is moving down a smooth inclined plane of inclination 60∘ with constant speed 5m/s. A current 2A is flowing in the conductor perpendicular to the paper inwards. A vertically upward magnetic field B exists in space there. The magnitude of magnetic field B is
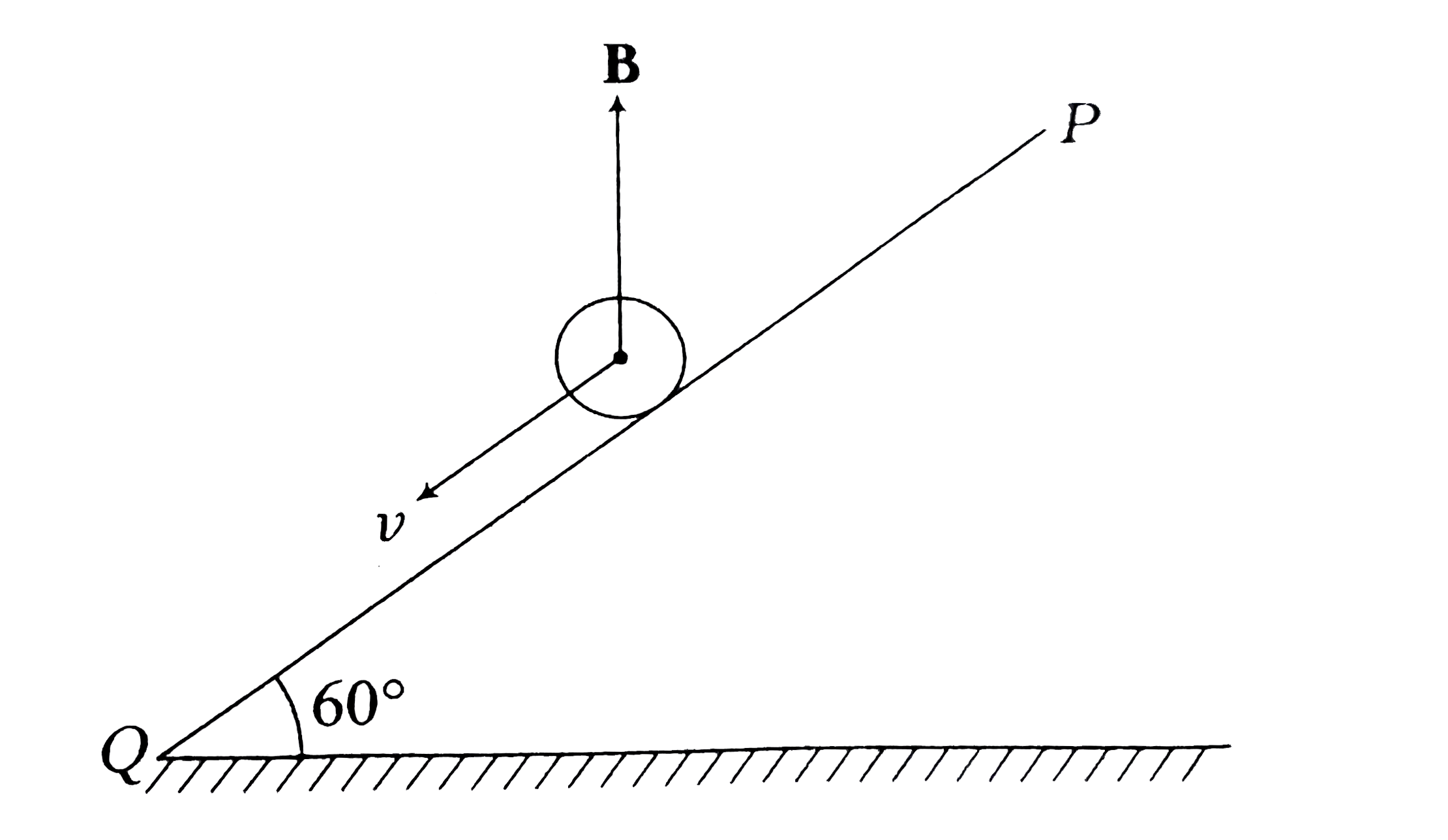 Amg4LBmgLC√3mg4LD√3mg2L
Amg4LBmgLC√3mg4LD√3mg2L
A conducting stick of length 2L and mass m is moving down a smooth inclined plane of inclination 60∘ with constant speed 5m/s. A current 2A is flowing in the conductor perpendicular to the paper inwards. A vertically upward magnetic field B exists in space there. The magnitude of magnetic field B is

View SolutionA V-shaped conducting wire is moved inside a magnetic field as shown in figure. Magnetic fiel d is perpendicular to paper inwards. Then
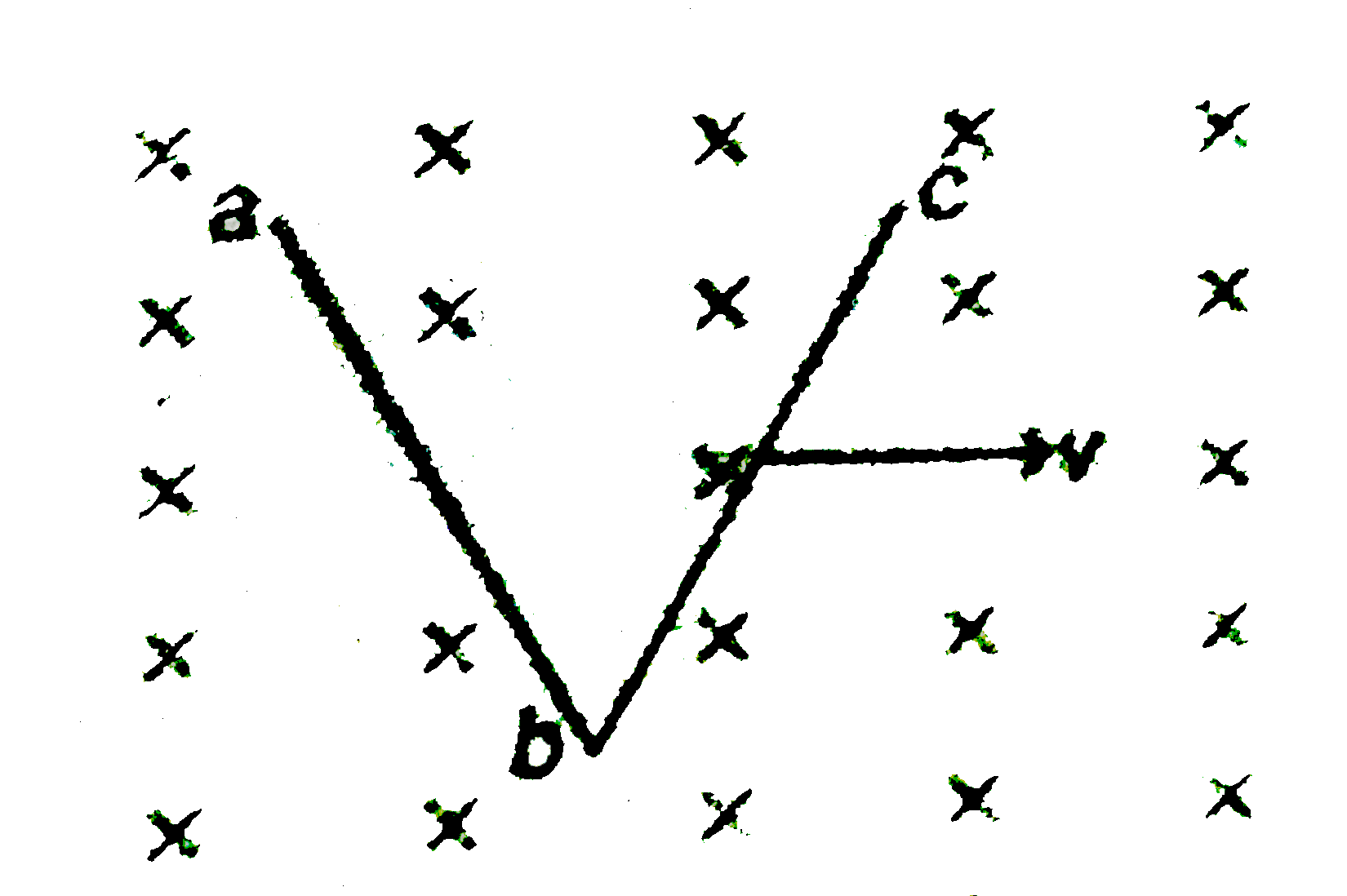
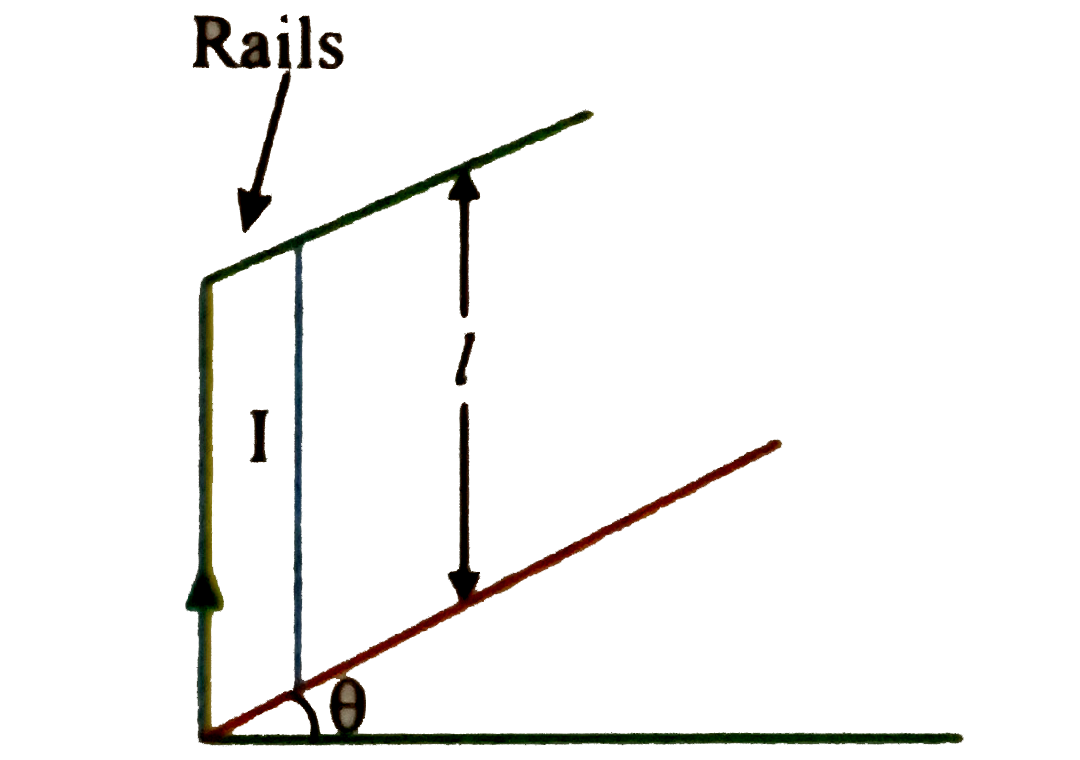
View SolutionA rod of mass m, length l and resistance R is sliding down on a smooth inclined parallel rails with a cinstant velocity v. If a uniform horizontal magnetic field B exists, then find thevalue of B.
View SolutionA conducting wire of length l and mass m is placed on two inclined rails as shown in figure. A current I is flowig in the wire in the direction shown When no magnetic field is present in the region, the wire is just on the verge of sliding. When a vertically upward magnetic field is switched on, the wire starts moving up the incline. The distance travelled by the wire as a function of time t will be
View SolutionA V-shaped conducting wire is moved inside a magnetic field as shown in figure. Magnetic fiel d is perpendicular to paper inwards. Then

View Solution
