A stationary hydrogen atom emits a photon corresponding to first line of the Lyman series. What velocity does the atom acquire?

A

3.25

B

3.5

C

1.5

D

$4.0$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

The problem is similar to gun firing. The momentum of photon is $\frac{h}{λ}$
According to conservation principle of momentum,

${\vec{P}}_{i} = {\vec{P}}_{f}$
$0 = m v - \frac{h}{λ}$
$∴ m v = \frac{h}{λ}$
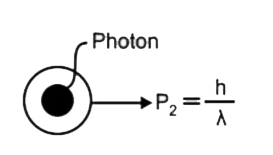
Here v is recoiling speed of H-atom. According to energy conservation principle,
$E_{i} - E_{f}$
The initial energy of atom is $E_{i} = - \frac{13.6}{n^{2}} e V = - \frac{13.6}{{(1)}^{2}} e V$
$∴ E_{i} = - \frac{13.6}{n^{2}} e V$
The final energy of the system (atom + photon) is $E_{f} = K . E .$ of atom + energy of electron + photon energy
$= \frac{1}{2} m v^{2} - \frac{13.6}{{(1)}^{2}} e V + \frac{h c}{λ}$
$∴ E_{i} = E_{f}$
$∴ - \frac{13.6}{4} e V = \frac{1}{2} m v^{2} - 13.6 e V + \frac{h c}{λ}$
or $(13.6 - \frac{13.6}{4}) e V = \frac{1}{2} m v^{2} + \frac{h c}{λ}$
or $(13.6 \times \frac{3}{4}) e V = \frac{1}{2} m v^{2} + m v c$
[From eqn(i)]
or $10.2 \times 1.6 \times 10^{- 19}$ joule $= \frac{1}{2} m v^{2} + m v c$
Here $m = 1.67 \times 10^{- 27} k g, c = 3 \times 10^{8} m / s$
After solving $v = 3.25 m / s$

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A stationary hydrogen atom emits photon corresponding to the first line of Lyman series. If R is the Rydberg constant and M is the mass of the atom, then the velocity acquired by the atom is
A $\frac{3 R h}{4 M}$
B $\frac{4 M}{3 R h}$
C $\frac{R h}{4 M}$
D $\frac{4 M}{R h}$
Question 2 - Select One
A stationary hydrogen atom emits a photon corresponding to first line of the Lyman series. What velocity does the atom acquire?
A3.25
B3.5
C1.5
D $4.0$
Question 3 - Select One
A hydrogen atom of mass m emits a photon corresponding to the fourth line of Brackett series and recoils. If R is the Rydberg's constant and h is the Planck's constant, then recoiling velocity is
A $\frac{3 R h}{36 m}$
B $\frac{3 R h}{64 m}$
C $\frac{7 R h}{120 m}$
D $\frac{7 R h}{3 m}$

NTA MOCK TESTS-NTA TPC JEE MAIN TEST 63-PHYSICS

Home
Ask Doubt
Profile