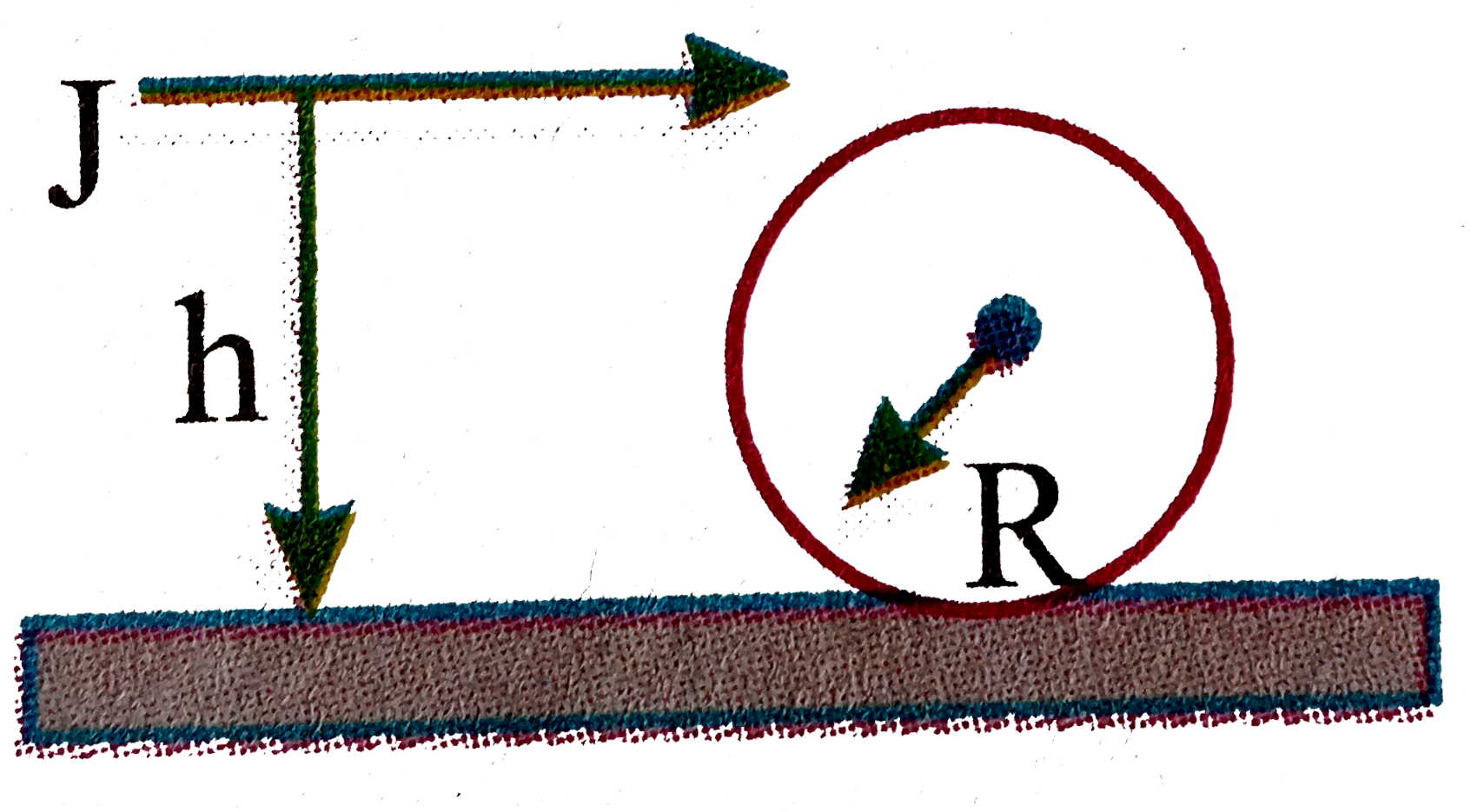
A uniform solid sphere of mass M and radius R is placed on a smooth horizontal surface. It is given a horizontal impulse J at a height h above the centre of mass and sphere starts rolling. Then the value of h and speed of ccentre of mass are
h=25Randv=JM
h=25Randv=25JM
h=75Randv=75JM
h=75Randv=JM
The correct Answer is:A
To solve the problem, we need to analyze the motion of the uniform solid sphere after it receives a horizontal impulse J at a height h above its center of mass. We will determine the value of h and the speed of the center of mass vcm after the impulse is applied.
1. Understanding the Setup:
- We have a uniform solid sphere of mass M and radius R.
- The sphere is placed on a smooth horizontal surface, meaning there is no friction.
- An impulse J is applied at a height h above the center of mass.
2. Torque and Angular Acceleration:
- The impulse J creates a torque about the center of mass. The torque τ due to the impulse is given by:
τ=J⋅h
- The moment of inertia I of a solid sphere about its center of mass is:
I=25MR2
- The angular acceleration α can be related to the torque by:
τ=Iα⟹J⋅h=25MR2α
3. Linear Acceleration:
- The linear acceleration a of the center of mass is related to the angular acceleration α by the equation for rolling without slipping:
a=Rα
- Substituting α from the torque equation, we get:
a=R⋅J⋅h25MR2=5J⋅h2MR
4. Impulse-Momentum Theorem:
- The impulse J also relates to the change in linear momentum of the sphere. The change in momentum is:
J=Δp=Mvcm−0⟹Mvcm=J⟹vcm=JM
5. Finding the Height h:
- For pure rolling to occur, the center of mass must rise to a height where the impulse is effectively applied. The height h can be determined from the condition that the angular momentum about the center of mass must be consistent with the linear motion:
h=25R
- This height corresponds to the point where the impulse causes the sphere to start rolling without slipping.
6. Final Results:
- The value of h is:
h=25R
- The speed of the center of mass vcm is:
vcm=JM
Summary of Results:
- Height h=25R
- Speed of center of mass vcm=JM
- We have a uniform solid sphere of mass M and radius R.
- The sphere is placed on a smooth horizontal surface, meaning there is no friction.
- An impulse J is applied at a height h above the center of mass.
- The impulse J creates a torque about the center of mass. The torque τ due to the impulse is given by:
τ=J⋅h
- The moment of inertia I of a solid sphere about its center of mass is:
I=25MR2
- The angular acceleration α can be related to the torque by:
τ=Iα⟹J⋅h=25MR2α
- The linear acceleration a of the center of mass is related to the angular acceleration α by the equation for rolling without slipping:
a=Rα
- Substituting α from the torque equation, we get:
a=R⋅J⋅h25MR2=5J⋅h2MR
- The impulse J also relates to the change in linear momentum of the sphere. The change in momentum is:
J=Δp=Mvcm−0⟹Mvcm=J⟹vcm=JM
- For pure rolling to occur, the center of mass must rise to a height where the impulse is effectively applied. The height h can be determined from the condition that the angular momentum about the center of mass must be consistent with the linear motion:
h=25R
- This height corresponds to the point where the impulse causes the sphere to start rolling without slipping.
- The value of h is:
h=25R
- The speed of the center of mass vcm is:
vcm=JM
- Height h=25R
- Speed of center of mass vcm=JM
Topper's Solved these Questions
- NTA TPC JEE MAIN TEST 100BOOK - NTA MOCK TESTSCHAPTER - NTA TPC JEE MAIN TEST 100EXERCISE - PHYSICS (SUBJECTIVE NUMERICAL)
10 Videos
- NTA NEET TEST 98BOOK - NTA MOCK TESTSCHAPTER - NTA NEET TEST 98EXERCISE - PHYSICS
45 Videos
- NTA TPC JEE MAIN TEST 101BOOK - NTA MOCK TESTSCHAPTER - NTA TPC JEE MAIN TEST 101EXERCISE - PHYSICS
30 Videos
Similar Questions
A uniform sphere of mass m and radius R is placed on a rough horizontal surface. The sphere is struck horizontally at a heighth from the floor. Match the following:
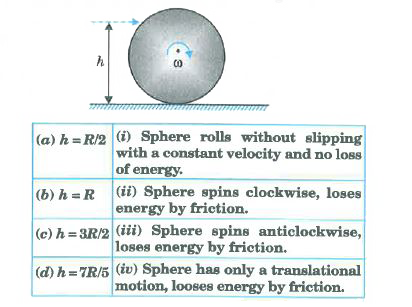
View SolutionA unifrom sphere of mass m and radius R is placed on a rough horizontal surface (figure) .the sphere is stuuck horizontally at a height h from the floor . Match the following
View Solution
Knowledge Check

A solid sphere of mass ma nd radis R is placed on a rough horizontal surface A horizontal force F is applied to sphere at a height h, (0≤h≤R) from centre. If sphere rolls slipping then,AThe maximum possible acceleration of sphere is FMBThe maximum possible acceleration of sphere is 10F7MCThe magnitude of friction force acting on the sphere is zero if h=2R5DThe magnitude of friction force acting on the sphere is zero h=3R4.A solid sphere of mass M and radius R is placed on a rough horizontal surface. It is stuck by a horizontal cue stick at a height h above the surface. The value of h so that the sphere performs pure rolling motion immediately after it has been stuck is
 A2R5B5R2C7R5D9R5
A2R5B5R2C7R5D9R5A hollow cylinder of mass m and radius r is kept on a smooth horizontal surface. Horizontal force F is applied at a height above the centre. Find the value of h/R so that ring performs pure rolling on the surface.
A1B12C13D25
A solid sphere of mass m and radius R is placed on a smooth horizontal surface. A sudden blow is given horizontally to the sphere at a height h=4R/5 above the centre line. If I is the impulse of the blow then find
(a) the minimum time after which the highest point B will touch the ground.
(b) the displacement of the centre of mass during this internal.
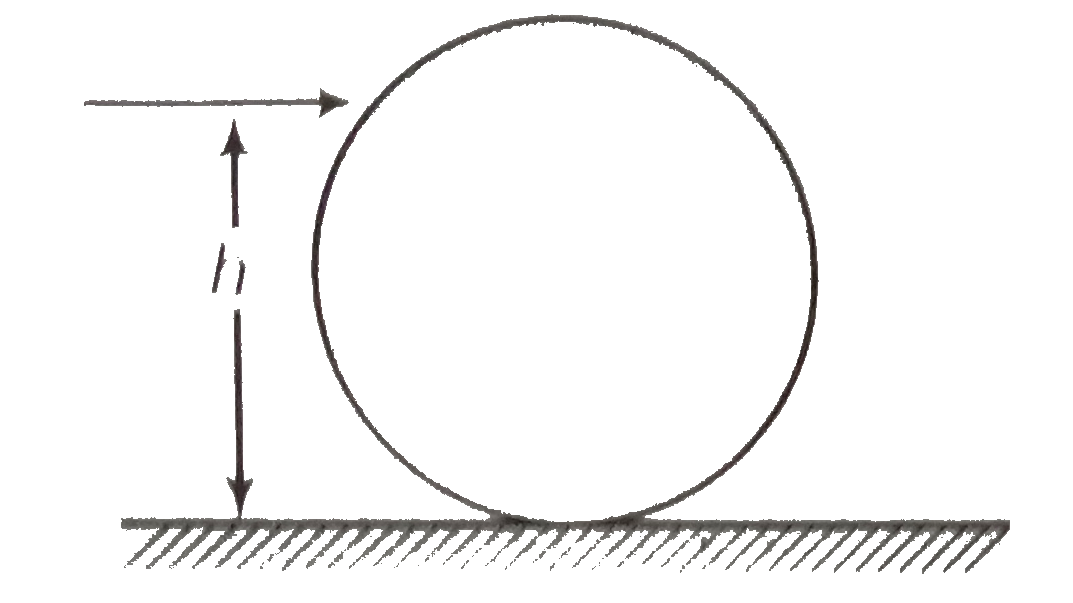
View SolutionA uniform sphere of mass m and radius R is placed on a rough horizontal surface. The spher is struck horizontally at a height h from the floor. Show that the sphere rolls without slipping with a constant velocity, when h=7R/5.
View SolutionA loop of mass M and radius R is rolling on a smooth horizontal surface with speed 'v'. It's total kinetic energy is
View SolutionA solid sphere of radius R is placed on a smooth horizontal surface. A horizontal force F is applied at height h from the lowest point. For the maximum acceleration of the centre of mass
View Solution
A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder is
View Solution
