During vertical motion under gravity a body passes through a point at 4s and 12 s in upwards and downwards journeys. The speed at this point is
g
2g
3g
4g
The correct Answer is:D
Time for (rise CB + fall BC) is 8 s.
Time for rise CB = Time for fall BC = 4s
Speed at C = speed gained in 4s
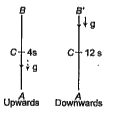
The body after projection reaches the highest point B where the speed becomes zero. Then, it falls under gravity.
Similar Questions
A body thrown vertically upwards crosses a point p on its path after 2 seconds during its upward journey and again crosses the same point after 6 seconds during downward journey. Find the height of the point p from the ground (as a multiple of acceleration due to gravity)
View SolutionA body is falling freely under gravity. It passes two points A and B (A being higher than B) 20m apart vertically in 2s. Find the elevation of the point above A from where it began to fall.
View Solution
Knowledge Check
During upwards and downwards journeys under gravity, a body passes through a point at 2 s and 14 s. The total height from ground is
A4gB8gC16gD32gDuring upwards and downwards journeys under gravity, a body passes through a point at 2 s and 14 s. The total height from ground is
A4gB8gC16gD32gA particle is projected vertically upwards. What is the value of acceleration
(i) during upward journey,
(ii) during downward journey and
(iii) at highest point?A10 ms2B−10 ms2C10 ms2 −10 ms20 ms2DVariable
A ball is thrown vertically up with a velocity u. It passes three points A,B and C in its upward journey with velocities us,u3 and u4, respectively. Find ABBC.
View SolutionA ball 4 s after the instant it was thrown from the ground passes through a point P, and strikes the ground after 5 s from the instant it passes through the point P. Assuming acceleration due to gravity to be 9.8m/s2 find height of the point P above the ground.
View SolutionA ball 4 s after the instant it was thrown from the ground passes through a point P, and strikes the ground after 5 s from the instant it passes through the point P. Assuming acceleration due to gravity to be 9.8m/s2 find height of the point P above the ground.
View SolutionA particle is projected vertically upwards. What is the value of acceleration
(i) during upward journey,
(ii) during downward journey and
(iii) at highest point?
View SolutionTwo balls of different masses are thrown vertically upwards with the same speed . They pass through the point of projection in their downward motion with the same speed ( Neglect air resistance ).
View Solution
