The feasible region of a sytem of linear inequations is shown below. If Z = 2x + y, then the minimum value of Z is:
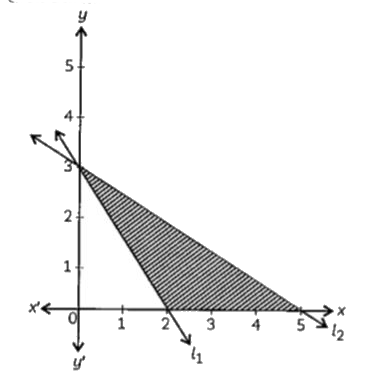
0
3
4
10
Step by step video & image solution for The feasible region of a sytem of linear inequations is shown below. If Z = 2x + y, then the minimum value of Z is: by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SAMPLE PAPER 09BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 09EXERCISE - SECTIONS-C
8 Videos
- SAMPLE PAPER 09BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 09EXERCISE - SECTIONS-C
8 Videos
- SAMPLE PAPER - 5BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER - 5EXERCISE - Section - C
10 Videos
- SAMPLE PAPER 11 BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 11 EXERCISE - SECTION- C
4 Videos
Similar Questions
The feasible region of a system of linear inequalities is shown below. If the objective function is maximise Z = 25x + 15y, then the maximum value of Z occurs at:
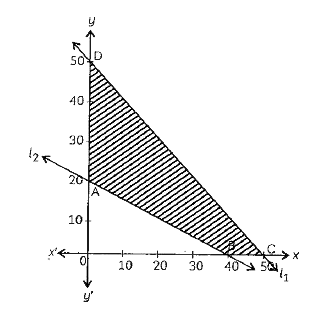
View SolutionThe feasible region of a system of linear inequations is shown shaded in the following figure If Z=4x+3y, then Zmax−Zmin=
View Solution
Knowledge Check
The feasible region of the system of linear inequations x+2y≤120,x+y≥60,x−2y≥0,x,y≥0:
Ais bounded and lies in first quadrant .Bis unbounded and lies in first quadrant.Cdoes in first quadrant .Ddoes not exist.Which of the following is a corner point of the feasible region of system of linear inequations 2x + 3y ≤6,x+4y≤4 and x, y ≥0 ?
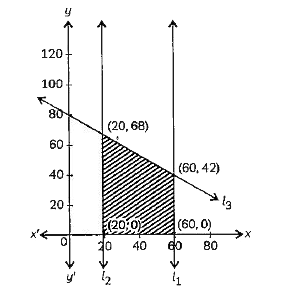
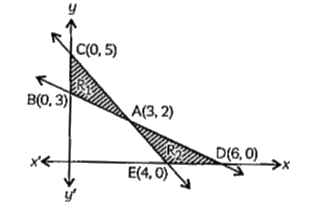
A(1,0)B(1,1)C(0,3)D(125,25)The feasible regions for two LPP is show in the following figure.
Based on the given information, answer the following questions:
If R1 is the feasible region, and the ojective function is Z1=2x−y, then the maximum value of Z1 occurs at:
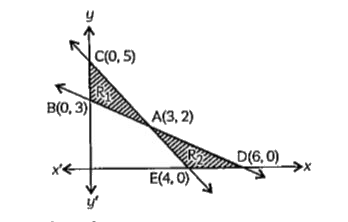 A(0,5)B(0,3)C(3,2)D(6,0)
A(0,5)B(0,3)C(3,2)D(6,0)
The feasible region by a system of linear inequalities is shown shaded in the given graph.
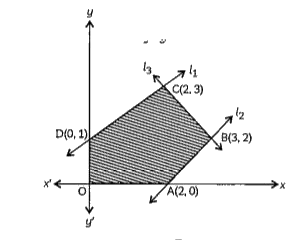
If Z = 4x - 6y, then Zmin =
View SolutionThe feasible region of a system of linear inequalities is shown shaded in the following graph . If the objective function is Z = ax + by where a,b are constants and the maximum of Z occurs at points A and D then :
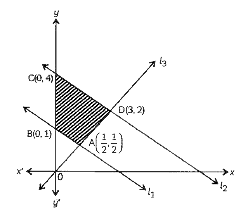
View SolutionThe feasible regions for two LPP is show in the following figure.
Based on the given information, answer the following questions:
If R2 is the feasible region and the objective function is Z2=4x+3y, then the minimum value of Z2 occurs at:
View SolutionA feasible region of a system of linear inequalities is said to be ..., if it can be enclosed within a circle.
View SolutionThe feasible region for an LPP is shown in the below. Let F = 3x - 4y be the objective function. Maximum value of F is :
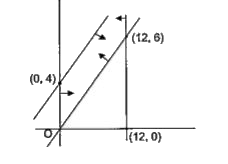
View Solution
