ΔABC~ΔDEF such that DE=3cm,EF=2cm,DF=2.5cm and BC=4cm. Find the perimeter of ΔABC.
To solve the problem, we need to find the perimeter of triangle ABC given that triangle ABC is similar to triangle DEF. We have the lengths of the sides of triangle DEF and one side of triangle ABC.
1. Identify the sides of the triangles:
- For triangle DEF, we have:
- DE = 3 cm
- EF = 2 cm
- DF = 2.5 cm
- For triangle ABC, we know:
- BC = 4 cm
- We need to find the lengths of AB and AC.
2. Set up the ratio of corresponding sides:
Since the triangles are similar, the ratios of their corresponding sides are equal:
ABDE=BCEF=ACDF
3. Substitute the known values into the ratios:
Using the values we have:
- DE=3 cm
- EF=2 cm
- DF=2.5 cm
- BC=4 cm
We can write the ratios as:
AB3=42=AC2.5
4. Calculate the ratio:
From 42, we simplify to:
42=2
So, we have:
AB3=2andAC2.5=2
5. Solve for AB:
From AB3=2:
AB=2×3=6 cm
6. Solve for AC:
From AC2.5=2:
AC=2×2.5=5 cm
7. Calculate the perimeter of triangle ABC:
The perimeter P of triangle ABC is given by:
P=AB+BC+AC
Substituting the values we found:
P=6+4+5=15 cm
Final Answer:
The perimeter of triangle ABC is 15 cm.
- For triangle DEF, we have:
- DE = 3 cm
- EF = 2 cm
- DF = 2.5 cm
- For triangle ABC, we know:
- BC = 4 cm
- We need to find the lengths of AB and AC.
Since the triangles are similar, the ratios of their corresponding sides are equal:
ABDE=BCEF=ACDF
Using the values we have:
- DE=3 cm
- EF=2 cm
- DF=2.5 cm
- BC=4 cm
AB3=42=AC2.5
From 42, we simplify to:
42=2
So, we have:
AB3=2andAC2.5=2
From AB3=2:
AB=2×3=6 cm
From AC2.5=2:
AC=2×2.5=5 cm
The perimeter P of triangle ABC is given by:
P=AB+BC+AC
Substituting the values we found:
P=6+4+5=15 cm
The perimeter of triangle ABC is 15 cm.
Topper's Solved these Questions
- SAMPLE PAPER 11BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 11EXERCISE - PART -A (SECTION-II)
20 Videos
- SAMPLE PAPER 11BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 11EXERCISE - PART -B (SECTION-III)
8 Videos
- SAMPLE PAPER 10 SOLVED BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 10 SOLVED EXERCISE - PART - B (SECTION - V)
4 Videos
- SAMPLE PAPER 11 (SELF - ASSESSMENT)BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 11 (SELF - ASSESSMENT)EXERCISE - SECTION -C
10 Videos
Similar Questions
ΔABC~ΔDEF such that DE = 3 cm , EF = 2 cm , DF = 2.5 and BC = 4 cm . Find the perimeter of ΔABC.
View SolutionFind the perimeter of ΔABC, if AP=12 cm
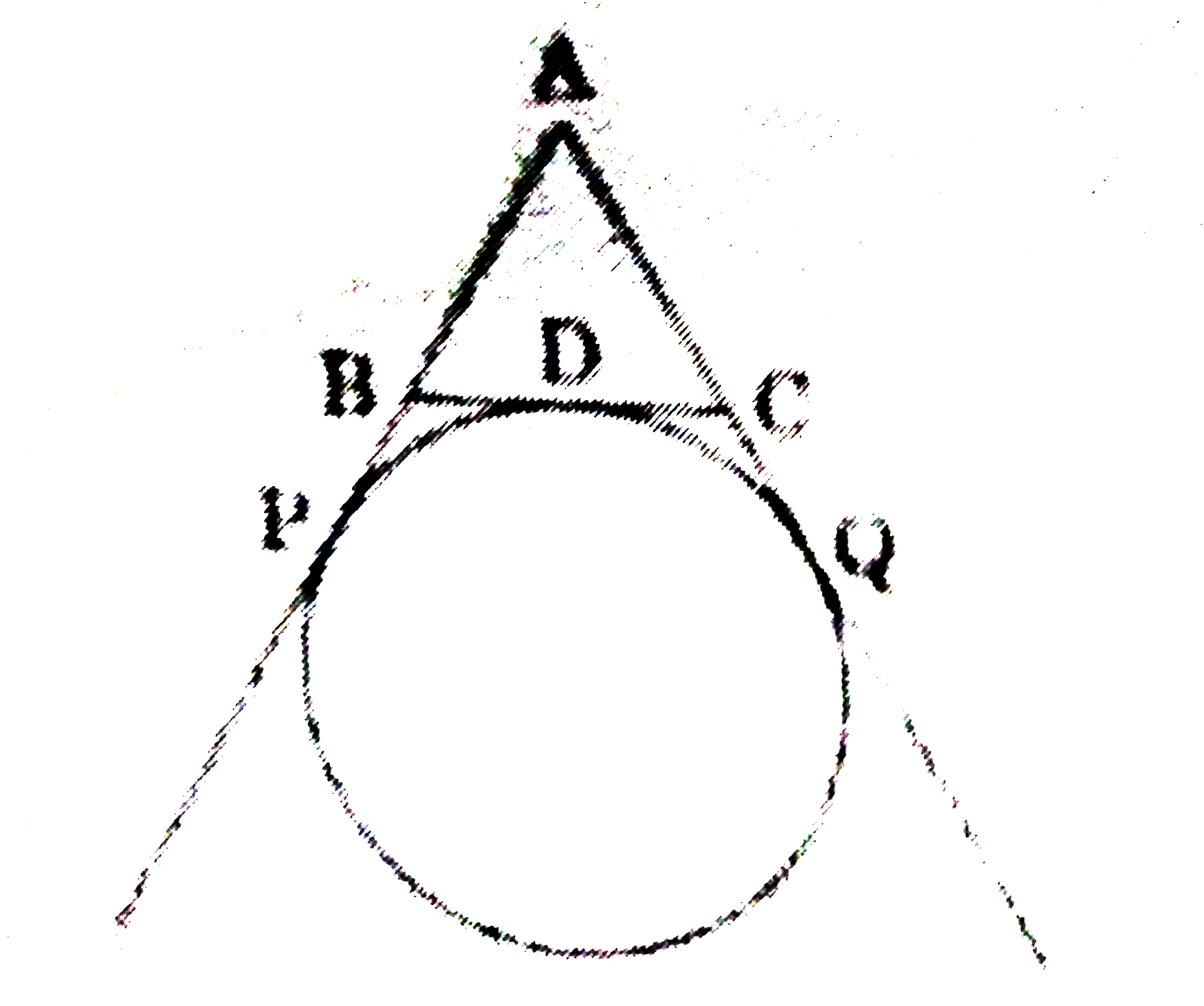
View Solution△ABC−△DEF such that DE=3cm,EF=2cm,DF=2.5cm and BC=4cm. Find perimeter of △ABC.
View SolutionIf ΔABC~ΔDEF AB =4 cm , DE = 6 cm, EF =9 cm and FD =12 cm find the perimeter of ΔABC .
View SolutionIf ΔABC~ΔDEF, AB=4cm, DE=6,EF=9 cm and FD=12 cm, then find the perimeter of ΔABC.
View SolutionIt is given that ΔABC~ΔEDF such that AB=5 cm, AC=7 cm, DF= 15 cm and DE = 12 cm. Find the lengths of the remaining sides of the triangles.
View SolutionΔABC~ΔDEF. If AB= 4cm,BC = 3.5cm,CA = 2.5cmandDF = 7.5cm, then find perimeter of ΔDEF.
View SolutionΔABC~ΔDEF such that AB = 9.1 cm and DE =6.5 cm. If the perimeter of ΔDEF is 25 cm, what is the perimeter of ΔABC?
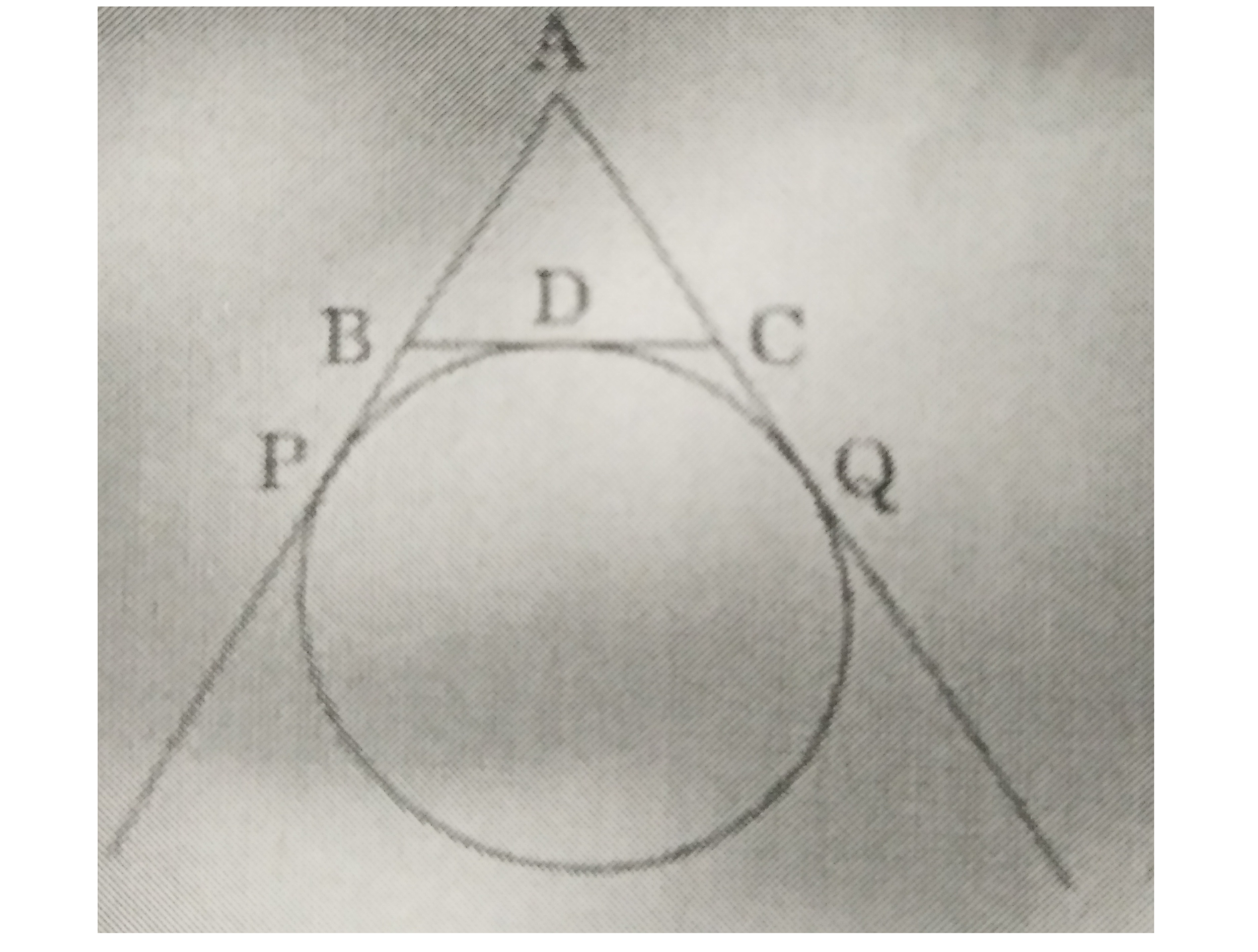
View SolutionIn Figure , find the perimeter of ΔABC. If AP = 12 cm.
View SolutionIf ΔABC is similar to ΔDEF such that BC=3cm,EF=4cm and area of ΔABC=54cm2. Find the area of ΔDEF.
View Solution
