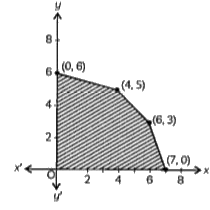
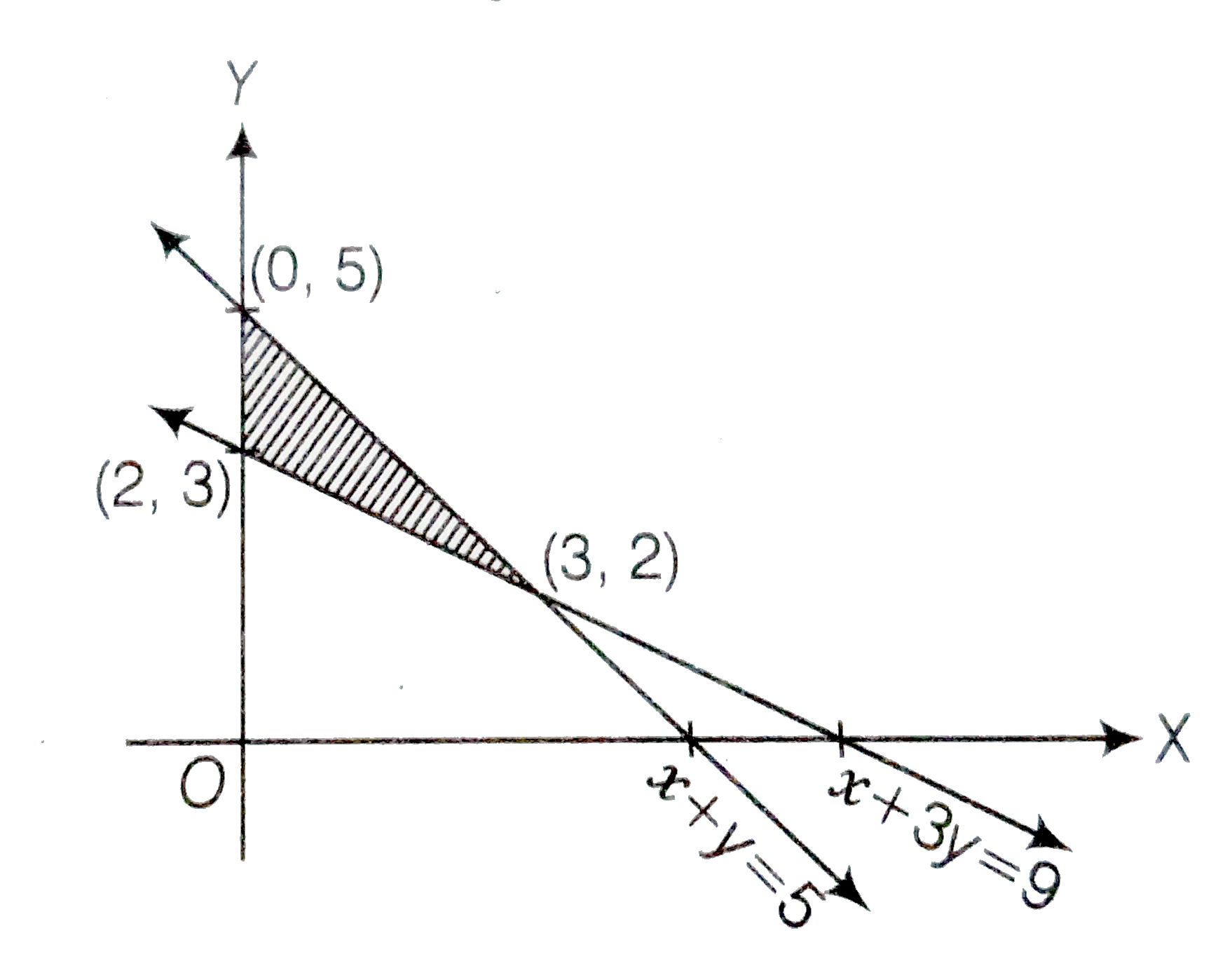
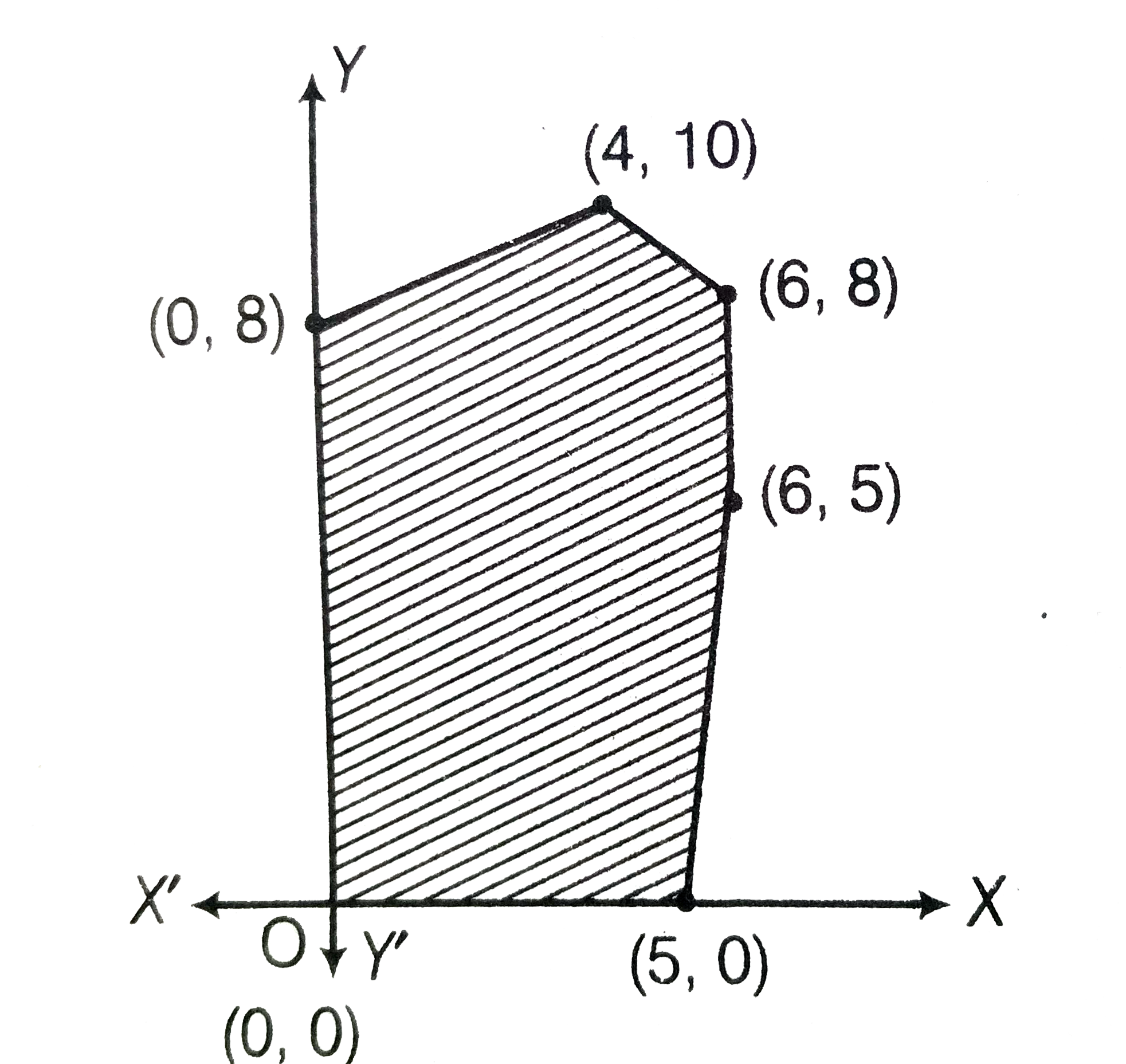
In the given graph,the feasible region for a LPP is shaded. The objective function Z=2x−3y, will be minimum at:
a) (4,10)
b) (6,8) c) (0,8) d) (6,5)
Step by step video & image solution for In the given graph,the feasible region for a LPP is shaded. The objective function Z=2x-3y, will be minimum at: a) (4,10) b) (6,8) c) (0,8) d) (6,5) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Similar Questions
Knowledge Check
- Question 1 - Select One
In the given figure, if the shaded region is the feasible region and the objective function is z = x - 2y, the minimum value of Z occurs at:
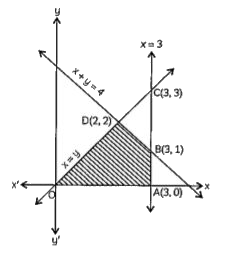 AOBBCCDD
AOBBCCDD - Question 2 - Select One
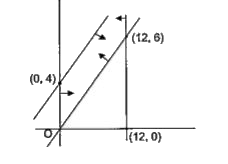
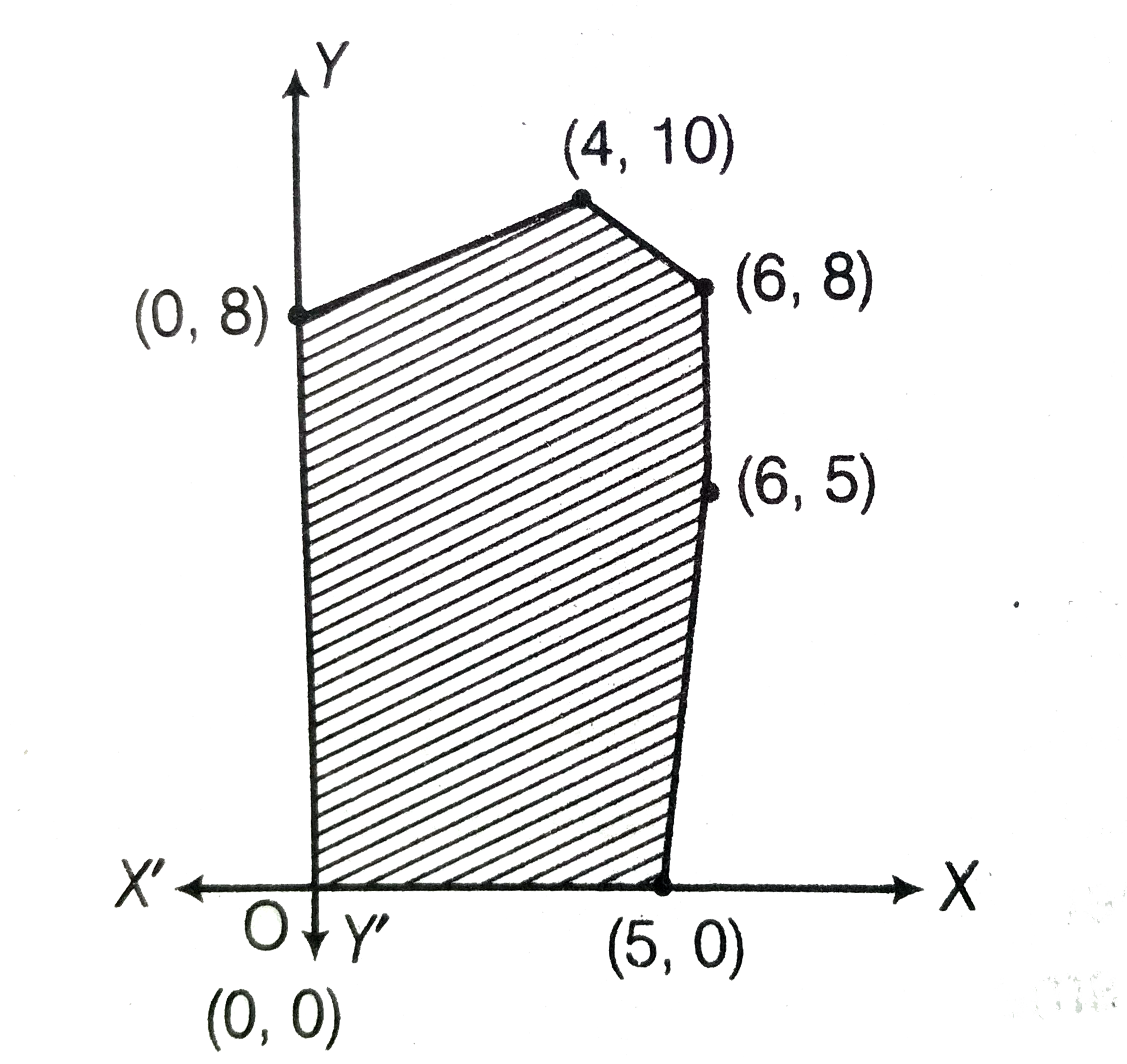
In the following the feasible region (shaded) for a LPP is shown Determine the maximum and minimum value of Z=x+2y
 Amaximum = 7 minimum = 479Bmaximum = 9 minimum = 317Cmaximum = 10 minimum = 345Dmaximum = 8 minimum = 547
Amaximum = 7 minimum = 479Bmaximum = 9 minimum = 317Cmaximum = 10 minimum = 345Dmaximum = 8 minimum = 547 - Question 3 - Select One
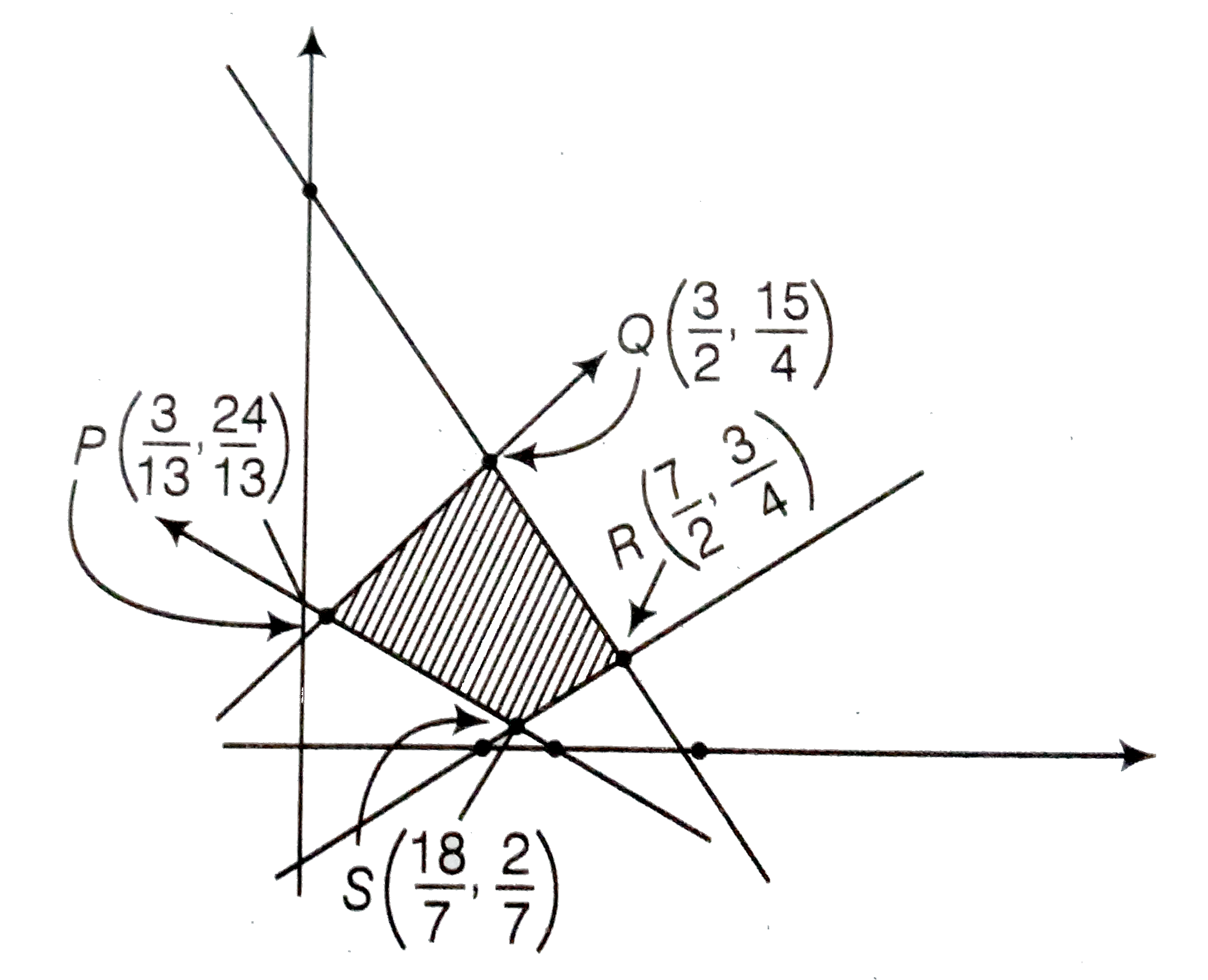
The feasible region for an LPP is shown in following figure. Find the minimum value of Z=11x+7y.
 A22B23C24D21
A22B23C24D21
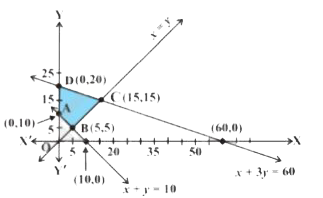
The feasible solution for a LPP is hown as below,
Let Z =3x -4y be the objective function . Then, Minimum of Z occurs atView SolutionBased on the given shaded region as the feasible region in the graph, at which point(s) is the objective function Z=3x+9y maximum?
 View Solution
View SolutionBased on the given shaded region as the feasible region in the graph, at which point(s) is the objective function Z = 3x + 9y is maximum?
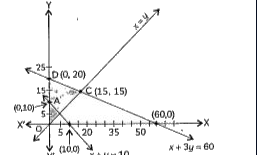 View Solution
View SolutionThe feasible region of an LPP is shown in the figure. If z=3x+9y ,then the minimum value of z occurs at
View SolutionThe feasible solution for a LPP is hown as below,
Let Z =3x -4y be the objective function . Then,
Maximum of Z occurs atView Solution