A solid sphere of mass 2 kg and radius R rolls on a smooth horizontal surface at and then rolls up on a smooth inclined plane of inclination angle with horizontal. Calculate the height (in m ) attained by the sphere before it stops.(Take
The correct Answer is:
at .h.
Topper's Solved these Questions
Similar Questions
A solid sphere rolls on a smooth horizontal surface at 10m/s and then rolls up a smooth inclined plane of inclination 30∘ with horizontal. The mass of the sphere is 2kg. Find the height attained by the sphere before it stops (in m).
View SolutionA solid sphere rolls on a smooth horizontal surface at 10m/s and then rolls up a smooth inclined plane of inclination 30∘ with horizontal. The mass of the sphere is 2kg. Find the height attained by the sphere before it stops (in m).
View Solution
Knowledge Check
A uniform solid sphere rolls on a horizontal surface at 20 m/s. It, then, rolls up a plane inclined at
A16 mB20 mC28 mD36 mA solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
AThe kinetic energy of rotation is 15mv20.BThe total kinetic energy is 710mv20.CThe mechanical energy (assume the ground as reference) is mgR+710mv20.DAll options are correct.
A uniform solid sphere rolls on a horizontal surface at 20ms−1 . It then rolls up in incline having an angle of inclination at 30∘ with the horizontal . If the friction losses are negligible , the value of height h above the ground where the ball stops is
View SolutionA sphere of mass 2 kg and radius 0.5 m is rolling with an initial speed of 1 ms−1 goes up an inclined plane which makes an angle of 30∘ with the horizontal plane, without slipping, How long will the sphere take to return to the starting point A ?

View SolutionA one kg rolling on a smooth horizontal surface at 20ms−1 comes to the bottom of an inclined plane making an angle of 30∘ with the horizontal. Calculate K.E. of the ball when it is at the bottom of incline. How far up the incline will the ball roll ? Neglect friction.
View SolutionA body of mass M and radius r, rolling on a smooth horizontal floor with velocity v, rolls up an irregular inclined plane up to a vertical height (3v2/4g). Compute the moment of inertia of the body.
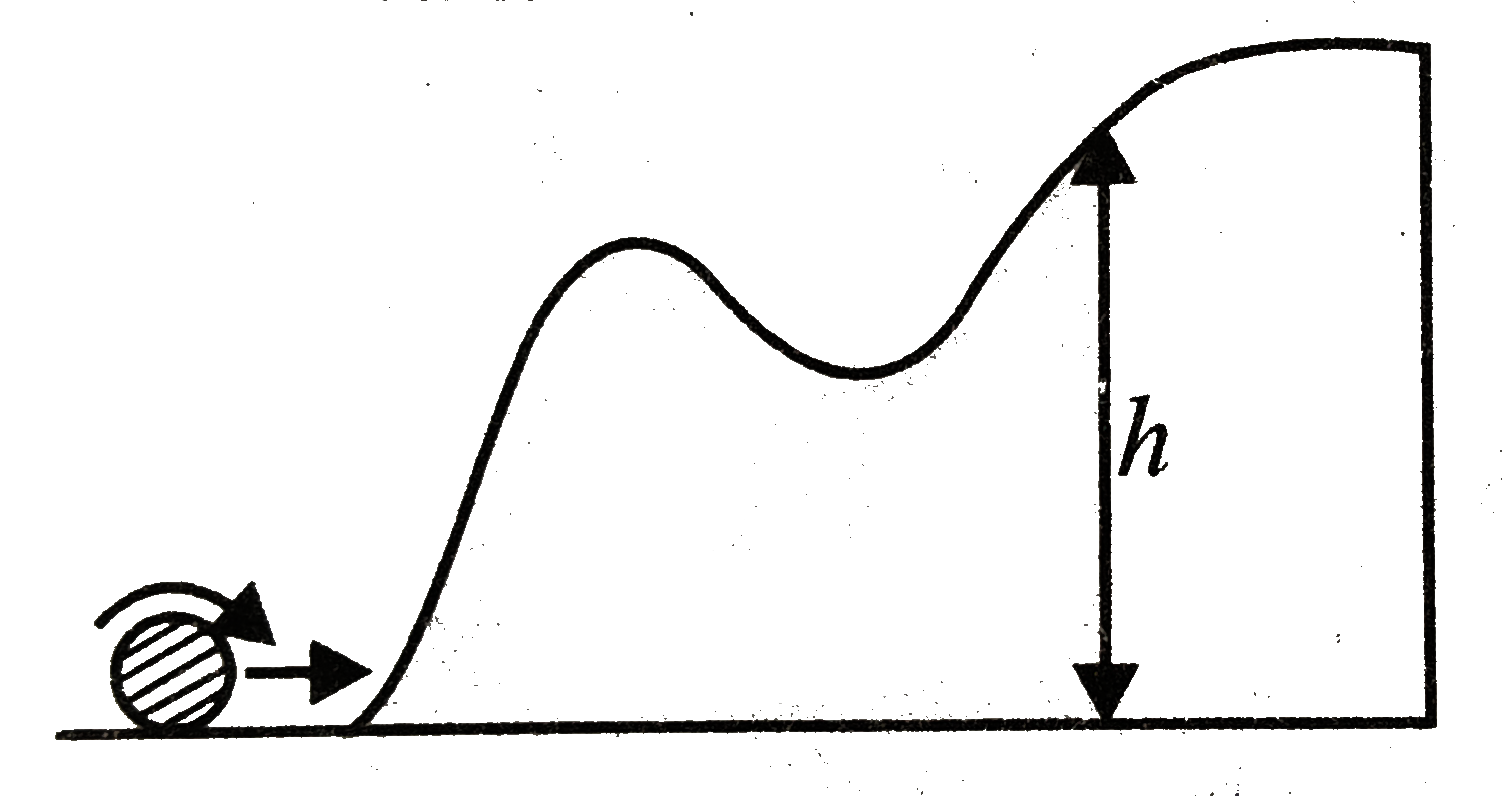
View SolutionA body of mass M and radius r, rolling on a smooth horizontal floor with velocity v, rolls up an irregular inclined plane up to a vertical height (3v2/4g). Compute the moment of inertia of the body.

View SolutionA solid sphere having mass m and radius r rolls down an inclined plane. Then its kinetic energy is
View Solution
