Moment of inertia of a uniform rod of mass m and length l is 712ml2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.
l2
l√2
l4
l√3
The correct Answer is:B
I=Icm+md2
7ml12=ml212+md2⇒d2=l22
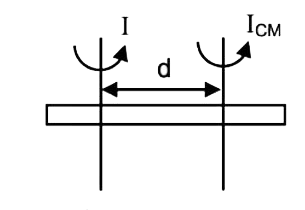
d=l√2
Topper's Solved these Questions
Similar Questions
the moment of inertia of a uniform rod of mass 0.50 kg and length 1 m is 0.10 kg m^2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.
View SolutionThe moment of inertia of a uniform rod of mass m = 0.50 kg and length I=1m is I=0.10kg−m2 about a line perpendicular to the rod. The distance of this line from the middle point of the rod is √abm rod where a and b are coprime. Find a + b
View Solution
Knowledge Check
Moment of inertia of a uniform rod of mass m and length l is 712ml2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.
Al2Bl√2Cl4Dl√3The moment of inertia of a thin rod of mass M and length L about an axis perpendicular to the rod at a distance L/4 from one end is
AML26BML212C7ML224D7ML248The moment of inertia of a thin uniform rod of mass M and length L about an axis perpendicular to the rod, through its centre is I. The moment of inertia of the rod about an axis perpendicular to rod through its end point is
AI4BI2C2ID4I
The moment of inertia of a thin rod of mass M and length L about an axis perpendicular to the rod at a distance L/4 from one end is
View SolutionThe radius of gyration of a uniform rod of mass m and length l about an axis perpendicular to its length and at distance l4 from its one end will be
View SolutionCalculate the moment of inertia of a rod of mass M, and length l about an axis perpendicular to it passing through one of its ends.
View SolutionThe moment of inertia of a thin uniform rod of mass M and length l about an axis perpendicular to the rod through its centre is I. The moment of inertia of the rod through its end point is
View SolutionMoment of inertia of a thin rod of mass 'M' and length 'L' about the axis perpendicular to the rod and passing through its centre is ............
View Solution
