The electric field lines coming out of an equipotential surface are
perpendicular to the surface
parallel to the surface
in all directions
zero
The correct Answer is:A
To solve the question regarding the electric field lines coming out of an equipotential surface, we can follow these steps:
Step 1: Understand the concept of an equipotential surface
An equipotential surface is defined as a surface on which the electric potential is the same at every point. This means that no work is done when moving a charge along this surface.
Hint: Recall that an equipotential surface has constant potential, so the potential difference between any two points on the surface is zero.
Step 2: Analyze the relationship between electric field and equipotential surfaces
The electric field (E) is related to the potential (V) by the equation:
E=−∇V
This implies that the electric field points in the direction of the greatest decrease of potential.
Hint: Remember that the electric field is always directed from regions of higher potential to lower potential.
Step 3: Determine the orientation of electric field lines
Since the electric field lines represent the direction of the electric field, they must be perpendicular to the equipotential surface. If they were not perpendicular, it would imply that there is a potential difference along the surface, which contradicts the definition of an equipotential surface.
Hint: Visualize the electric field lines as arrows that must intersect the equipotential surface at right angles.
Step 4: Conclude the relationship
Based on the above analysis, we can conclude that the electric field lines coming out of an equipotential surface are always perpendicular to that surface.
Final Answer: The electric field lines coming out of an equipotential surface are perpendicular to the surface.
An equipotential surface is defined as a surface on which the electric potential is the same at every point. This means that no work is done when moving a charge along this surface.
The electric field (E) is related to the potential (V) by the equation:
E=−∇V
This implies that the electric field points in the direction of the greatest decrease of potential.
Since the electric field lines represent the direction of the electric field, they must be perpendicular to the equipotential surface. If they were not perpendicular, it would imply that there is a potential difference along the surface, which contradicts the definition of an equipotential surface.
Based on the above analysis, we can conclude that the electric field lines coming out of an equipotential surface are always perpendicular to that surface.
Topper's Solved these Questions
- NTA TPC JEE MAIN TEST 104BOOK - NTA MOCK TESTSCHAPTER - NTA TPC JEE MAIN TEST 104EXERCISE - PHYSICS (SUBJECTIVE NUMERICAL)
10 Videos
- NTA TPC JEE MAIN TEST 103BOOK - NTA MOCK TESTSCHAPTER - NTA TPC JEE MAIN TEST 103EXERCISE - PHYSICS
30 Videos
- NTA TPC JEE MAIN TEST 105BOOK - NTA MOCK TESTSCHAPTER - NTA TPC JEE MAIN TEST 105EXERCISE - PHYSICS
30 Videos
Similar Questions
Can electric field exist longitudinal to an equipotential surface ? Give reason .
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Draw equipotential surfaces around an isolated positive point charge + q. Mark direction of electric field →E also.
View Solution
Knowledge Check
The electric flux coming out of the equi-potential surface is -
Aperpendicular to the surfaceBparallel to the surfaceCin all directionsDzero
On the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Four equipotential surfaces are shown in the adjoining figure. Find the magnitude and direaction of the electric field.
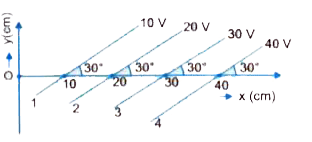
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
If a charge of 2 nC is taken from equipotential surface number 2 to 3, what is the amount of work done ?
View SolutionDefine an equipotential surface. Draw equipotential surfaces :
(i) in the case of single point charge and
(ii) in a constant electric field in Z-direction.
Why the equipotential surfaces about a single charge are not equidistant ?
(iii) Can electric field exist tangential to an equipotential surface ? Given reason.
View SolutionDefine an equipotential surface. Draw equipotential surfaces :
(i) in the case of single point charge and
(ii) in a constant electric field in Z-direction.
Why the equpotential surfaces about a single charge are not equidistant ?
(iii) Can electric field exist tangential to an equipotential surface ? Given reason.
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
If electric potential at origin point O be 100 V, then for electric field specified in part (b), draw equipotential surfaces corresponding to potentials 80 V, 60 V and 40 V respectively.
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
A uniform electric field →E=1000ˆiVm−1 exists in space at a given place. What is the shape of equipotential surfaces there ?
View SolutionThe angle between the electric lines of force and an equipotential surface is
View Solution
