In a right angled , right-angled at A, what is the value of secB if BC is 5.2 cm and AB is 3 cm
1.6
1.07
1.5
1.73
To find the value of sec B in the given right-angled triangle, we can follow these steps:
Step 1: Understand the triangle
We have a right-angled triangle ABC with the right angle at A. The sides are given as follows:
- BC (hypotenuse) = 5.2 cm
- AB (adjacent side to angle B) = 3 cm
Step 2: Identify the relationship for sec B
The secant of angle B (sec B) is defined as the reciprocal of the cosine of angle B:
secB=1cosB
And cosine is defined as the ratio of the length of the adjacent side to the length of the hypotenuse:
cosB=AdjacentHypotenuse=ABBC
Step 3: Substitute the values
Now we can substitute the values we have:
cosB=ABBC=3 cm5.2 cm
Step 4: Calculate cos B
cosB=35.2
To simplify this, we can divide both the numerator and the denominator by 1:
cosB=35.2≈0.5769
Step 5: Calculate sec B
Now, we can find sec B:
secB=1cosB=10.5769≈1.733
Final Answer
Thus, the value of sec B is approximately:
secB≈1.733
We have a right-angled triangle ABC with the right angle at A. The sides are given as follows:
- BC (hypotenuse) = 5.2 cm
- AB (adjacent side to angle B) = 3 cm
The secant of angle B (sec B) is defined as the reciprocal of the cosine of angle B:
secB=1cosB
And cosine is defined as the ratio of the length of the adjacent side to the length of the hypotenuse:
cosB=AdjacentHypotenuse=ABBC
Now we can substitute the values we have:
cosB=ABBC=3 cm5.2 cm
cosB=35.2
To simplify this, we can divide both the numerator and the denominator by 1:
cosB=35.2≈0.5769
Now, we can find sec B:
secB=1cosB=10.5769≈1.733
Thus, the value of sec B is approximately:
secB≈1.733
Similar Questions
Knowledge Check
- Question 1 - Select One
ABC is a right-angled triangle, right angled at A and AD is the altitude on BC. If AB : AC= 3:4, what is the ratio BD:DC?
A3:4B9:16C2:3D1:2 - Question 2 - Select One
In ΔABC, right angled at B, AB = 24 cm, BC = 7 cm. The value of sinC is :
A2425B725C724DNone of these - Question 3 - Select One
△ABC is a right angled at A and AD is the altitude to BC. If AB= 7 cm and AC = 24 cm. Find the ratio of AD is to AM if M is the mid-point of BC.
A25:41B32:41C336625D625336
A right triangle ABC, right angled at A, is circumscribing a circle. If AB = 8 cm and BC = 6 cm, find the radius of the circle.
View SolutionIn the given figure, ABC is a right angled triangle, right -angled at A in which AB=6cm,BC=10cm and I is the incentre of ΔABC.
Find the area of the shaded region. [Take π=3.14]
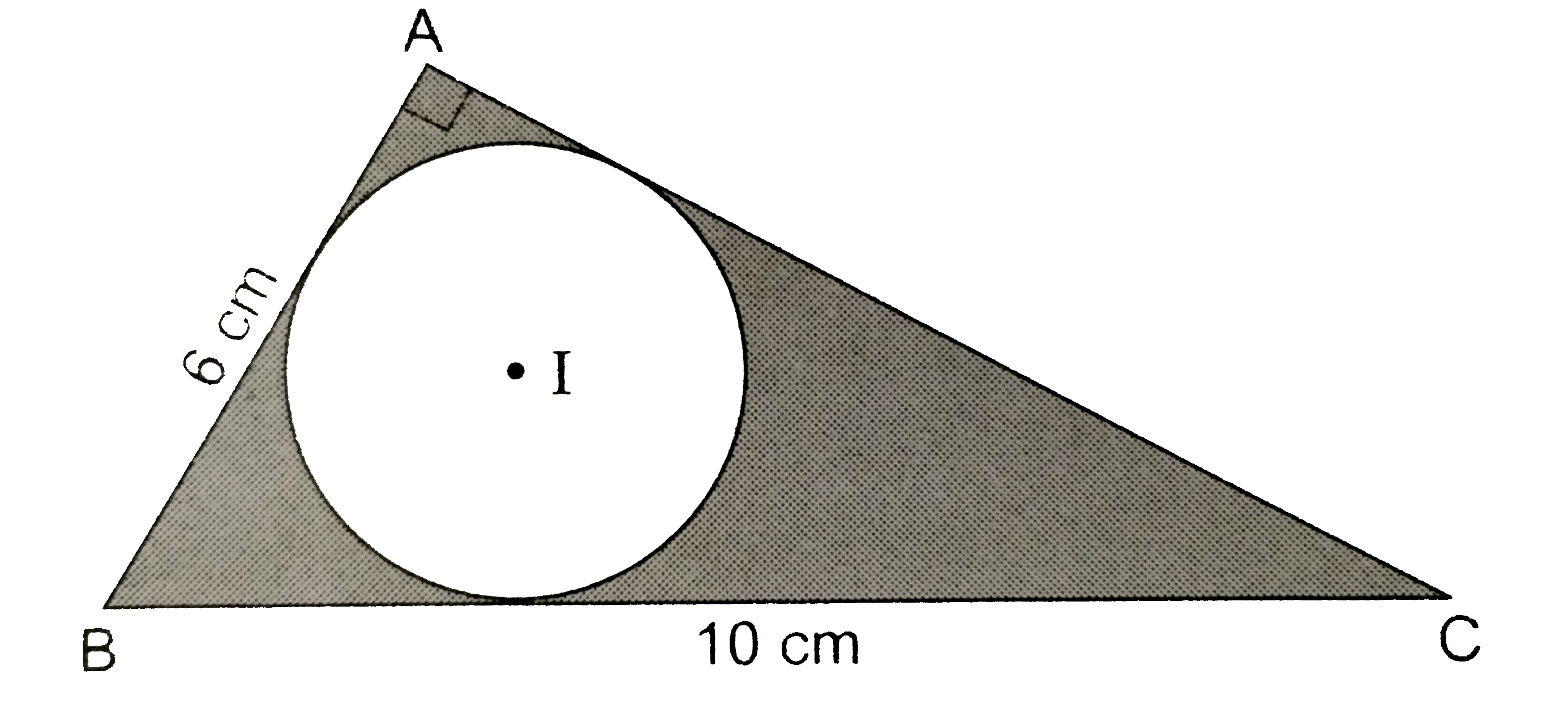 View Solution
View SolutionIn a right angled triangle, with angle at A being 90∘, the side AB is of length 3 cm and BC is 12 cm. What is the length of side AC?
View SolutionIn a right angled triangle, with angle of A being right angle, the side AB is of length 4 cm and BC is 15 cm. What is the length of side AC?
View SolutionIn ΔABC, right angled at B, AB=24cm,BC=7cm. The value of cosC is :
View Solution
